Economic Dispatch for Power Systems of Multiple Wind Farms Based on MVTV Copula Method
-
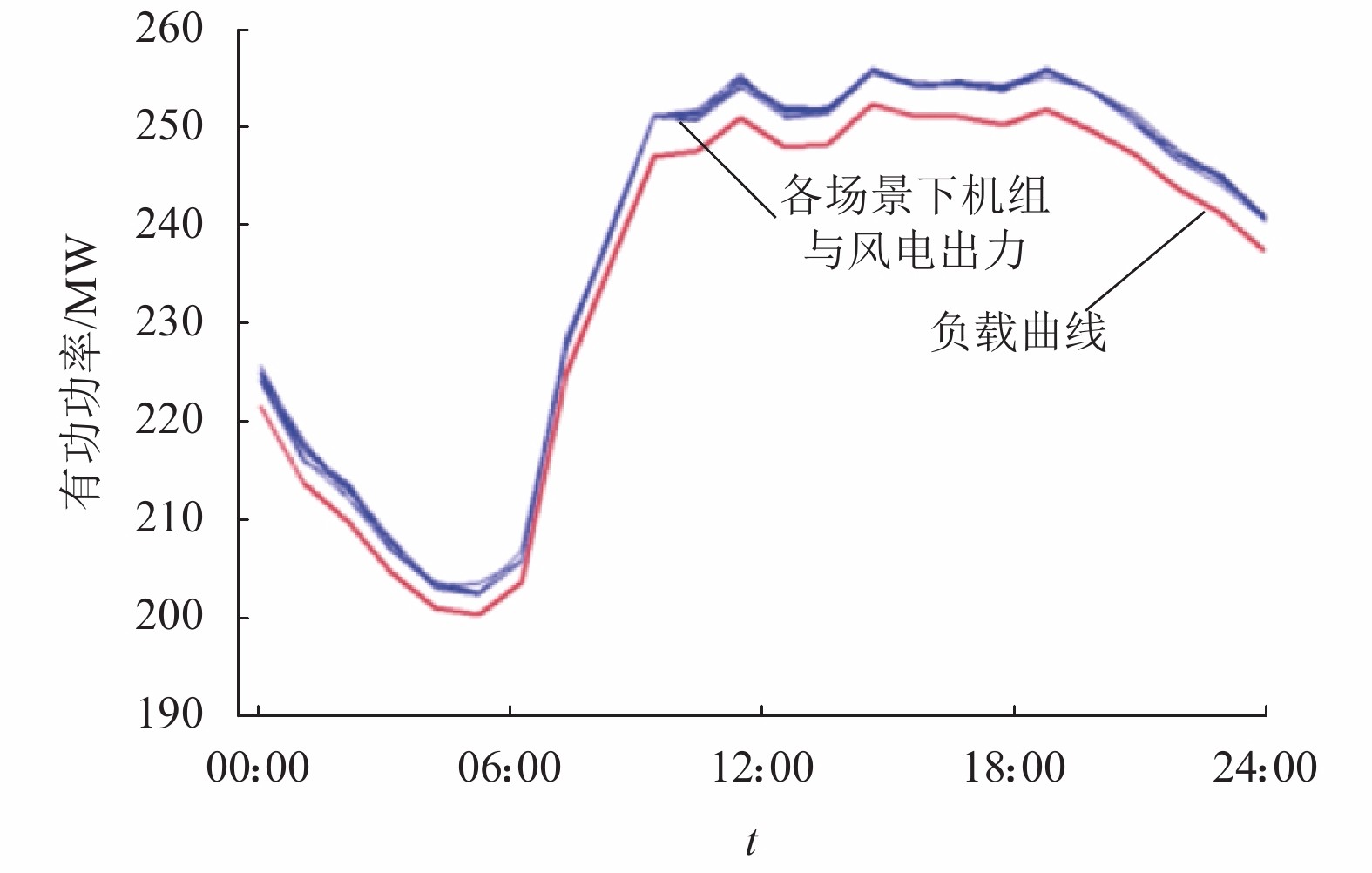
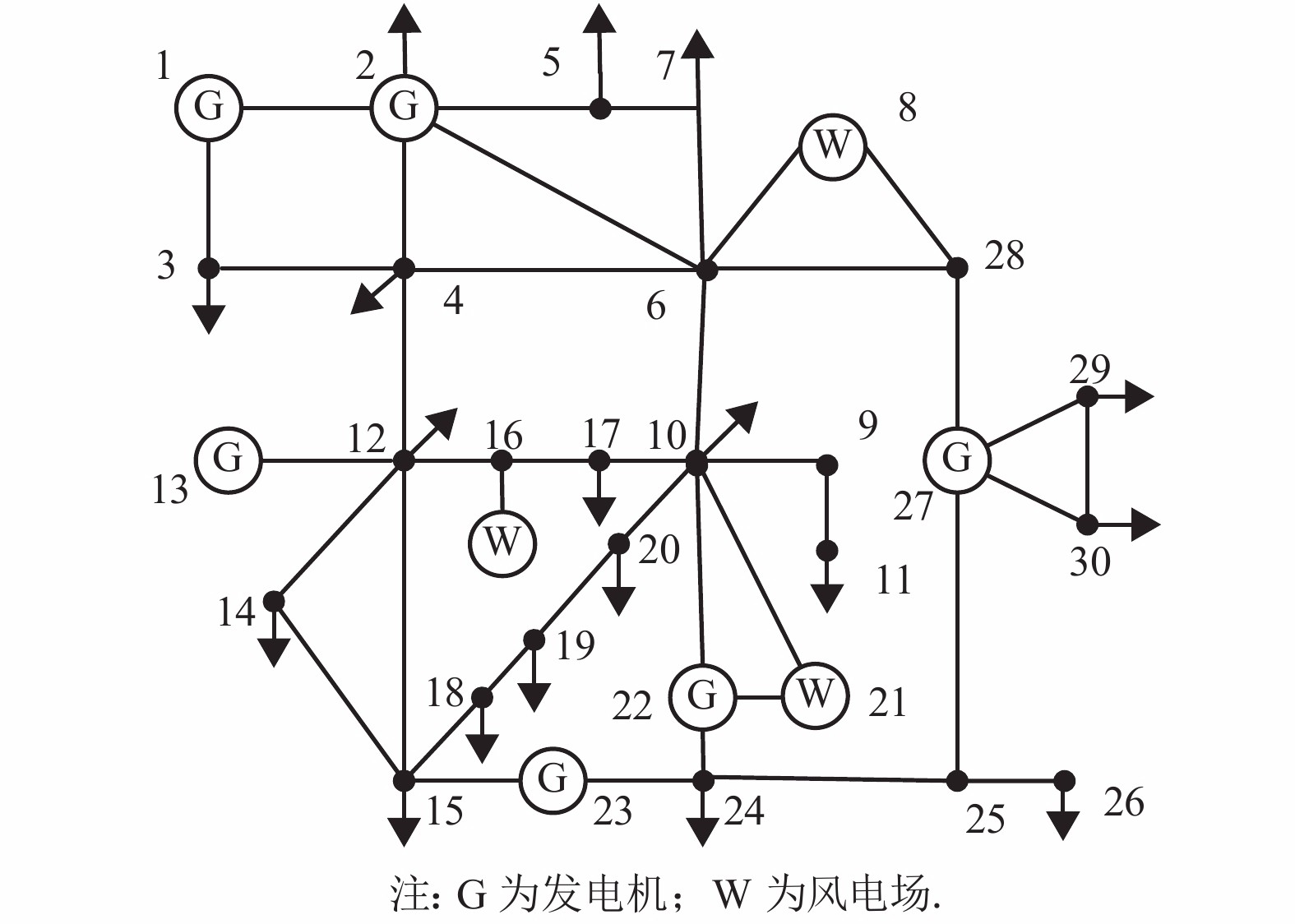
摘要: 针对多维风电出力数据间相关性对含有多风电场的电力系统经济调度影响问题，提出了MVTV Copula （mix vine time-varying Copula）方法，以此构建多风电场出力数据随机场景，并建立基于机组燃料费用及再调度费用最小为目标的电力系统经济调度模型. 为验证所述方法的有效性，采用IEEE-30节点系统，并以我国某地3个相邻风电场的实际出力为例对所述方法进行了仿真验证. 仿真结果表明：不考虑风电出力相关性的经济调度费用仅为实际调度费用的43.6%，而应用所述MTVT Copula方法建模后的再调度费用则更加接近实际调度费用，为实际调度费用的82%，验证了本文所提方法的有效性.
-
关键词:
- 风电出力 /
- 相关性 /
- MTVT Copula方法 /
- 经济调度
Abstract: As the correlation of multiple wind power outputs is of great significance for the economic dispatch analysis of wind farm power systems, the mix vine time-varying Copula (MVTV Copula) is proposed to construct the random scenarios of multiple wind power outputs.The economic dispatch model is built with the objective of minimizing unit fuel cost and dispatch cost. An IEEE-30 node system is referenced and the measured outputs of three neighboring wind farms in China are used to validate the proposed method. The simulation results show that the cost of the economic dispatch that ignores the correlation of wind power output is only 43.6% of the actual scheduling cost, while that of the model based on the MTVT Copula method is much closer to the actual scheduling cost, being 82% of the actual cost. It is proved that theoutput model of multiple wind farms is more consistent with the actual situation, which can well describe the relevant characteristics of multiple windfarm outputs, and the economic dispatch based on this method can yield ideal dispatch results.-
Key words:
- wind power output /
- correlation /
- MVTV Copula method /
- economic dispatch
-
图 4 基于MVTV Copula模型的典型随机场景
Figure 4. Typical random scenarios based on MVTV Copula model
图 5 不考虑相关性的典型随机场景
Figure 5. Typical random scenarios ignoring dependence of wind outputs
图 6 负载曲线及各场景下机组和风电总出力曲线
Figure 6. Load curve and total output curve of unit and wind power output under different scenarios
表 1 各场景下最优藤结构及最优参数
Table 1. Optimal vine structure and optimal parameters for different scenarios
场景 藤结构 最优 Copula 函数 AIC 值 1 C {tv-gumbel，tv-SJC，tv-gumbel} 0.384 2 D {tv-gumbel，tv-normal，tv-gumbel} 0.195 3 C { tv-SJC，tv-gumbel，tv-gumbel} 0.119 4 D {tv-SJC，tv-SJC，tv-gumbel} 0.097 5 D {tv-normal，tv-SJC，tv-gumbel} 0.062 注：tv-SJC、tv-gumbel、tv-normal 分别为时变SJC Copula函数、时变gumbel copula函数、时变normal函数. 
下载: 导出CSV
表 2 采用混合藤与孤立藤结构的AIC值对比
Table 2. AIC values of mix vine and isolated vine structures
藤结构 C 藤 D 藤 混合藤 AIC 值 0.197 0.217 0.129
下载: 导出CSV
表 3 两类典型随机场景情况调度费用对比
Table 3. Economic dispatch results of two typical random scenarios
USD 场景 预测场景
煤耗费用再调度费用 总费用 不考虑相关性 9 969.16 1 544.45 11 513.61 考虑相关性 9 969.16 3 540.64 13 509.80 实际出力 9 969.16 4 320.77 14 289.93
下载: 导出CSV
-
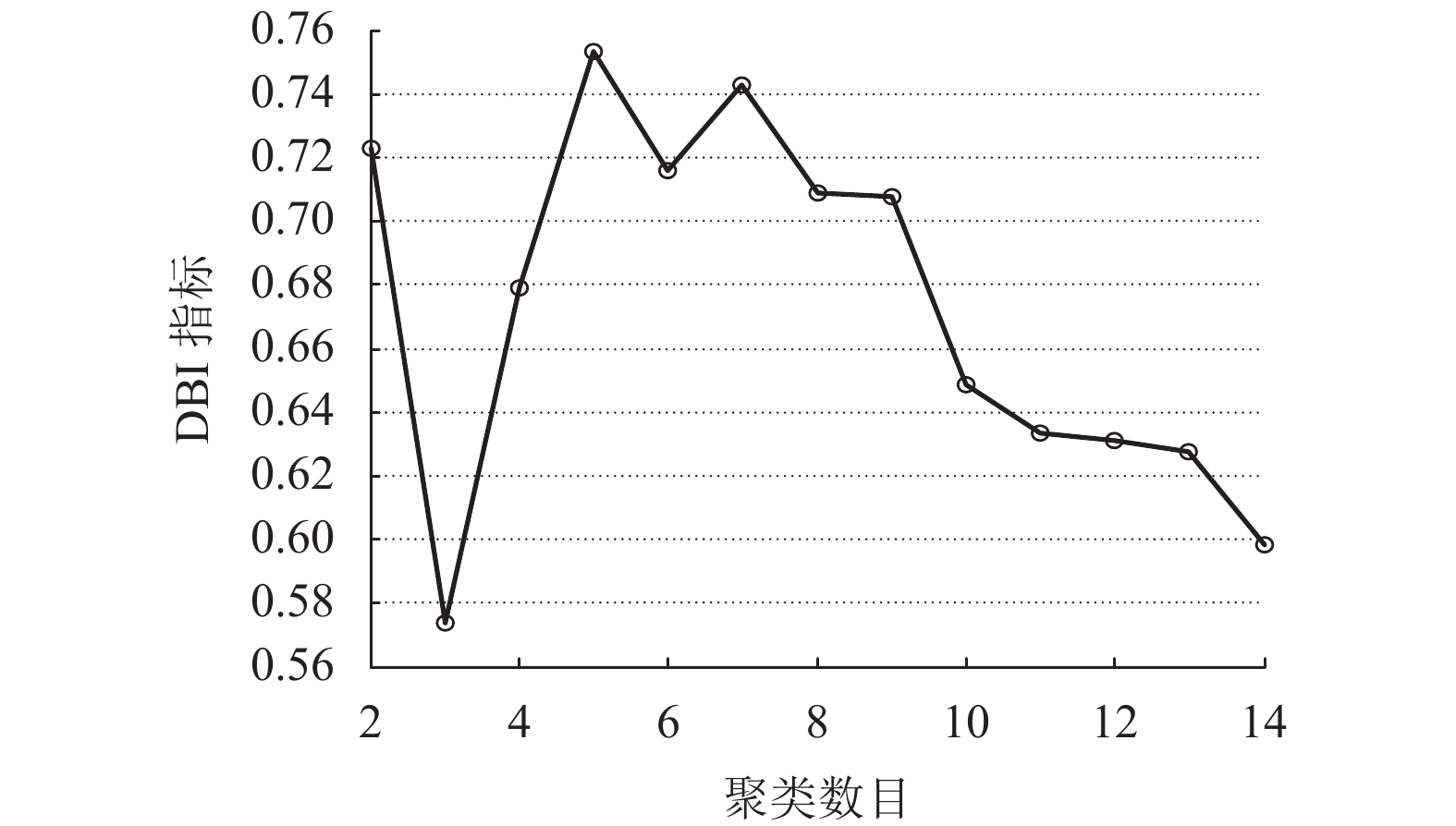
卢锦玲,於慧敏. 基于混合Copula的风光功率相关结构分析[J]. 太阳能学报,2017,38(11): 3188-3194.LU Jinling, YU Huimin. Dependence structure analysis of wind and PV power based on hybrid copula[J]. Acta Energiae Sloaris Sinica, 2017, 38(11): 3188-3194. LIU H, HU Z, SONG Y, et al. Decentralized vehicle-to-Grid control for primary frequency regulation considering charging demands[J]. IEEE Transactions on Power Systems, 2013, 28(3): 3480-3489. doi: 10.1109/TPWRS.2013.2252029 薛禹胜,雷兴,薛峰,等. 关于风电不确定性对电力系统影响的评述[J]. 中国电机工程学报,2014,34(29): 5029-5040.XUE Yusheng, LEI Xing, XUE Feng, et al. A review on impacts of wind power uncertainties on power systems[J]. Proceedings of the CSEE, 2014, 34(29): 5029-5040. 张宗益,亢娅丽,郭兴磊. 基于时变Copula的供电公司多期购电组合优化模型[J]. 管理工程学报,2013,27(1): 147-152. doi: 10.3969/j.issn.1004-6062.2013.01.021ZHANG Zongyi, KANG Yali, GUO Xinglei. A multi-period electricity purchasing model for power supply company[J]. Journal of Industrial Engineering and Engineering Management, 2013, 27(1): 147-152. doi: 10.3969/j.issn.1004-6062.2013.01.021 潘雄,王莉莉,徐玉琴,等. 基于混合Copula函数的风电场出力建模方法[J]. 电力系统自动化,2014,38(14): 17-22. doi: 10.7500/AEPS20130506008PAN Xiong, WANG Lili, XU Yuqin, et al. A wind farm power modeling method based on mixed Copula[J]. Automation of Electric Power System, 2014, 38(14): 17-22. doi: 10.7500/AEPS20130506008 王小红,周步祥,张乐,等. 基于时变Copula函数的风电出力相关性分析[J]. 电力系统及其自动化学报,2015,27(1): 43-48. doi: 10.3969/j.issn.1003-8930.2015.01.008WANG Xiaohong, ZHOU Buxiang, ZHANG Le, et al. Wind power correlation analysis based on time-variant Copula function[J]. Automation of Electric Power System, 2015, 27(1): 43-48. doi: 10.3969/j.issn.1003-8930.2015.01.008 卢锦玲,於慧敏,杨进. 计及风电输出相依结构和柔性负荷激励/补偿机制的随机调度策略[J]. 电网技术,2017,41(6): 1793-1800.LU Jinling, YU Huimin, YANG Jin. Stochastic scheduling strategy considering wind power dependence structure and motivation-compensation mechanism of flexible load[J]. Power System Technology, 2017, 41(6): 1793-1800. PATTON A. Modeling time-varying exchange rate dependence using the conditional copula[R]. San Diego: University of Carlifornia, 2001. 吴巍,汪可友,李国杰,等. 基于Pair Copula的多维风电功率相关性分析及建模[J]. 电力系统自动化,2015,39(16): 37-42. doi: 10.7500/AEPS20141031011WU Wei, WANG Keyou, LI Guojie, et al. Correlation analysis and modeling of multiple wind power based on Pair Copula[J]. Automation of Electric Power System, 2015, 39(16): 37-42. doi: 10.7500/AEPS20141031011 CIVICIOGLU P. Backtracking search optimization algorithm for numerical optimization problems[J]. Applied Mathematics and Computation. 2013, 219(15): 8121-8144. SKLAR M. Fonctions de répartition á n dimensions et leurs marges[J]. Publ. Inst. Statist. Univ. Paris, 1959, 8: 229-231. AAS K, CZADO C, FRIGESSI A, et al. Paircopula constructions of multiple dependence[J]. Insurance:Mathematics and Economics, 2009, 44(2): 182-198. 张充,檀勤良,汤石雨,等. 基于风速变化周期的风电场功率分类组合预测模型[J]. 太阳能学报,2017,38(4): 999-1006.ZHANG Chong, TAN Qinliang, TANG Shiyu, et al. Classification and combination predicition model for wind power based on change period of wind speed[J]. Acta Energiae Sloaris Sinica, 2017, 38(4): 999-1006. 邱宜彬,孟翔,欧阳誉波,等. 基于混合藤Copula模型的多维风电出力相关性建模[J]. 太阳能学报,2017(9): 2512-2519.QIU Yibin, MENG Xiang, OUYANG Yubo, et al. Denpendence modeling of multidimensional wind farm output based on mixture vine Copula structure[J]. Acta Energiae Solaris Sineca, 2017(9): 2512-2519. 刘燕驰. 基于密度的最佳聚类数确定方法[J]. 中国理信息化,2011(9): 30-33.LIU Yanchi. Method for determing optimal number of clusters based on density[J]. China Management Imformation, 2011(9): 30-33. 张国富,杜子平,张俊. 基于混合C藤Copula的我国一篮子货币汇率相依结构研究[J]. 数学的实践与认识,2014,44(11): 74-84.ZHANG Guofu, DU Ziping, ZHANG Jun. Study on depedence structure of RMB exchange rates with basket of currencies based on mixed C-vines[J]. Mathematics in Practice and Theory, 2014, 44(11): 74-84. GRZEGORZEWSKI P, SZYMANOWSKI H. Goodness-of-fit tests for fuzzy data[J]. Information Sciences, 2014, 288: 374-386. 王永杰,吴文传,张伯明. 考虑负荷量测和光伏不确定性的主动配电网鲁棒电压控制[J]. 电力系统自动化,2015,39(9): 138-144.WANG Yongjie, WU Wenchuan, ZHANG Boming. Rubust voltage control model for active distribution network considering load and photovoltaic uncertainties[J]. Automation of Electric Power System, 2015, 39(9): 138-144. 孙元章,吴俊,李国杰. 风力发电对电力系统的影响[J]. 电网技术,2007,31(20): 55-62.SUN Yuanzhang, WU Jun, LI Guojie. Influence research of wind power generation on power system[J]. Power System Technology, 2007, 31(20): 55-62. 田蓓,于珍,李旭涛,等. 考虑多风电场功率相关性的概率潮流联合分布计算[J]. 中国电力,2017,50(10): 71-77.TIAN Bei, YU Zhen, LI Xutao et al. Joint distribution calculation with pribabilistic powe flow cosidering dependencies among multiple wind power generations[J]. Eletric Power, 2017, 50(10): 71-77. 杨柳青,林舜江,刘明波,等. 考虑风电接入的大型电力系统多目标动态优化调度[J]. 电工技术学报,2014,29(10): 286-295. doi: 10.3969/j.issn.1000-6753.2014.10.035YANG Liuqing, LIN Shunjiang, LIU Mingbo, et al. Multi-objective dynamic optimal dispatch for large-scale power systems considering wind power penetration[J]. Transactions of China Electrotechnical Society, 2014, 29(10): 286-295. doi: 10.3969/j.issn.1000-6753.2014.10.035 黎静华,文劲宇,程时杰,等. 考虑多风电场出力Copula相关关系的场景生成方法[J]. 中国电机工程学报,2013,33(16): 30-36.LI Jinghua, WEN Jingyu, CHENG Shijie, et al. A scene generation method considering Copula correlation relationship of multi-wind farms power[J]. Proceedings of the CSEE, 2013, 33(16): 30-36. 向红吉,戴朝华,明杰,等. 考虑低谷时刻负调峰能力及风电预测区间的多目标机组组合优化研究[J]. 电网技术,2017,41(6): 1912-1918.XIANG Hongjie, DAI Chaohua, MING Jie, et al. Research on multi-objective optimization of unit commitment considering negative peak load regulation ability in valley load period and wind power prediction interval[J]. Power System Technology, 2017, 41(6): 1912-1918. -
下载:
点击查看大图
图(6) / 表(3)
计量
- 文章访问数: 438
- HTML全文浏览量: 237
- PDF下载量: 12
- 被引次数: 0