Extreme Value Distribution Estimation Method for Nonlinear Seismic Response of Bridge Structures
-
摘要: 为了研究近断层脉冲地震作用下桥梁非线性地震响应极值分布,进行小失效概率下的桥梁动力可靠度精确计算,提出了一种有效的近断层脉冲地震作用下桥梁结构非线性地震响应极值分布分析方法. 首先考虑桥梁结构的非线性和地震动的不确定性,采用拉丁超立方抽样对近断层脉冲地震动随机参数和结构随机参数进行随机抽样,通过模拟的高频地震动均方值和与精确值的相对误差确定出所需要的样本数量;其次以合成的近断层脉冲地震动作为地震激励,通过时程分析对结构非线性动力方程进行求解,从而得到结构非线性地震响应极值样本,再采用改进的分数阶矩最大熵原理获得结构非线性地震响应的极值分布;最后通过非线性单自由度系统和三层非线性剪切框架验证了该方法的有效性. 研究结果表明:该方法不仅能够有效的模拟近断层脉冲地震作用时,桥梁结构与地震动双重不确定性影响下的动力响应极值分布,更能在兼顾效率和计算精度时,精确估计桥梁结构非线性地震响应极值的尾部分布,能够为桥梁结构非线性动力可靠度评估提供一种有效的途径.Abstract: To study the extreme value distribution (EVD) of nonlinear seismic response of bridges subjected to near-fault impulse ground motion and accurately estimates the dynamic reliability of bridges at small failure probability level, an effective method is proposed and validated. Considering the nonlinearity of bridge structures and the uncertainty of ground motions, the random parameters of near-fault impulse ground motion and structure are sampled by Latin hypercube sampling (LHS). The required number of seismic samples is determined by the relative error between the mean square value of simulated high-frequency ground motion and the exact value. Using synthetic near-fault impulse ground motions as seismic excitation, the structural nonlinear dynamic equation is solved by time history analysis, and the structural nonlinear seismic response extreme value samples are obtained. Then, the improved maximum entropy principle with fraction moment is adopted to obtain the EVD of bridges. The accuracy and efficiency of the proposed method are verified by a single degree freedom system and a three-story shear frame structure in comparison with the Monte Carlo simulation. Results indicate that the proposed method can not only effectively simulate the EVD of dynamic response of bridge under the double uncertainties influence of structure and ground motion, but also accurately estimate the tail distribution of the extreme value of nonlinear seismic response of bridge structure, with a balanced between efficiency and calculation accuracy. This method provides an effective way for evaluating the non-linear dynamic reliability of bridge structures.
-
边坡中的软弱夹层虽然厚度较薄,但由于其强度较低,因而备受研究者重视,特别是当作用地震等动荷载时. 刘汉香等[1]通过振动台模型试验分析了含软弱夹层斜坡的动力响应特征;闫孔明等[2]发现随着地震荷载强度的增大,软弱夹层中响应加速度的低频成分逐渐增多;唐云波等[3]通过分析测试数据发现软弱层的强度越低,边坡的动力响应越显著;刘晋南等[4]认为水平地震作用下,斜坡路基的动力稳定性并非随着软弱夹层厚度的增大而减小,软弱夹层厚度存在最优值.
为了指导地震工况下边坡的设计,研究者通常采用极限平衡法、极限分析法、数值模拟等计算方法. 尽管数值仿真可较好地模拟结构响应特性,但其往往需要一定编程基础,同时,模型本构关系的模拟也较为复杂. 目前设计人员多采用极限平衡法进行边坡设计,例如瑞典圆弧法、毕肖普法、简布法、摩根斯坦-普顿斯法等. 尽管极限平衡法满足力或者力矩平衡、摩尔-库伦准则等条件,但其未考虑土体自身的应力-应变关系[5]. 相较于上述极限平衡法,极限分析法通过刚塑性假定可反映土体应力-应变关系,同时由于其计算更方便、简单[6],因此被广泛应用于边坡稳定性计算分析. Michalowski等[7]分析了转动模式下边坡极限高度与安全系数;何思明等[8]根据上限定理研究了设计参数对破裂面形状及稳定系数的影响;Utili[9]讨论了裂缝位置与深度对边坡稳定性的影响. 与极限平衡法相比,极限分析法解答更严密,同时由于其计算中仅需要求解一个能量方程,因而计算更简便[10],然而,目前鲜有关于地震荷载下含软弱夹层边坡滑动极限分析的研究报道.
为此本文采用极限分析法中的上限定理,对含软弱夹层边坡稳定性进行了求解,并将计算结果与既有方法进行了对比,最后分析了荷载、软弱夹层形状、强度等对边坡稳定性的影响.
1. 计算模型
1.1 基本假定
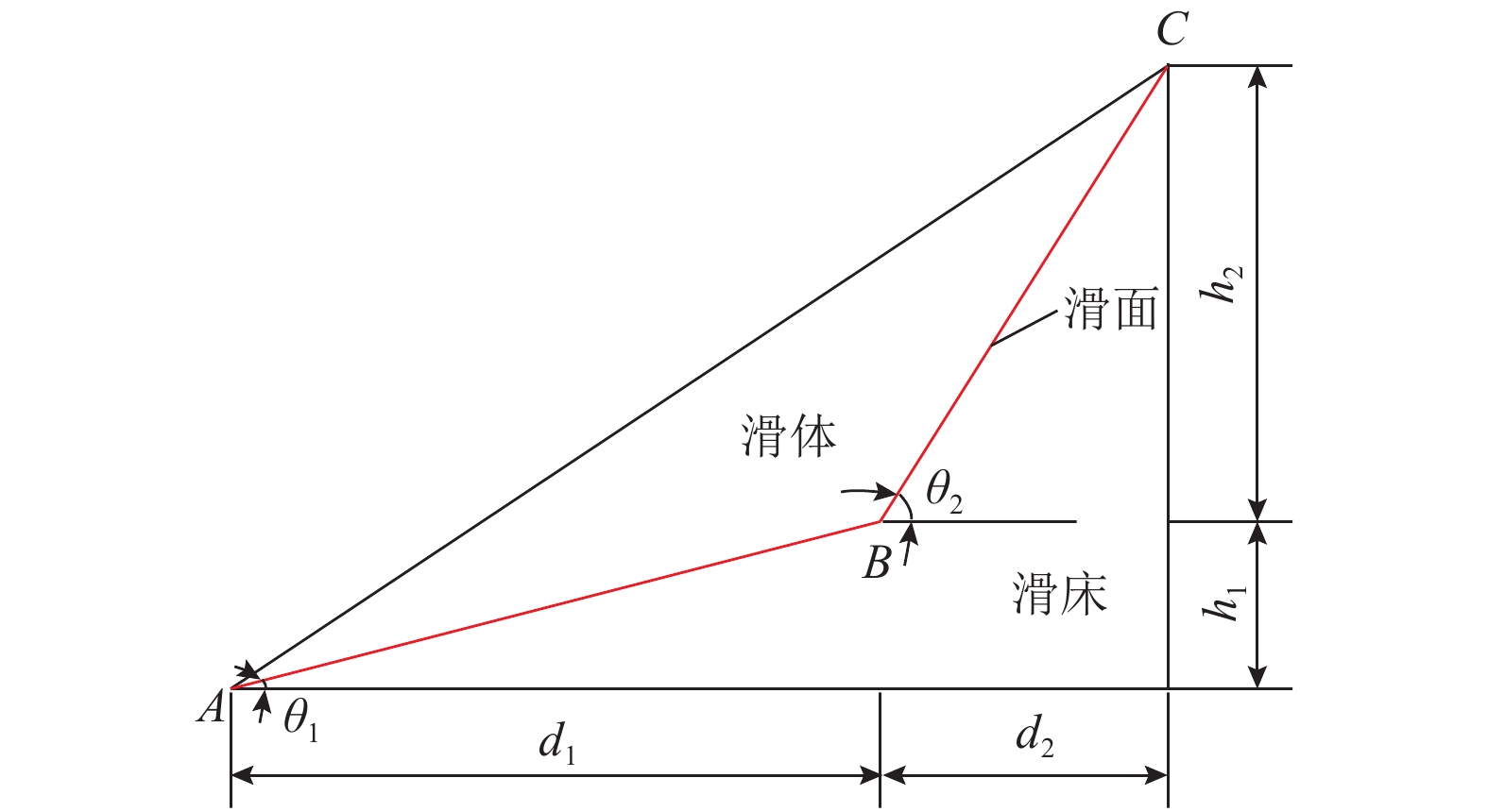
边坡模型如图1所示,其中,折线ABC表示薄软弱夹层(抗剪强度较弱的层带结构),△ABC表示滑体. 为了实现拟动力法与上限定理的应用,对图1模型作以下简化与假定:
1) 模型为二维平面模型,满足平面应变条件.
2) 软弱薄夹层AB、BC与水平面的夹角分别为θ1、θ2 ,夹层AB、BC的水平长度与竖直高度分别为d1与h1,d2与h2.
3) 地震动荷载作用在土块质心.
根据Choudhury等[11-13]研究,为了考虑动荷载在介质中传播特性、放大效应、时程特征等,水平加速度系数为
kh(z,t)=kp[1+H−zz(fa−1)]×sin[ω(t−H−zvs)], (1) 式中:kh(z,t)为高度z、 时间t时的水平加速度系数;kp为水平加速度峰值系数;H(H=h1 + h2)为滑坡高度;fa为加速度放大系数;ω、vs分别为角速度、剪切波速,如式(2)、(3).
ω=2πf, (2) vs=√Gρ, (3) 式中:f为频率;G为土体剪切模量;ρ为土体密度.
4) 填土满足极限分析原理中的刚塑性假定条件、摩尔库伦屈服条件,同时遵循关联流动法则.
5) 根据强度折减系数的概念,边坡安全系数F采用式(4)表示.
F=ccm=tanφtanφm, (4) 式中:c、φ为软弱夹层黏聚力与摩擦角;cm、φm为折减后对应的强度参数.
1.2 极限分析
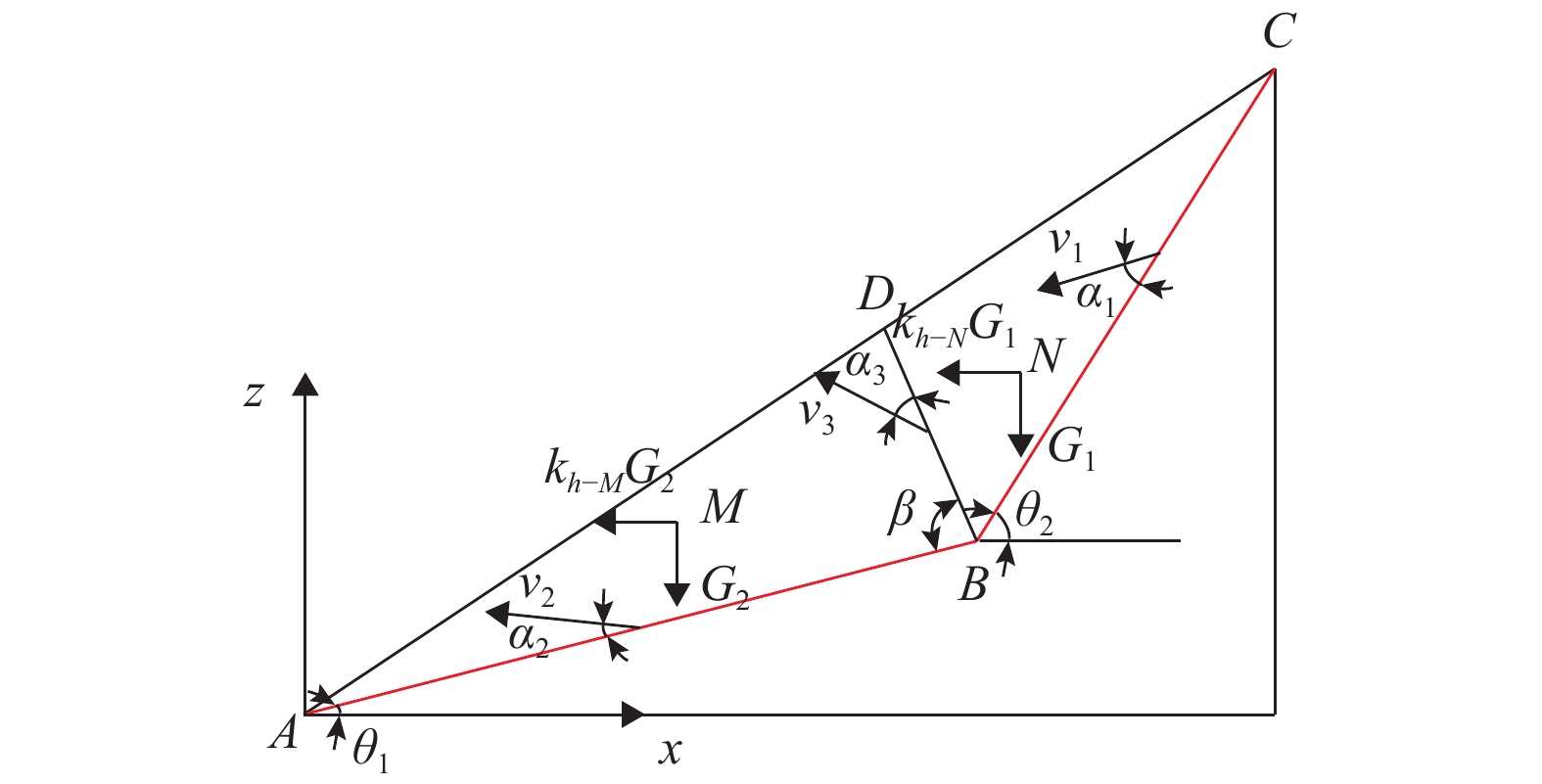
对于图1所示折线形滑面,建立图2所示双楔块破坏模式协调速度场. 图中:
$ {v_1} $ 、$ {v_2} $ 、$ {v_3} $ 分别为楔块CBD、ABD速度以及两者相对速度,即楔块沿着间断面BC、AB、BD的速度;$ {\alpha _1} $ 、$ {\alpha _2} $ 、$ {\alpha _3} $ 分别为速度$ {v_1} $ 、$ {v_2} $ 、$ {v_3} $ 与速度间断面BC、AB、BD之间夹角;$ \beta $ 为待定偏角.根据图2中的速度矢量关系,楔块之间的速度满足:
v3=v1sin(θ2−θ1+α2−α1)sin(β−α2−α3), v2=v1sin(π+θ1−β−θ2+α1+α3)sin(β−α2−α3). 根据假设条件,力学模型如图3所示. 图中:kh-M、kh-N为楔块ABD、BCD质心M、N 处水平地震加速度系数,可由式(1)求解;
$ {G_1} $ 、$ {G_2} $ 分别为楔块BCD、ABD重力. 图3模型外力为作用在质心M、N 处的楔块重力与水平地震力(方向向左),而内能的耗散主要来自速度间断面BC、AB、BD上的黏聚力.1) 外荷载功率
图3所示模型外功率为
Pe=P1+P2+P1h+P2h, 式中:
$ {P_1} $ 为楔块BCD重力所做外功率,如式(7);$ {P_2} $ 为楔块ABD重力所做外功率,如式(8);$ {P_{1{\rm{h}}}} $ 为楔块BCD地震力所做外功率,如式(9);$ {P_{2{\rm{h}}}} $ 为楔块ABD地震力所做外功率,如式(10).P1=G1v1sin(θ2−α1), (7) P2=G2v2sin(θ1−α2), (8) P1h=kh−NG1v1cos(θ2−α1), (9) P2h=kh−MG2v2cos(θ1−α2). (10) 如图(3)所示,当将坐标原点取为点A时,质心M、N的高度分别为
zM=zB+zD3, (11) zN=zB+zD+zC3, (12) 式中:点B、C、D 在z轴方向坐标zB、zC、zD可根据几何关系(d1、d2、h1、h2、θ1、θ2、β)求得.
2) 内能耗散功率
滑坡系统的内能耗散功率为
Pi = 3∑j=1cjFvjljcosαj, (13) 式中:cj为速度间断面上的黏聚力;vj为速度;lj为速度间断面的长度.
3) 安全系数
为求得安全系数,令Pe与Pi相等,则
P1+P2+P1h+P2h=3∑j=1cjFvjljcosαj. (14) 由于本文计算中通过拟动力法考虑了地震荷载的时程规律(式(1)),因此,式(14)中的安全系数大小不仅与β有关,还与时间t有关. 当时间t已知时,
F=F(β). (15) 对式(15)求极值,即可得到时间t的安全系数F.
2. 计算方法验证
2.1 规范法
为验证本文计算方法的可靠性,将本文计算值与摩根斯坦-普莱斯法进行对比. 与其他经典边坡极限平衡方法(简布法、毕肖普法、瑞典条分法、斯宾塞法等)相比,由于摩根斯坦-普拉斯法可针对多种破裂面形状,并在计算中同时考虑了力与力矩的平衡,因而,被公认为是目前最严谨的边坡稳定性计算方法[14 -15].
不同水平加速度峰值系数、边坡几何形状、抗剪强度下,本文计算的安全系数结果与摩根斯坦-普莱斯法的计算结果如表1所示. 表中:γ为滑体重度. 根据表1结果可知:本文方法与摩根斯坦-普莱斯法计算结果接近;两种方法求得的安全系数均随着水平峰值加速度的增大以及边坡强度的减小而减小. 另外,与本文极限分析法中仅需要求解一个能量方程不同,由于摩根斯坦-普莱斯法计算中将滑体分成n个单元,因而其需要求解3n个方程. 综上可知,本文方法可以在保证计算精度的同时,显著降低求解时间,提高计算速度.
表 1 计算结果Table 1. Calculation results工况 kP d1
/md2
/mh1
/mh2
/mγ
/(kN•m−3)c
/kPaφ
/(o)安全系数 本文方法 摩根斯坦-普莱斯法 1 0 11.59 4.95 3.11 4.95 20 6 30 2.02 2.00 2 0.2 11.59 4.95 3.11 4.95 20 6 30 1.35 1.32 3 0.4 11.59 4.95 3.11 4.95 20 6 30 0.99 0.97 4 0.2 11.59 4.95 3.11 4.95 20 6 20 1.05 1.03 5 0.2 9.78 3.54 2.08 3.54 20 6 20 1.22 1.19 注:表中本文方法计算结果为时间 t 内的最小值. 2.2 二楔块法
采用二楔块法,Qian等[16]对沿着衬垫滑动的垃圾场开展了稳定性研究,结果如表2所示.
由表2可知:与二楔块法计算值相比,本文方法计算值更接近有限元数值解. 另外,虽然与二楔块法相同,本文在计算中同样将边坡分成了两个楔块,但是计算中却仅需要求解一个能量方程,而二楔块法需要建立4个平衡方程.
表 2 计算结果Table 2. Calculation resultskP d1
/md2
/mh1
/mh2
/mγ
/(kN•m−3)c
/kPaφ
/(o)安全系数 本文方法 二楔块法[16] 有限元 0 53.56 90.18 1.03 30.00 10.2 0 17 1.36 1.35 1.39 3. 参数分析
为了分析水平加速度、土体强度(c、ϕ)与夹层形状等参数对边坡安全系数的影响,定义以下参数基准值:θ1=18°,θ2=42°,l2=9 m,l1=6 m,kp=0.3,水平加速度放大系数fa=1.3,f=2 Hz,ρ=2 200 kg/m3,c=5 kPa,φ=25°.
3.1 地震加速度
边坡安全系数除了采用式(4)表示,在极限分析法中也可采用式(16)表示[17].
F=PiPe. (16) 结合式(14)可知:F > 1,表明系统可产生的内能功率Pi大于外荷载功率Pe,即系统越稳定.
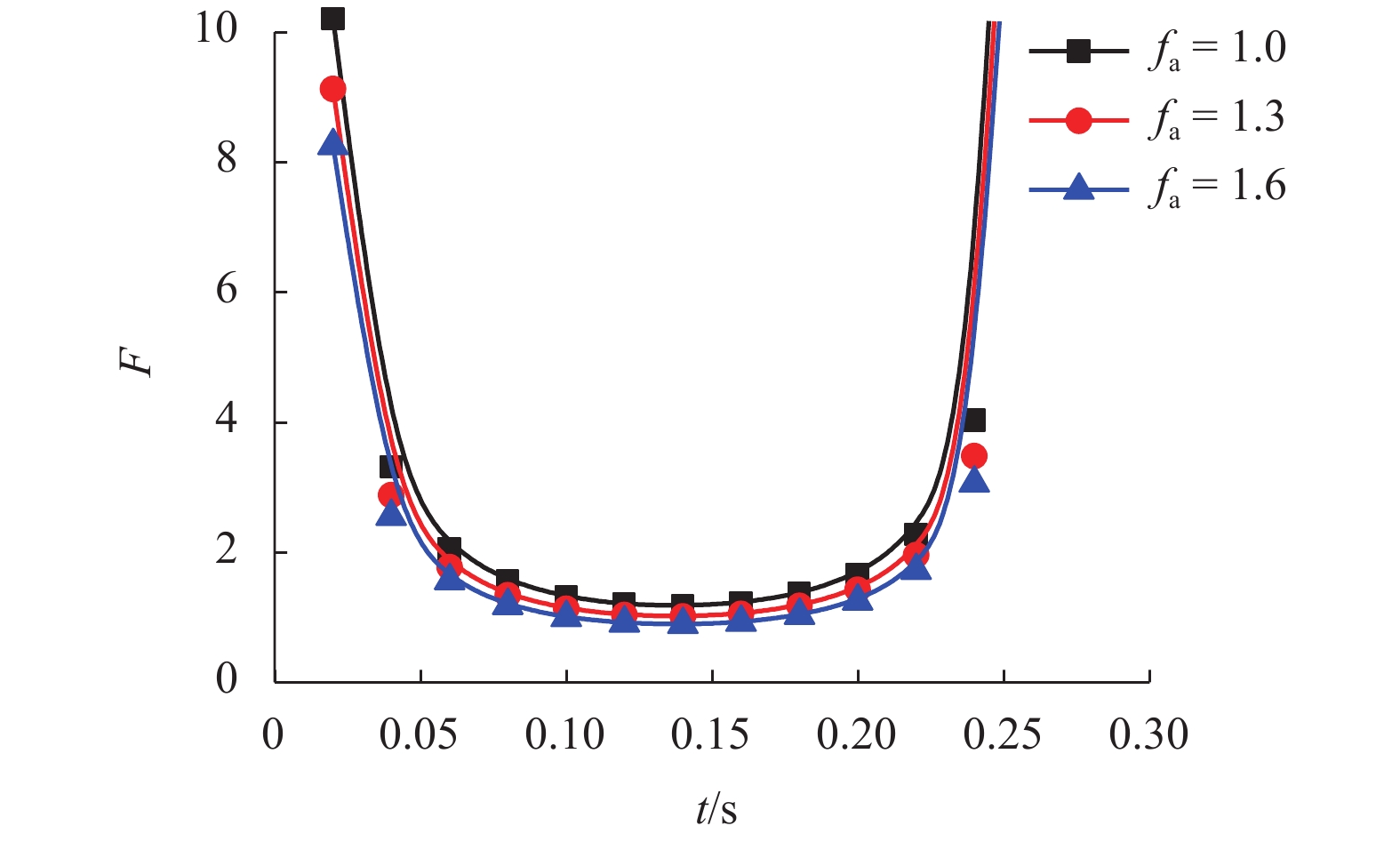
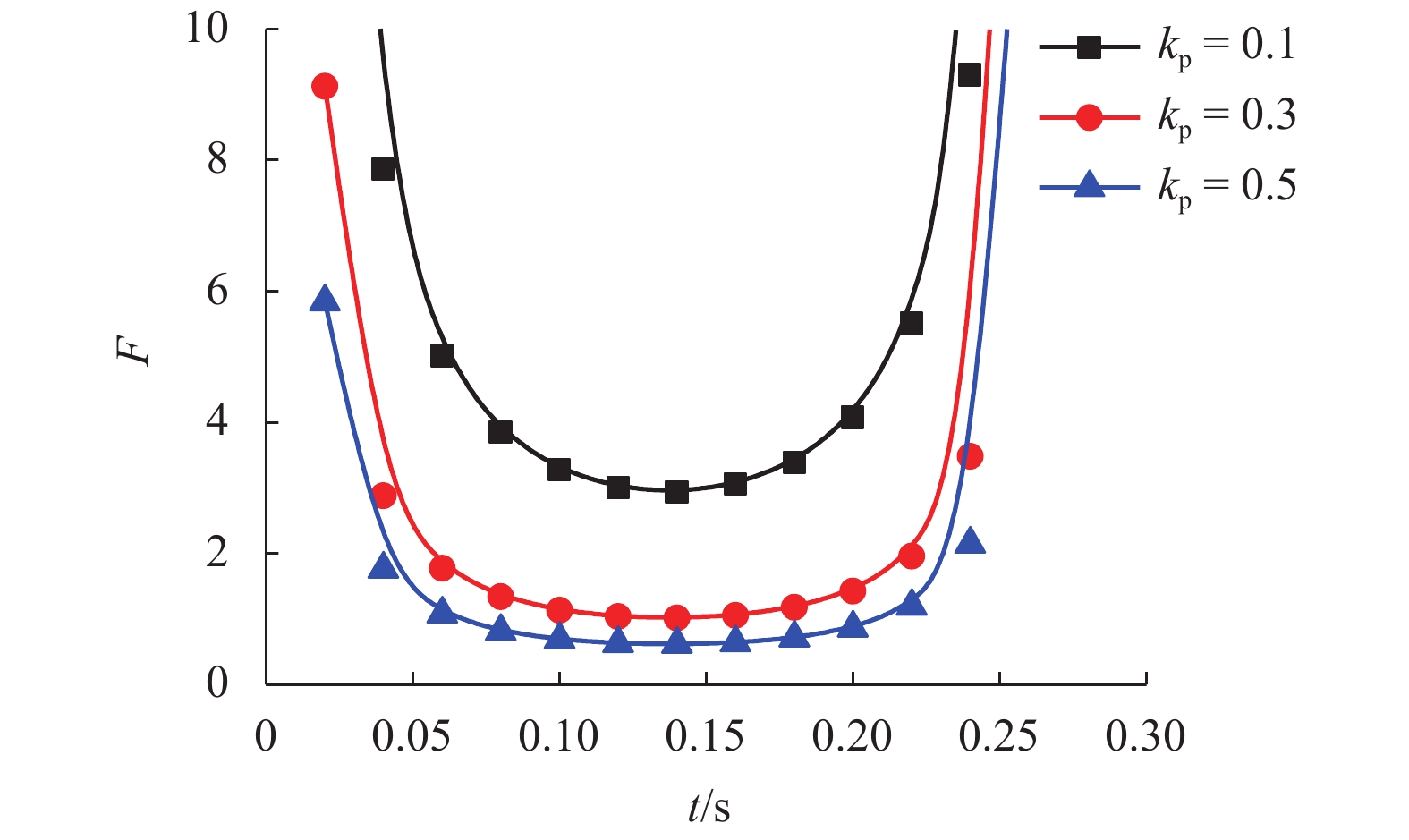
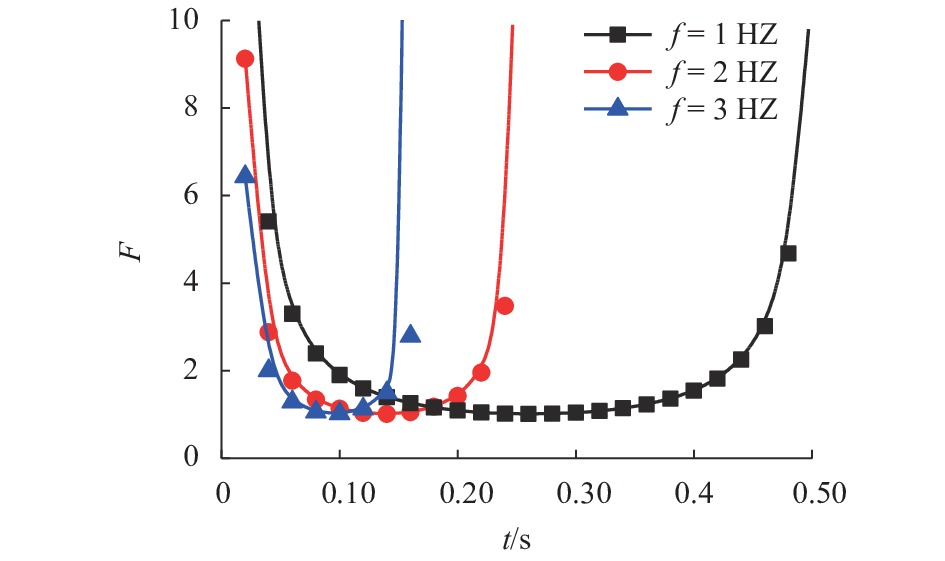
根据式(1),模型加速度系数大小与fa、kp、f等有关. 图4~6分别为F随fa、kp、f的变化规律.
不同于拟静力法中采用恒值峰值加速度系数进行求解计算,由于本文式(1)考虑了加速度随时间等的变化,因此,图4中本文计算求得的安全系数随着时间不断变化,并呈现先减小后增大的趋势. 出现上述变化规律原因为:式(1)表示的加速度系数随着时间呈先增大后减小的趋势,即安全系数的大小与加速度系数大小有关. 需要说明的是,尽管为了便于计算,本文中加速度的时程曲线被假定为简谐函数形式,但对于实际中任意已知的外荷载时程工况,本文方法同样适用. 上述结果表明:拟静力中采用单一的峰值加速度系数进行计算将可能导致设计较为保守. 除此之外,图4表明:F随着fa的增大而减小,其中fa=1.0,1.3,1.6时对应的最小安全系数分别为1.20、1.01、0.89.
与fa对F的影响类似,由图5可知:F 随着kp的增大而减小,即地震荷载的强度越大,滑坡稳定性越差. 对比图4与图5可知:相较于fa,kp对安全系数的影响更显著.
尽管本文在计算中考虑了地震波参数对安全系数的影响,但由于式(1)表示的拟动力法并非真正的动力法,因而计算中的地震波参数不能影响边坡的动力特性. 因为式(1)中fa、kp仅能改变加速度的数值大小,而不能影响加速度随时间的变化规律,因此图4与图5中各自的曲线形式未发生变化. 与之不同的是,f的变化将影响式(1)的曲线形状而不改变其数值大小. 结合图6可知:f 越大,周期则越短,即式(1)越早达到峰值,因此,F出现峰值的时刻越早;由于f的改变不影响F数值的大小,所以不同f时的最小安全系数均为1.01. 上述研究表明:当外荷载的频率越大时,结构可能越易提前产生破坏. 需要说明的是:理论上结构的动力响应大小与外荷载频率以及自身固有频率有关,即外荷载频率的变化将影响结构的稳定,但是由于本文计算中没有考虑滑坡体自身的固有频率,故未能体现出上述现象.
3.2 软弱夹层折线ABC形状
由于设计中通常关注的是地震峰值荷载时的安全系数,因而下文仅针对时间t内的安全系数最小值进行分析.
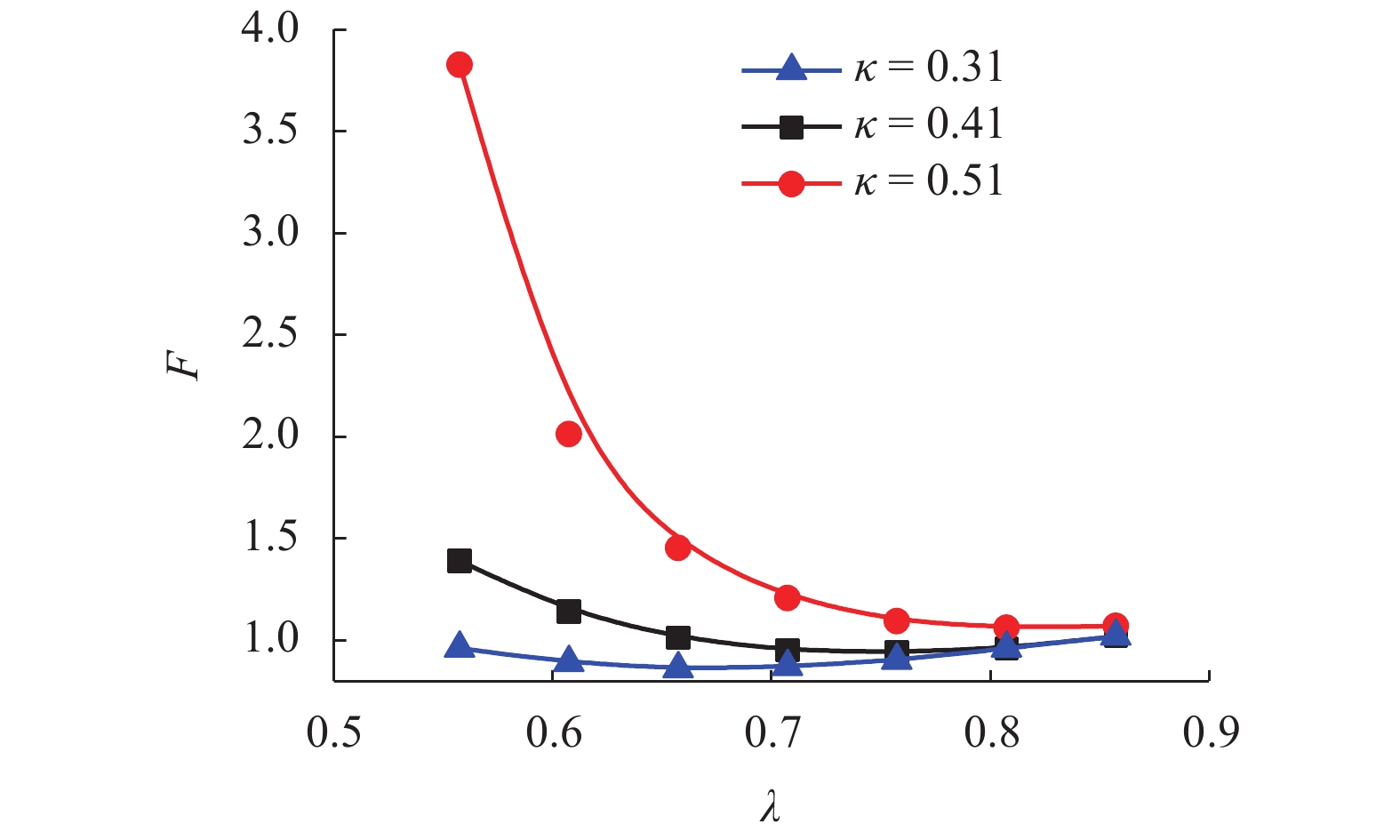
为了表示折线夹层形状(软弱夹层折线ABC的交点B)对滑坡安全系数大小的影响,将图1及图7中的几何参数无量纲化:
{λ=d1d1+d2,κ=h1h1+h2. (17) 图7为F随折线夹层形状的变化曲线. 当图中的点A与点C确定时,软弱夹层的形状主要由点B影响. 由图7可知:随着λ值的增大,即点B逐渐远离坡面,安全系数呈现先迅速减小后缓慢增大的趋势,上述现象表明当软弱夹层靠近坡面时,其对安全系数的影响更明显;由于当软弱夹层靠近坡顶时,滑体的面积较小,因而随着κ值的增大,即点B逐渐靠近坡顶,安全系数整体呈现增大的趋势.
3.3 填土强度
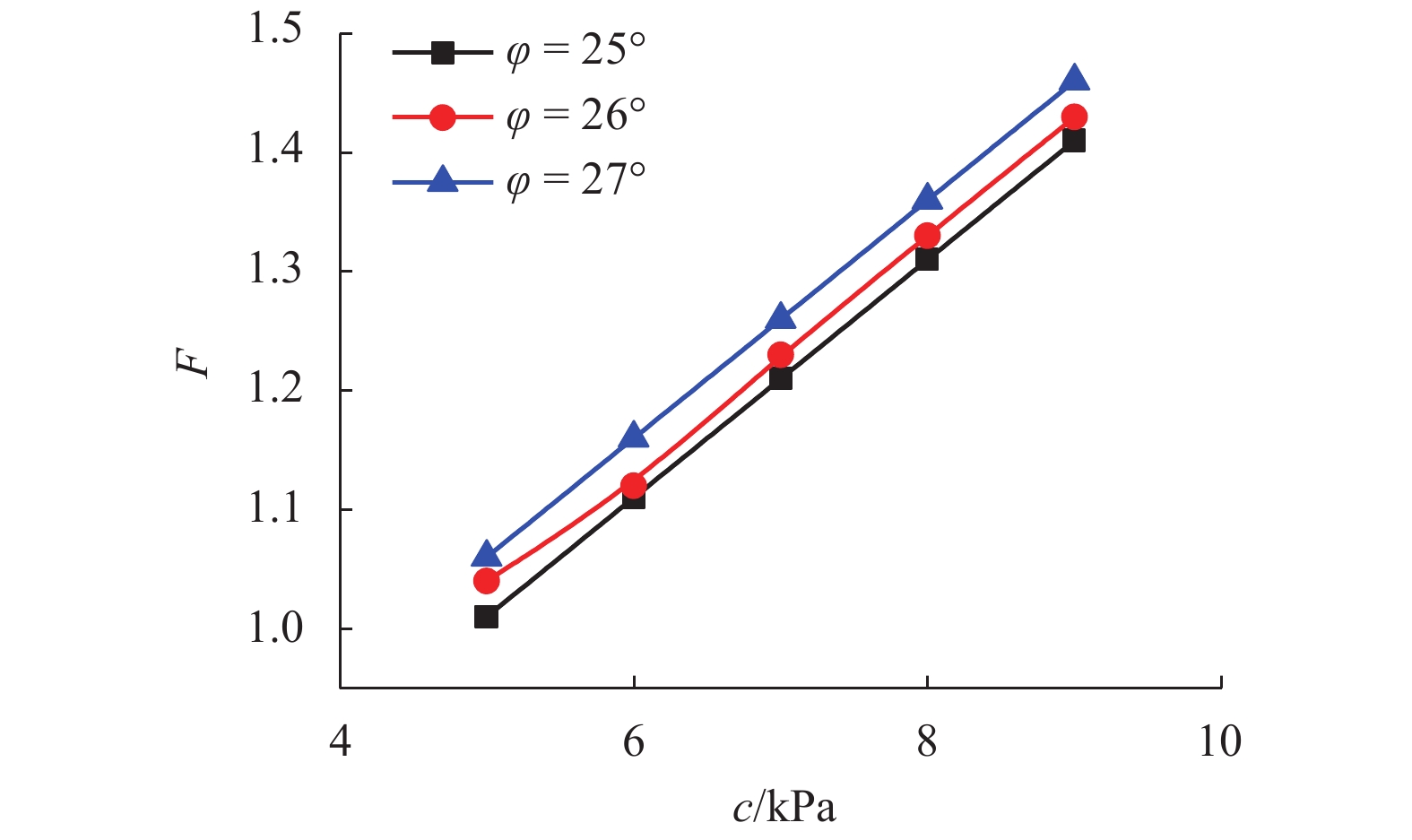
图8为F与土体强度的关系曲线. 由图可知: F随着c的增大线性增大;随着摩擦角的增大安全系数也增大.
4. 结 论
采用解答更严密、求解更简捷的极限分析法建立了含软弱夹层边坡的稳定性计算模型,并通过既有极限平衡法验证了本文方法的准确性. 通过参数分析,研究了荷载、夹层形状与强度对边坡稳定性的影响:
1) 与加速度放大系数相比,外荷载峰值大小对安全系数的影响更大;由于当外荷载频率越大时,外荷载更早达到峰值,此时边坡安全系数先达到最小值.
2) 随着软弱夹层逐渐远离坡面,安全系数呈先迅速减小后缓慢增大的趋势. 当软弱夹层越靠近坡面与坡顶时,安全系数的大小对其形状的变化越敏感.
3) 安全系数随着软弱夹层黏聚力的增大近似线性增大;随着软弱夹层摩擦角的增大,其对安全系数的影响逐渐增大.
-
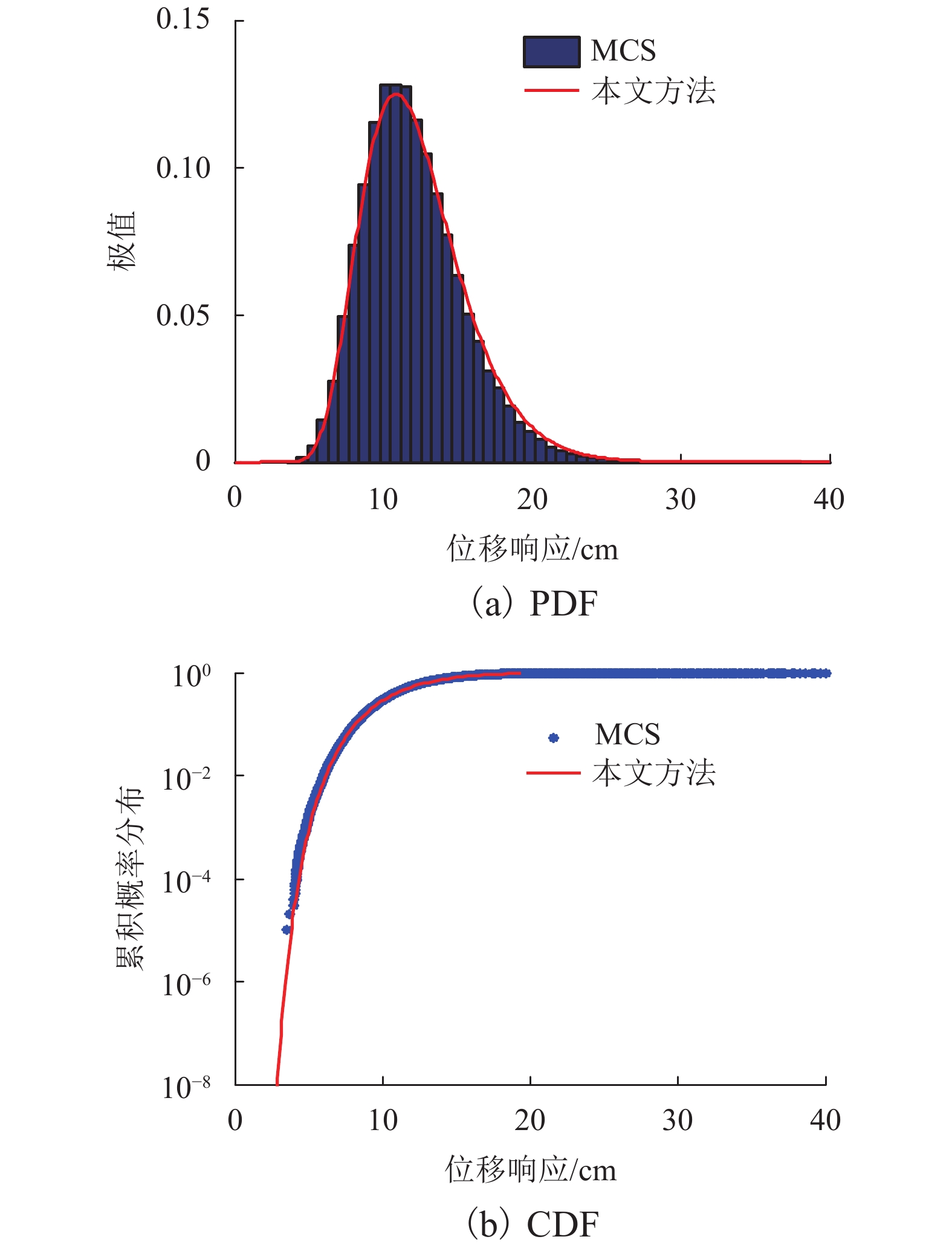
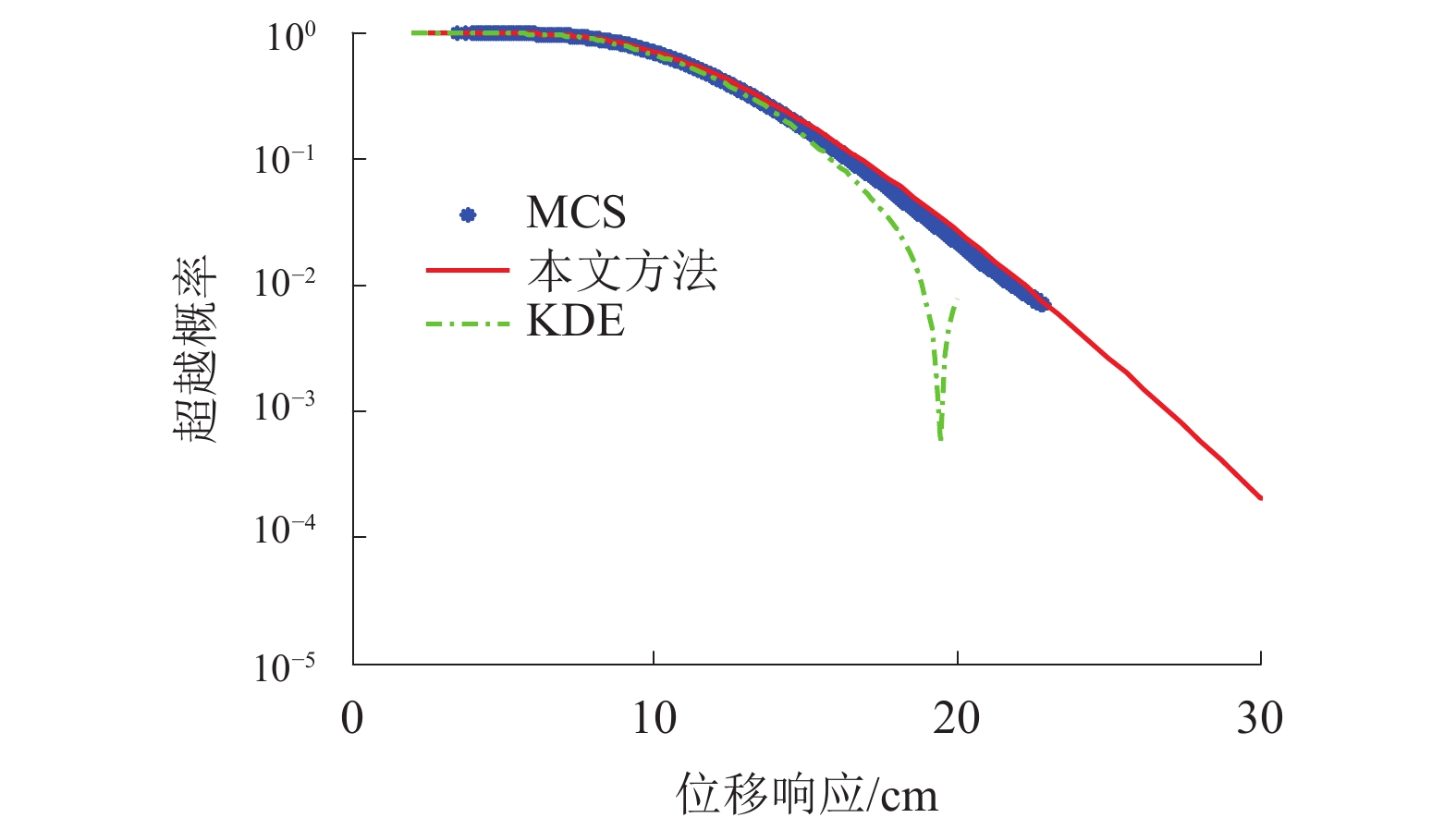
表 1 单自由度系统地震响应估计值与MCS的比较
Table 1. Comparison of estimated SDOF system seismic response with MCS
方法 μ $\sigma ' $ E(X (0.25)) E(X (1.20)) LHS 12.17 3.454 1.854 3 20.250 0 MCS(105 次) 12.05 3.424 1.849 7 20.000 0 相对误差/% 1.04 0.88 0.25 1.26 表 2 单自由度系统地震响应极值最大熵分布参数
Table 2. MaxEnt distribution parameters of SDOF system
分布 r λr αr $M_X^{ {(\alpha _r)} } $ I(λ,α) 1 0 14.537 0 2 1 1.672 1 0.863 9 8.622 1 2.603 4 3 2 −13.279 3 0.303 9 2.119 4 4 3 86.647 8 −1.617 5 0.020 7 表 3 框架结构的集中质量和层间刚度
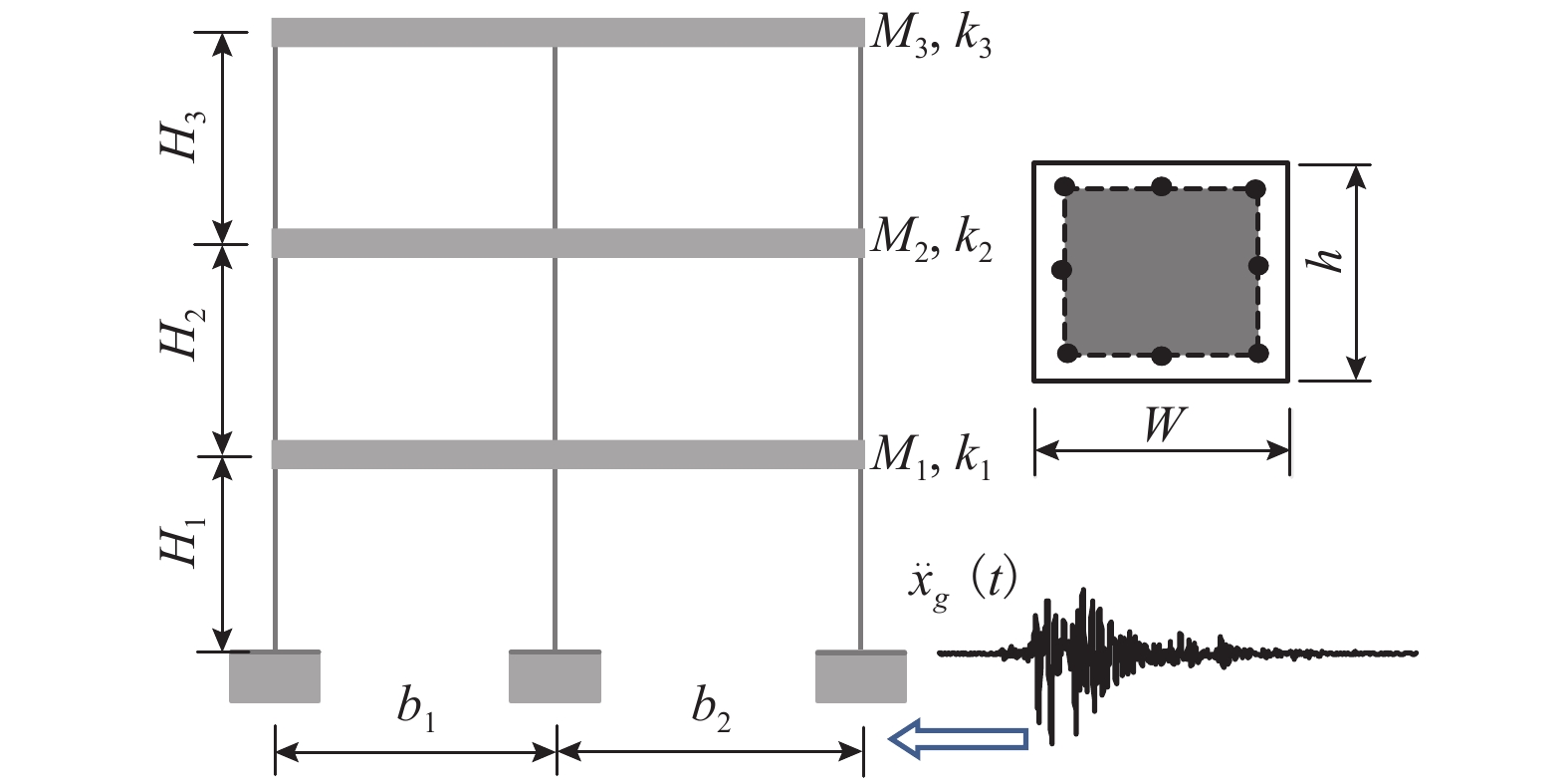
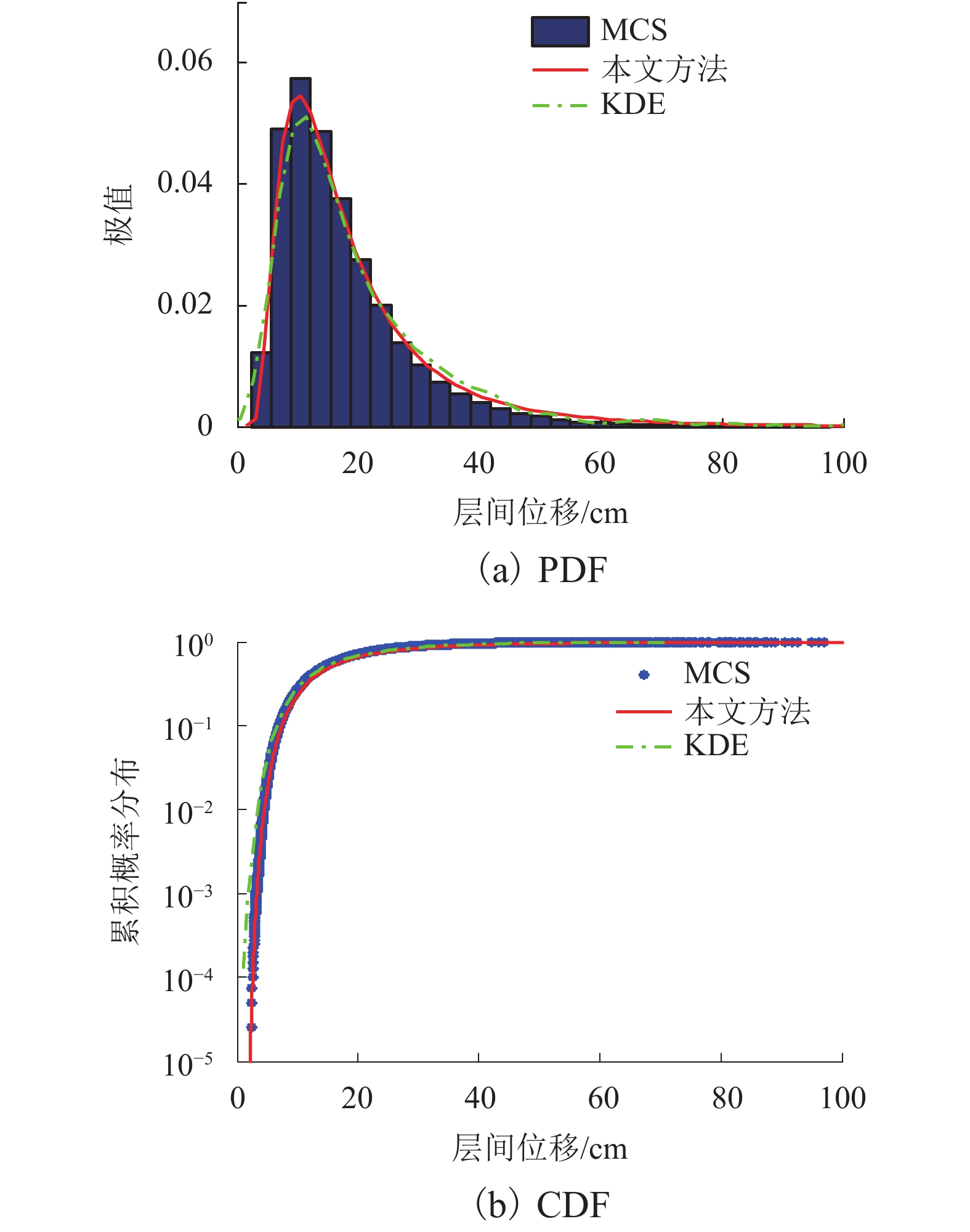
Table 3. Lumped mass and lateral inter-story stiffness of the frame
变量 均值 变异系数 分布类型 M1 2.71 × 105 kg 正态 M2 2.71 × 105 kg 0.10 M3 2.71 × 105 kg k1 2.42 × 107 N/m k2 2.42 × 107 N/m 0.15 k3 2.42 × 107 N/m -
CHANG Z, SUN X, ZHAI C, et al. An improved energy-based approach for selecting pulse-like ground motions[J]. Earthquake Engineering & Structural Dynamics, 2016, 45(14): 2405-2411. SOMERVILLE P G, SMITH N F, GRAVES R W, et al. Modification of empirical strong ground motion attenuation relations to include the amplitude and duration effects of rupture directivity[J]. Seismological Research Letters, 1997, 68(1): 199-222. doi: 10.1785/gssrl.68.1.199 郑史雄,陈志强,陈志伟,等. 近场多脉冲地震作用下高墩桥梁地震响应分析[J]. 西南交通大学学报,2019,54(5): 897-907.ZHENG Shixiong, CHEN Zhiqiang, CHEN Zhiwei, et al. Seismic response analysis of high-pier bridge under near-fault multiple pulse record excitation[J]. Journal of Southwest Jiaotong University, 2019, 54(5): 897-907. 武芳文,孟园英,陈月,等. 大跨度斜拉桥地震易损性及可恢复性分析[J]. 西南交通大学学报,2020,55(1): 126-133.WU Fangwen, MENG Yuanying, CHEN Yue, et al. Analysis of seismic fragility and recoverability of long-span cable-stayed bridge[J]. Journal of Southwest Jiaotong University, 2020, 55(1): 126-133. GRIGORIU M, SAMORODNITSKY G. Reliability of dynamic systems in random environment by extreme value theory[J]. Probabilistic engineering mechanics, 2014, 38: 54-69. doi: 10.1016/j.probengmech.2014.08.005 CHEN J B, LI J. The extreme value distribution and dynamic reliability analysis of nonlinear structures with uncertain parameters[J]. Structural Safety, 2007, 29(2): 77-93. doi: 10.1016/j.strusafe.2006.02.002 GOLLER B, PRADLWARTER H J, SCHUËLLER G I. Reliability assessment in structural dynamics[J]. Journal of Sound & Vibration, 2013, 332(10): 2488-2499. MELCHERS R E. Importance sampling in structural system[J]. Structural Safety, 1989, 6(1): 3-10. doi: 10.1016/0167-4730(89)90003-9 AU S K, BECK J L. Subset simulation and its application to seismic risk based on dynamic analysis[J]. Journal of Engineering Mechanics, 2003, 129(8): 901-917. doi: 10.1061/(ASCE)0733-9399(2003)129:8(901) XU J, DING Z, WANG J. Extreme value distribution and small failure probabilities estimation of structures subjected to non-stationary stochastic seismic excitations[J]. Structural Safety, 2018, 70: 93-103. doi: 10.1016/j.strusafe.2017.10.007 LOW Y M. A new distribution for fitting four moments and its applications to reliability analysis[J]. Structural Safety, 2013, 42(3): 12-25. MICHAEL S. Large sample properties of simulations using latin hypercube sampling[J]. Technometrics, 1987, 29(2): 143-151. doi: 10.1080/00401706.1987.10488205 LI S, ZHANG F, WANG J, et al. Effects of near-fault motions and artificial pulse-type ground motions on super-span cable-stayed bridge systems[J]. Journal of Bridge Engineering, 2016, 22(3): 1-17. YANG D, ZHOU J. A stochastic model and synthesis for near-fault impulsive ground motions[J]. Earthquake Engineering & Structural Dynamics, 2015, 44(2): 243-264. ZHANG X, PANDEY M D. Structural reliability analysis based on the concepts of entropy,fractional moment and dimensional reduction method[J]. Structural Safety, 2013, 43: 28-40. doi: 10.1016/j.strusafe.2013.03.001 XIONG F, GREENE S, CHEN W, et al. A new sparse grid based method for uncertainty propagation[J]. Structural & Multidisciplinary Optimization, 2010, 41(3): 335-349. SOBCZYK K, TRCEBICKI J. Approximate probability distributions for stochastic systems:maximum entropy method[J]. Computer Methods in Applied Mechanics and Engineering, 1999, 168(1/2/3/4): 91-111. JAYNES E T. Information theory and statistical mechanics[J]. Physical Review, 1957, 106(4): 620. doi: 10.1103/PhysRev.106.620 CHEN B, HU J, ZHU Y. Computing maximum entropy densities:a hybrid approach[J]. Signal Processing:An International Journal(SPIJ), 2010, 4(2): 114. MIRANDA E, RUIZ-GARCÍA J. Evaluation of approximate methods to estimate maximum inelastic displacement demands[J]. Earthquake Engineering & Structural Dynamics, 2010, 31(3): 539-560. IWAN W D. Estimating inelastic response spectra from elastic spectra[J]. Earthquake Engineering & Structural Dynamics, 2010, 8(4): 375-388. 欧进萍, 王光远. 结构随机振动[M]. 北京: 高等教育出版社, 1998: 295-303. 期刊类型引用(1)
1. 李正良,王成,王涛,方智远. 下击暴流作用下直立锁缝屋面系统抗风揭可靠度评估. 湖南大学学报(自然科学版). 2022(09): 90-99 .  百度学术
百度学术其他类型引用(5)
-





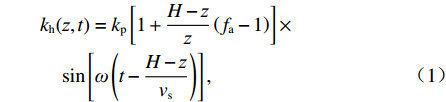




 下载:
下载:
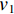
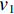
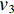
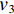
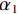
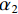
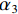
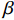

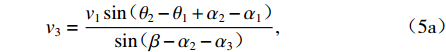
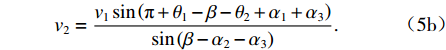

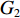

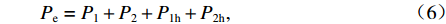
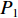


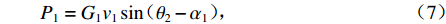
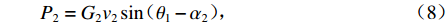
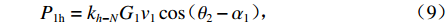
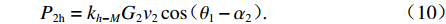


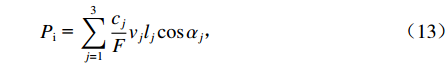
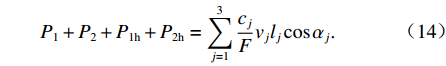








 下载:
下载:
 百度学术
百度学术