单级光伏并网多逆变器系统并联交互影响分析

陈维荣; 王璇; 李奇

doi:10.3969/j.issn.0258-2724.20180900

单级光伏并网多逆变器系统并联交互影响分析

doi: 10.3969/j.issn.0258-2724.20180900

详细信息

作者简介:
陈维荣(1965—)，男，教授，博士生导师，研究方向为电力系统及其自动化、燃料电池技术及其应用， E-mail： wrchen@swjtu.cn

通讯作者:
李奇(1984—)，男，教授，博士生导师，研究方向为分布式发电并网技术、电力系统无功优化， E-mail： liqi0800@163.com

中图分类号: V221.3
计量
- 文章访问数: 683
- HTML全文浏览量: 690
- PDF下载量: 23
- 被引次数: 0
出版历程
- 收稿日期: 2018-11-12
- 修回日期: 2019-05-22
- 网络出版日期: 2020-03-06
- 刊出日期: 2020-08-01

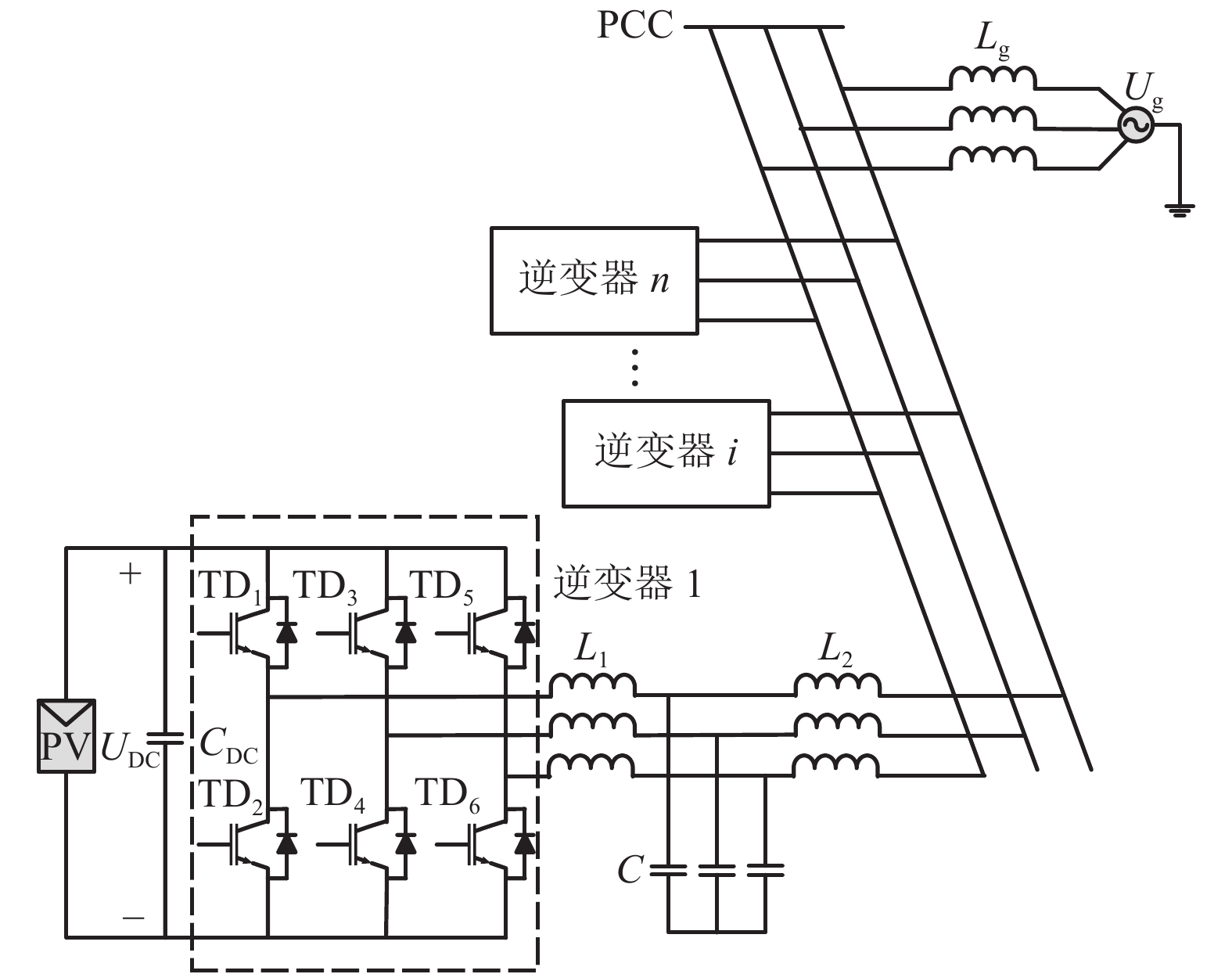
Parallel Interaction Influence of Single-Stage Photovoltaic Grid-Connected Multi-Inverter System

摘要

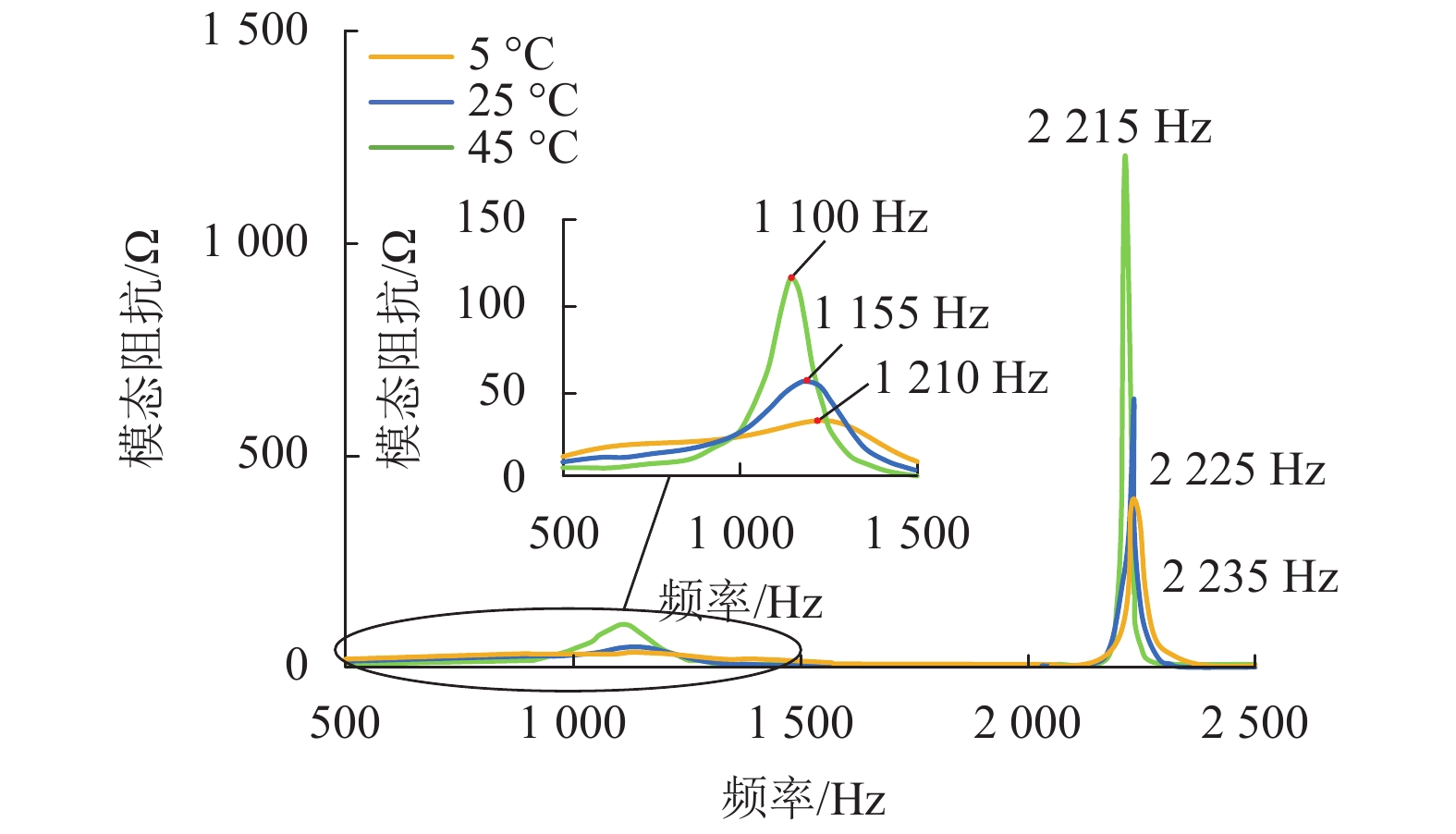
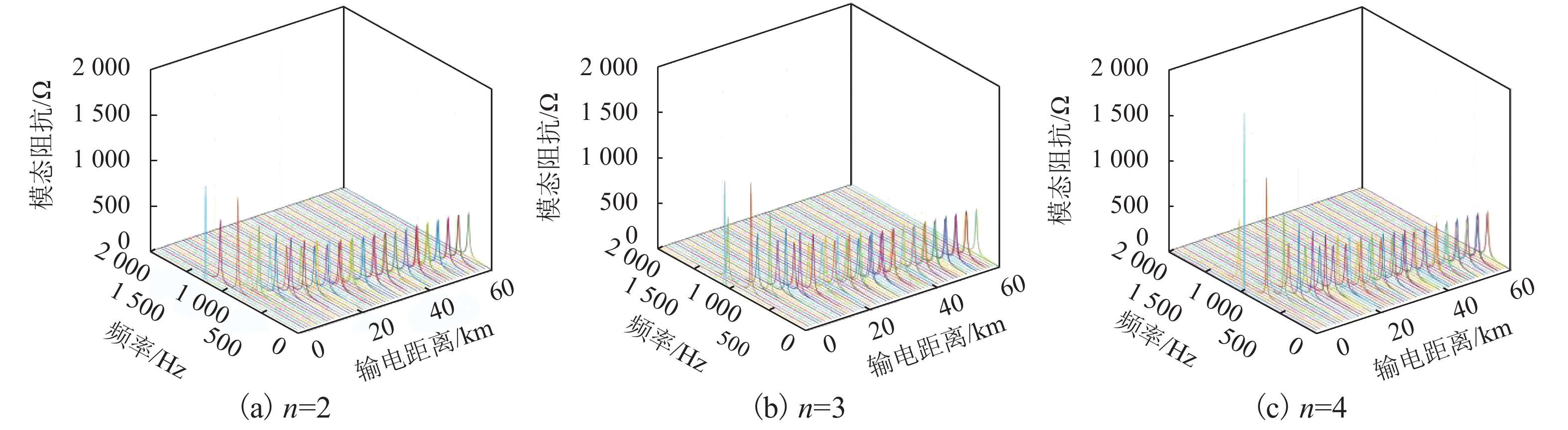
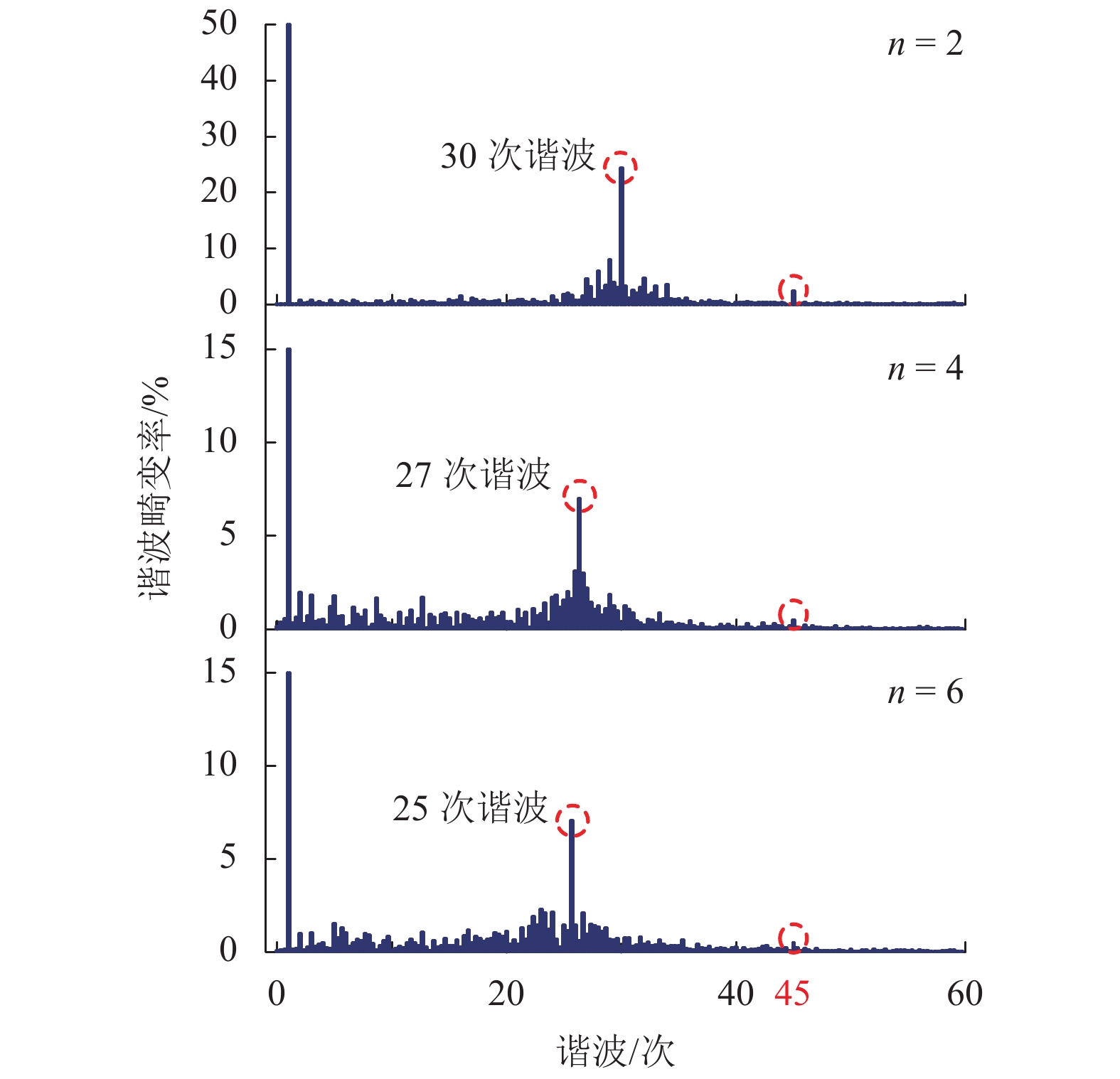
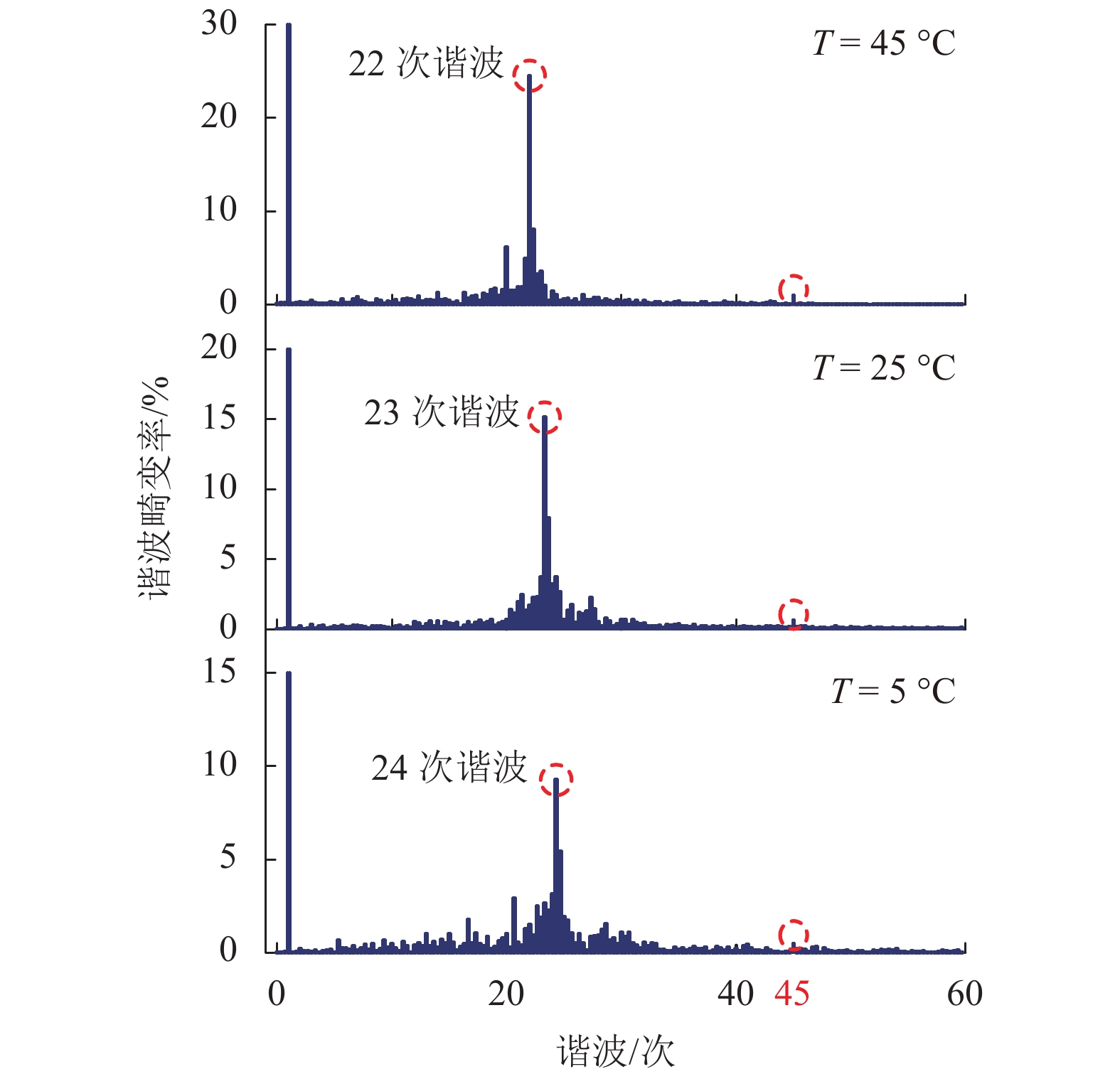
摘要: 在弱电网条件下，多逆变器间、多逆变器与电网间的动态交互作用影响着电力系统的电能质量和稳定性，易引发谐波谐振等问题. 为了研究单级光伏并网多逆变器系统的谐波谐振特性，考虑光伏源与系统之间的交互作用，采用模态分析法对谐振问题进行了系统的分析与讨论. 首先，根据三相单级式光伏并网系统结构及控制策略，建立了多逆变器戴维南等效模型；其次，通过构建多逆变器系统节点导纳矩阵，应用了一种能够确定系统谐振频率、谐振中心以及各节点参与程度的模态分析方法，从逆变器台数、外界环境、输电距离3个方面研究了系统谐振特性与变化规律；最后，基于MATLAB/Simulink仿真平台，通过搭建三相单级式光伏并网多逆变器系统仿真模型，验证了模态分析法的正确性与有效性. 研究结果表明：当逆变器台数增加时，低频谐振频率呈降低趋势，依次为30、27、25次谐波，高频谐振频率为2 230 Hz保持不变；当外界环境温度降低时，低频谐振频率逐渐升高，依次为22、23、24次谐波，高频谐振频率固定约为2 225 Hz；当输电距离增长时，低频与高频谐振频率均逐渐降低且变得接近.
- 谐波谐振 /
- 模态分析 /
- 多逆变器 /
- 单级光伏
Abstract: Under the condition of weak grid, the dynamic interactions between multi-inverters and between multi-inverters and the grid affect the power quality and stability of the power system, which is likely to cause harmonic resonance. In order to study the harmonic resonance characteristics of the single-stage photovoltaic grid-connected multi-inverter system, the modal analysis method is used for systematical analysis and discussion on the resonance problem while the interaction between the photovoltaic generation and the system is considered. Firstly, according to the structure and control strategy of three-phase single-stage photovoltaic grid-connected system, the Thevenin equivalent model for the multi-inverter system is established. Secondly, a modal analysis method is applied, which can determine the system resonance frequency, resonance center and the participation degree of each node by constructing node admittance matrix of the multi-inverter system. The resonance characteristics and variation laws of the system are studied from three aspects: the number of inverters, external environment and transmission distance. Finally, with the use of MATLAB/Simulink simulation platform, the correctness and effectiveness of the modal analysis method are validated by a simulation model of a three-phase single-stage photovoltaic grid-connected multi-inverter system. The results show that when the number of inverters increases, the low resonance frequency tends to decrease, which is 30th, 27th, and 25th harmonics respectively, while the high resonance frequency remains unchanged at 2 230 Hz. When the ambient temperature decreases, the low resonance frequency increases gradually, which is 22th, 23th, and 24th harmonics respectively, and the high resonance frequency is stable at about 2 225 Hz. When the transmission distance increases, the low and high resonance frequencies gradually decrease and become close to each other.
- harmonic resonance /
- modal analysis /
- multi-inverter /
- single-stage photovoltaic

HTML全文

逆变回馈装置不但可以有效利用城轨列车再生制动能量，还可以改善城轨牵引供电系统网压水平，近些年国内广泛投入使用^[1-2]. 逆变回馈装置容量配置及安装位置是影响系统节能效果评估的重要因素，装置选址定容等问题是现阶段亟需解决的问题之一^[3-4].

针对城轨再生制动能量利用装置定容选址的优化问题，是现阶段研究的热点. 文献[5]根据地铁列车全线对向行驶时供电臂和走行轨等效电阻的损耗功率最小对逆变回馈装置进行选址，由于实际全线牵引变电所数量多、计算量大，该法收敛速度较慢. 文献[6-7]考虑超级电容储能装置的节能电量与投资成本，同时优化装置能量管理策略控制参数，结合城轨供电仿真平台与遗传算法，实现了多目标同时优化. 多目标优化问题受各个目标量纲属性不同的影响，很难找到一组同时满足所有目标最优的解，通常存在一个Pareto最优解集，其各组非劣解之间互不支配，无法在优化任何目标的同时不削弱其他目标^[8]. 这一多目标优化问题的求解思想常与智能算法结合以搜索非劣解集.

在利用遗传算法求解多目标优化问题上，相关文献展开了研究. 吴广宁等^[9]在牵引变电所接地网优化设计上对传统遗传算法做出了改进，添加适应度函数并采用自适应算法根据适应度值动态调整交叉概率和变异概率，避免算法进入局部最优解. Deb等^[10]为进一步提高遗传算法的计算效率和鲁棒性，在NSGA （non-dominated sorting genetic algorithm）的基础上提出了一种带精英策略的快速非支配排序遗传算法（fast NSGA-Ⅱ）. 文献[11-12]验证了NSGA-Ⅱ相对传统遗传算法和并行粒子群算法具有计算精度高、收敛速度快的优点.

本文首先分析了含逆变回馈装置的系统级节能指标，并建立城轨逆变回馈装置定容选址多目标优化模型，其次将考虑逆变回馈装置周期性间歇工作制的城轨交直流混合潮流算法与NSGA-Ⅱ结合求解多目标函数的Pareto解集，采用基于信息熵的序数偏好法（technique for order preference by similarity to ideal solution，TOPSIS）归一化各目标函数值，计算相对理想距离，进而在Pareto解集中筛选出逆变回馈装置选址定容最优方案. 最后以广州某地铁线路为例进行仿真分析，验证算法有效性.

1. 优化模型

1.1 目标函数

从逆变回馈装置的投资成本以及逆变回馈装置对城轨牵引供电系统节能效果两个角度，建立逆变回馈装置定容选址优化模型.

1）逆变回馈装置投资成本. 受地铁线路坡度及曲线信息影响，列车在各站间产生的再生制动能量不同，考虑逆变回馈装置经济投资因素，应在各牵引所安装不同容量的逆变回馈装置. 选择全线逆变回馈装置优化容量配置投资总成本的相反数作为目标函数f₁（X），如式（1）.

$\left\{ {\begin{array}{l} {f_{\rm{1}}}(X) = {\rm{ - }}\displaystyle\sum\limits_{i = 1}^{{N}_{\rm{all}}} {{c_{{\rm{EFS,}}i}}} , \\ X = \{ {x_i}\} , \\ \end{array} } \right.$

(1)

式中：${c_{{\rm{EFS,}}i}}$为第i个牵引所内逆变回馈装置投资成本；N_all为全线逆变回馈装置投入总数量；X为全线各牵引所逆变回馈装置容量配置集合；x_i为第i个牵引所内逆变回馈装置容量.

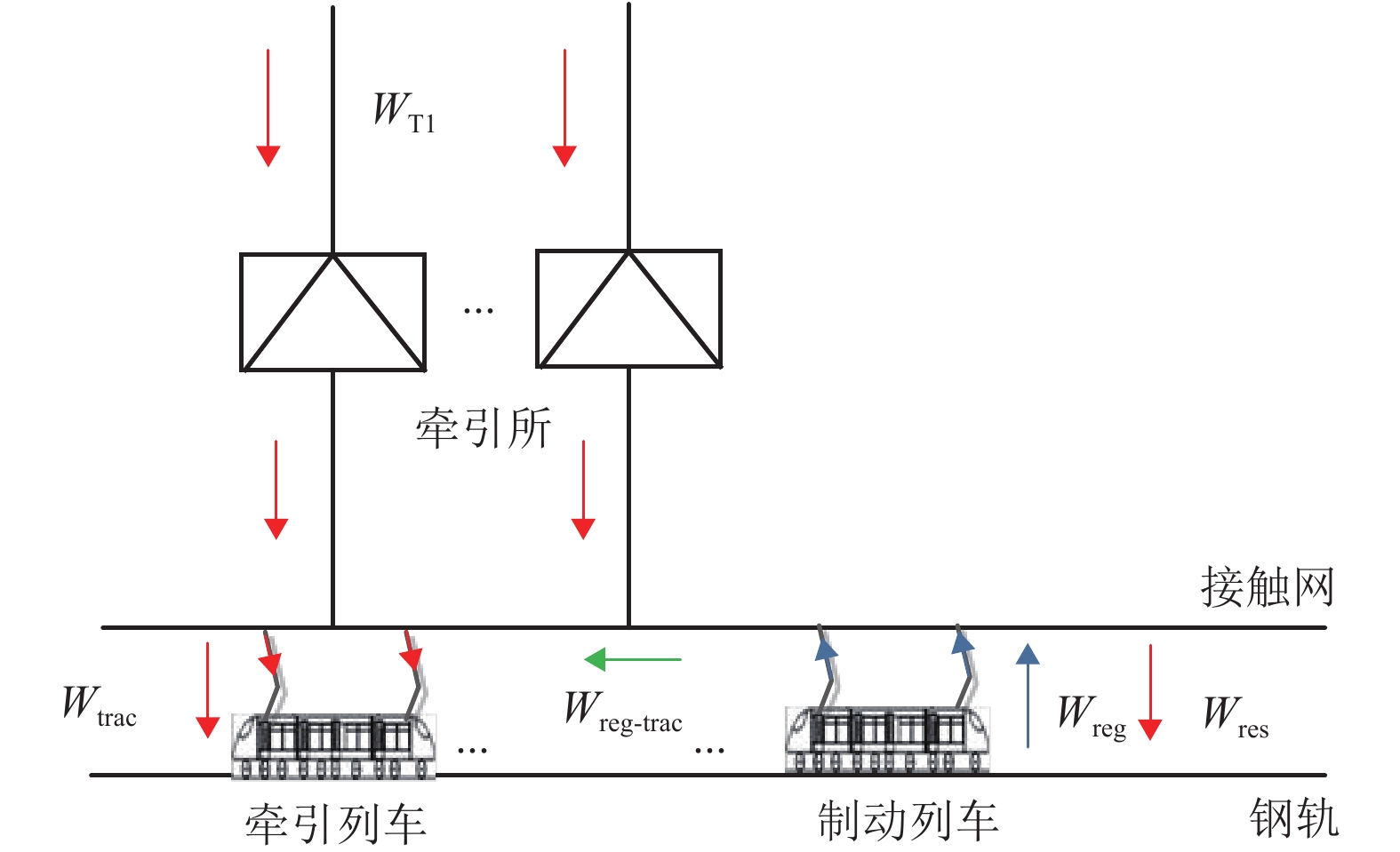
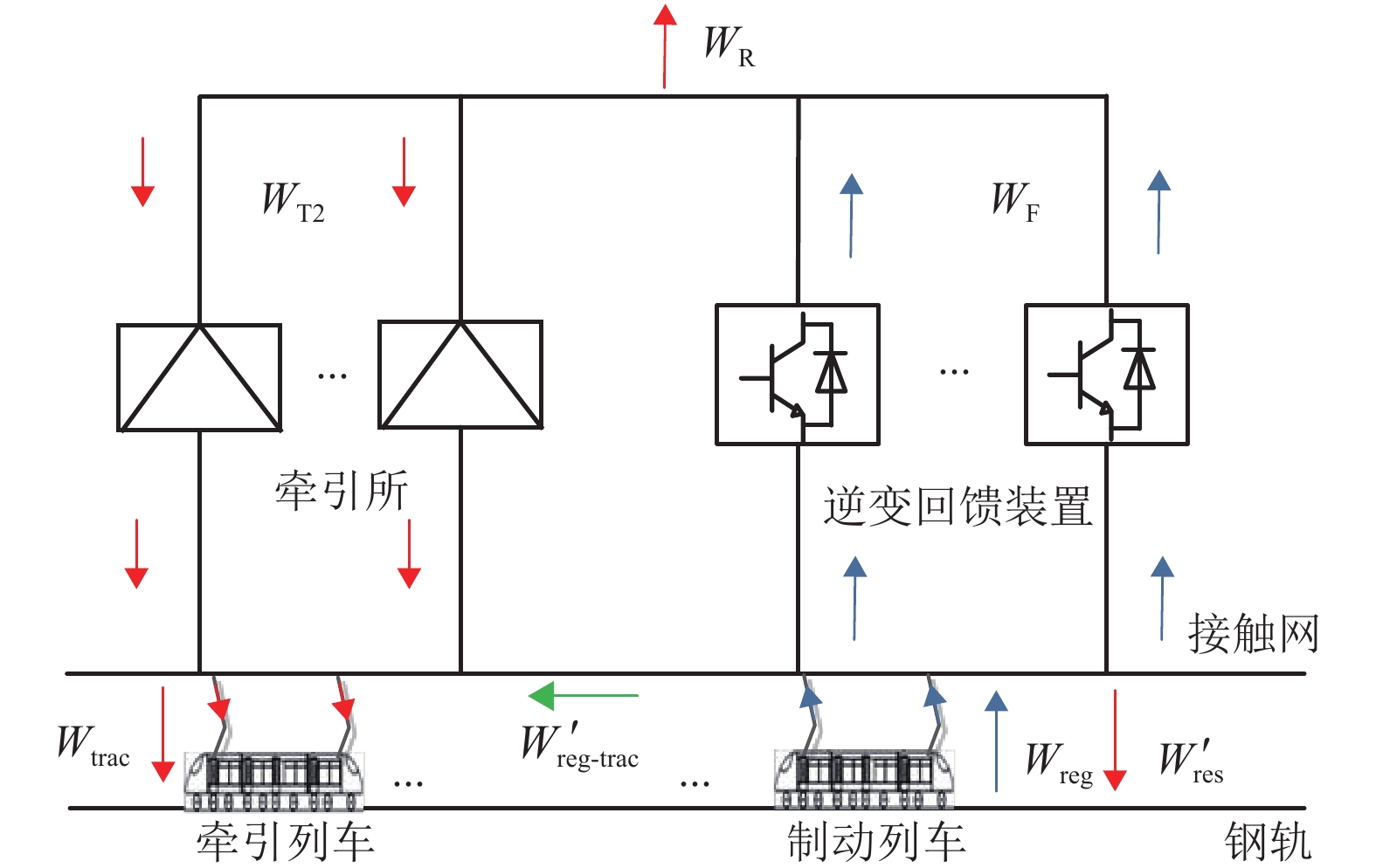
2）系统级节能率. 全线不安装逆变回馈装置的城轨牵引供电系统作为参考系统，其系统能量流向如图1所示. 安装逆变回馈装置后，城轨牵引供电系统能量流向如图2所示.

图 1 参考系统能量流向示意

Figure 1. Energy flow direction of reference system

下载: 全尺寸图片幻灯片

图 2 安装逆变回馈装置的系统能量流向示意

Figure 2. Energy flow direction for installation of inverter feedback devices

下载: 全尺寸图片幻灯片

图1中：W_T1为全线牵引所牵引能耗之和；W_trac为全线列车牵引总能耗；W_reg为全线再生制动总能量；$W_{{\rm{reg-trac}}}$为全线被邻车吸收再生制动能量之和；W_res为全线消耗在车载制动电阻上的再生制动能量之和. 图2中：W_T2为全线牵引所牵引能耗之和；W_F为全线被逆变回馈装置反馈再生制动能量之和；W_R为返送主所逆功率；$W_{{\rm{reg-trac}}}'$为全线被邻车吸收再生制动能量之和；$W_{{\rm{res}}}' $为全线消耗在车载制动电阻上的再生能量之和.

全线牵引能耗及再生能量关系如式（2）、（3）.

$\left\{ {\begin{array}{l} {W_{{\rm{trac}}}} = {W_{{\rm{T}}1}} + {W_{{\rm{reg - trac}}}}, \\ {W_{{\rm{res}}}} = {W_{{\rm{reg}}}} - {W_{{\rm{reg - trac}}}}; \\ \end{array} } \right.$

(2)

$\left\{ {\begin{array}{l} {W_{{\rm{trac}}}} = {W_{{\rm{T}}2}} + {W'_{{\rm{reg - trac}}}}, \\ {W'_{{\rm{res}}}} = {W_{{\rm{reg}}}} - {W'_{{\rm{reg - trac}}}} - {W_{\rm{F}}}. \\ \end{array}} \right.$

(3)

相对于参考系统，投入逆变回馈装置后全线车载制动电阻消耗的能量变化即为列车运行所节省的总电量W，如式（4）.

$\begin{split} &W{\rm{ = }}{W_{{\rm{res}}}} - {W'_{{\rm{res}}}} - {W_{\rm{R}}} = {W'_{{\rm{reg - trac}}}} - {W_{{\rm{reg - trac}}}}{\rm{ + }} {W_{\rm{F}}} - {W_{\rm{R}}} =\\ &\quad {W_{{\rm{T}}1}} - \left( {{W_{{\rm{T}}2}} - {W_{\rm{F}}}} \right) - {W_{\rm{R}}}. \\[-13pt] \end{split}$

(4)

计及主所逆功率返送，W_R未被城轨牵引供电系统利用，因此式（4）中没将W_R计入总节省电量中.

定义投入逆变回馈装置后列车运行所节省的总电量与参考系统下全线牵引所输出能耗的比值为系统级节能率，选择其作为目标函数f₂（X），如式（5）.

$\begin{split} &{f_{\rm{2}}}(X) = \frac{W}{{{W_{{\rm{T}}1}}}} \times 100{\text{%}} =\\ &\quad \frac{{{W_{{\rm{T}}1}} - \left( {{W_{{\rm{T}}2}} - {W_{\rm{F}}}} \right) - {W_R}}}{{{W_{{\rm{T}}1}}}} \times 100{\text{%}}. \end{split}$

(5)

综合考虑逆变回馈装置投资成本相反数以及系统级节能率，可表达为式（6）所示的城轨牵引供电系统逆变回馈装置定容选址多目标优化问题.

$\left\{ {\begin{array}{l} \max {V}(j) = \left\{ {{f_1}(X),{f_2}(X)} \right\}, \\ {\rm{s.t.}}\quad X \in \varepsilon , \\ \end{array}} \right.$

(6)

式中：V(j)为优化目标，由子目标函数f₁(x)和f₂(x)组成，表示逆变回馈装置容量配置Pareto方案；j为方案数；X属于可行域ε，是以0.5 MW为步长的随机离散变量集合^[13].

1.2 约束条件

求解上述多目标函数需满足逆变回馈装置容量、逆变回馈装置启动电压、整流机组空载电压和车载制动电阻启动电压的约束条件，如式（7）.

$\left\{ \begin{array}{l} {x_{\min }} \leqslant {x_i} \leqslant {x_{\max }} ,\\ {U_{{\rm{min}}}} \leqslant {U_i} \leqslant {U_{{\rm{max}}}} ,\\ {U_{{\rm{d0,min}}}} \leqslant {U_{{\rm{d0}}}} \leqslant {U_{{\rm{d0,max}}}}, \\ {U_{{\rm{br,min}}}} \leqslant {U_{{\rm{br}}}} \leqslant {U_{{\rm{br,max}}}}, \\ \end{array} \right.$

(7)

式中：第i个牵引所的U_i为逆变回馈装置启动电压；U_d0为整流机组空载电压；U_br为车载制动电阻启动电压；x_max和x_min分别为投入逆变回馈装置容量的上、下限值；U_max和U_min分别为逆变回馈装置启动电压的上、下限值；U_d0,max和U_d0,min分别为整流机组空载电压的上、下限值；U_br,max和U_br,min分别为车载制动电阻启动电压的上、下限值.

2. 基于NSGA-Ⅱ求解逆变回馈装置定容选址优化算法

2.1 城轨牵引供电系统交直流混合潮流算法

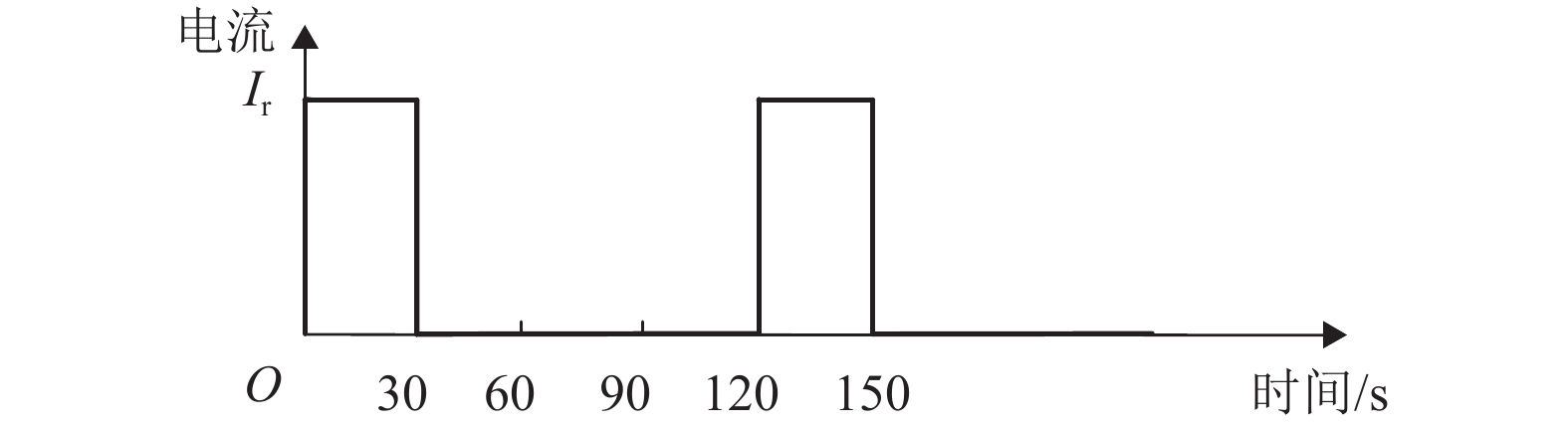
含逆变回馈装置的城轨交直流混合潮流计算以列车牵引计算信息和全日行车计划为计算条件，根据交直流供电系统拓扑结构建立直流侧节点电导矩阵和交流侧节点导纳矩阵，先进行直流侧潮流计算，收敛后更新牵引所运行状态，然后将直流侧电压参数收敛结果代入交流侧潮流计算，直至交流侧电压收敛. 其中，算法的可靠性以及控制策略改进分别在文献[14-15]中验证. 本文在上述算法基础上考虑了国标《城市轨道再生制动能量吸收逆变装置》（GB/T 37423—2019）规定的逆变回馈装置周期性间歇工作制^[13]，以120 s为工作周期，占空比为0.25，矩形工作制如图3所示. 图中：I_r为逆变回馈装置周期性间歇工作峰值电流.

图 3 逆变回馈装置矩形工作制

Figure 3. Rectangular work cycle of inverter feedback devices

下载: 全尺寸图片幻灯片

计算120 s内逆变回馈装置吸收电流的有效值，如式（8）.

${I_{\rm{RMS} }} = \sqrt {{{\displaystyle\sum\limits_{t = 1}^T {{I_{{t}}}^2} }}\bigg /{{120}}},$

(8)

式中：I_t为逆变回馈装置在时刻t吸收的直流电流；T为逆变回馈装置运行时间.

则120 s内逆变回馈装置的占空比σ计算如式（9）.

$\sigma = {\left( {\frac{{{I_{\rm{RMS} }}}}{{{I_{\rm{r}} }}}} \right)^2}.$

(9)

在交直流混合潮流算法中，根据式（9）对牵引所逆变回馈装置直流侧的电流进行限制，确保每120 s时间周期内逆变回馈装置工作占空比在0.25以内.

2.2 NSGA-Ⅱ算法优化逆变回馈装置定容选址

NSGA-Ⅱ算法首先根据个体之间的支配关系对N组种群进行非支配排序分层，经选择、交叉和变异获得第一代子种群；然后在传统NSGA算法基础上将父代种群与子代种群合并进行快速非支配排序，同时对每个非支配层中的个体进行拥挤距离计算，从而筛选出新的优质父代种群；最后逐次迭代直至达到迭代收敛条件或最大迭代次数，输出结果为Pareto最优解集.

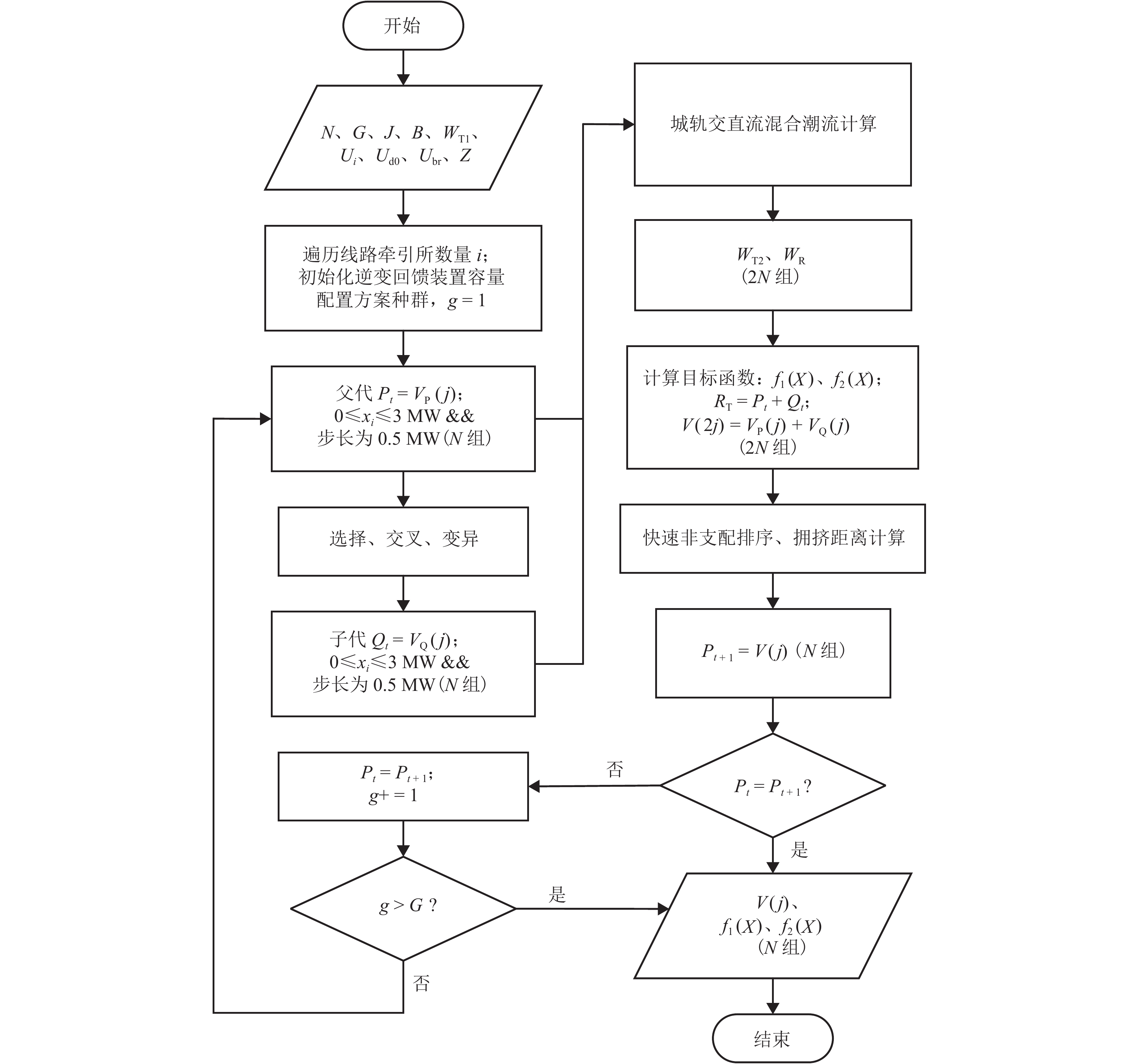
针对所提逆变回馈装置定容选址多目标优化问题，在城轨牵引供电仿真系统^[16]模拟列车运行工况、牵引计算、交直流混合潮流计算，得到牵引所整流机组输出能耗、逆变回馈装置反馈能量等牵引供电系统参数，进而求解目标函数. 采用交直流混合潮流算法与NSGA-Ⅱ结合求解流程如图4所示. 图中：g为迭代次数；V_P(j)和V_Q(j)分别为父代方案和子代方案.

图 4 基于NSGA-Ⅱ求解逆变回馈装置容量配置优化流程

Figure 4. Optimization process of siting and sizing for inverter feedback devices based on NSGA-Ⅱ algorithm

下载: 全尺寸图片幻灯片

图4中，初始父代种群是以0.5 MW为步长，随机产生的N组逆变回馈装置容量配置方案；G为最大迭代次数；J为交叉概率；B为变异概率；Z为整流机组额定功率；P_t和Q_t分别为时刻t的父代和子代容量配置.

3. 基于信息熵的TOPSIS法最优解选取

由于上述Pareto解集的每组解具有两个量纲属性，无法在N组Pareto解集中挑选出一组最优解. 为防止决策者偏好影响最优解选取，本文采用基于信息熵的TOPSIS将f₁(X)和f₂(X)无量纲属性归一化处理，在N组Pareto解集中筛选出与理想方案相对距离最小、与负理想方案相对距离最大的逆变回馈装置定容选址最优方案^[17]. 其中，通过信息熵赋予Pareto解集中各目标函数值的影响权重，权值大小反应了该目标函数值对最优解选取的影响程度^[18].

累和归一化与向量归一化方法均不会改变目标函数的原始值属性^[19]，考虑本文目标函数f₁(X)值为负数，因此采用累和归一化方法，如式（10）.

${f^\prime_{{m}}} (X) = \frac{{{f_{{m}}}(X)}}{{\displaystyle\sum\limits_{m = 1}^{{n}} {{f_{{m}}}(X)} }},$

(10)

式中：f_m(X)为方案V(j) 归一化处理前的第m个目标函数原始值；n为目标函数个数；$f_m' (X)$为归一化目标函数值.

子目标函数的信息熵计算如式（11）.

$E({{m}}) = {\rm{ - }}K\sum\limits_{j = 1}^{{N}} {\left[ {{f^\prime_{{m}}} (X) \ln {f^\prime_{{m}}} (X)} \right]},$

(11)

式中：E(m)为第m个子目标函数信息熵；熵系数K=1/ln m；当$f_m' (X)$为0时，令ln $f_m' (X) $为0.

子目标函数权重计算如式（12）.

${\varepsilon _{{m}}} = \frac{{1 - E(m)}}{{\displaystyle\sum\limits_{{{m}} = 1}^{{n}} {(1 - E(m))} }},$

(12)

式中：ε_m为第m个子目标函数的权重，0 < ε_m < 1，且ε₁+ε₂+···+ε_m=1.

根据$f_m' (X) $求解方案V(j)的信息熵以及权重得到V(j)的归一化相对理想距离d（j），如式（13）.

${{ d}}(j) = \frac{{{{{d}}_ + }(j)}}{{{{{d}}_ + }(j) + {{{d}}_ - }(j)}},$

(13)

式中：d₊(j)为优化方案V(j)到理想方案归一化相对距离；d₋(j)为优化方案V(j)到负理想方案归一化相对距离.

d(j)值越小则V(j)越接近理想方案.

4. 算例分析

4.1 仿真模型

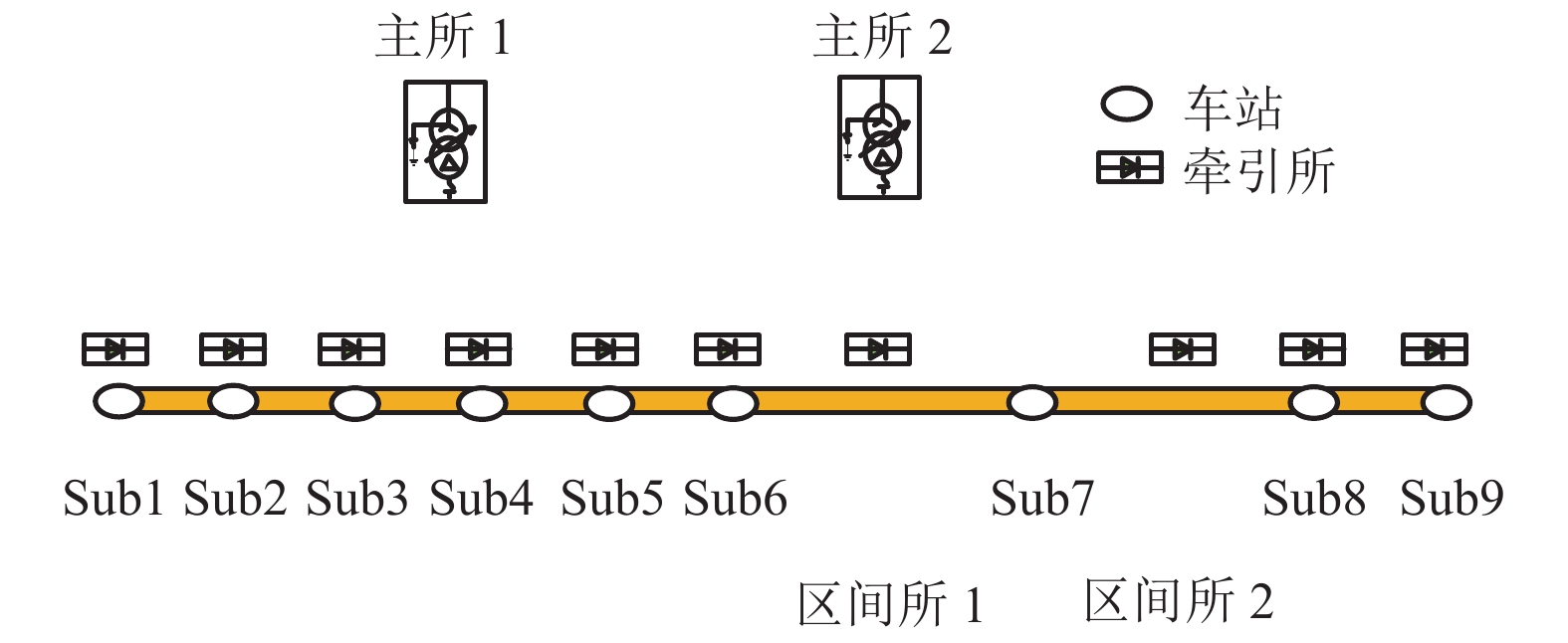
以广州某地铁线路一期工程进行算例仿真，该系统设有两座主变电所、10座牵引所，线路自西向东Sub1～Sub9共9个车站，全长26 km，除Sub7处为降压所外，其余车站均设有牵引所，另两座区间牵引所位置如图5，各牵引所位置信息如表1.

图 5 线路供电系统结构

Figure 5. Structure of line power supply system

下载: 全尺寸图片幻灯片

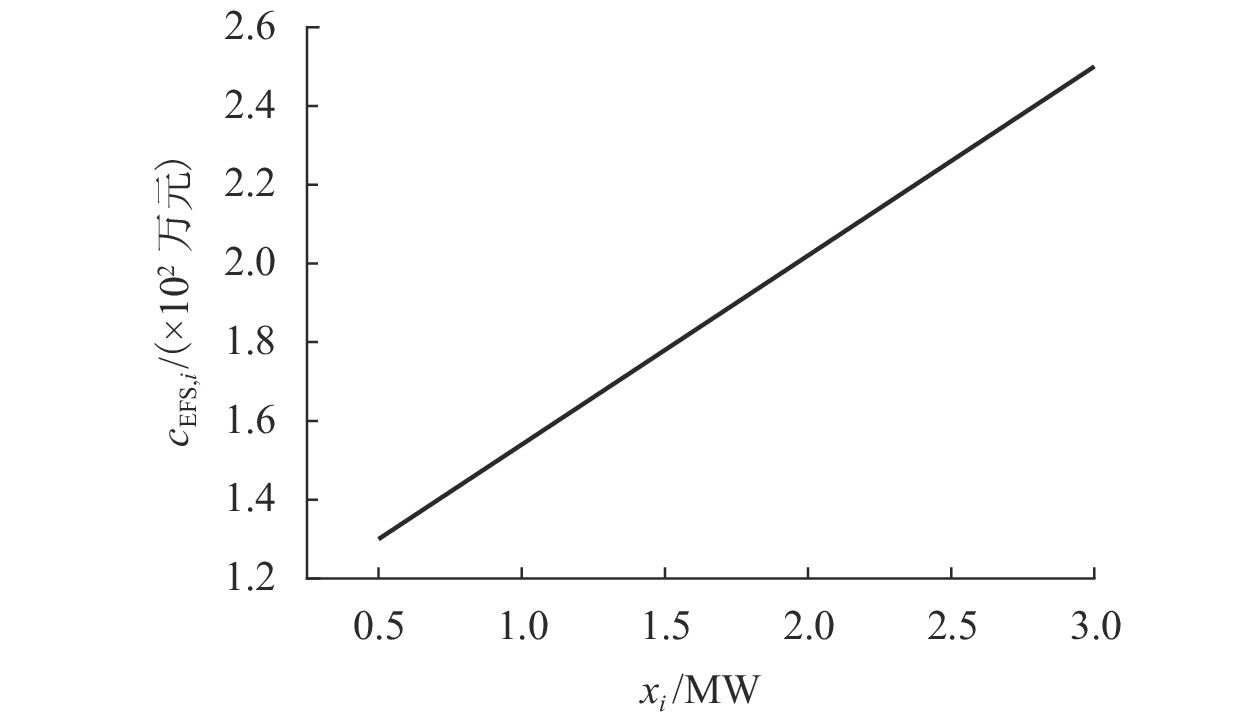
表 1 牵引所位置信息

Table 1. Traction station position information

牵引所	位置/km	牵引所	位置/km
Sub1	0.243	Sub6	13.900
Sub2	2.456	区间所 1	16.287
Sub3	4.568	区间所 2	20.527
Sub4	7.804	Sub8	23.322
Sub5	10.670	Sub9	25.685

下载: 导出CSV

| 显示表格

设计考虑列车初期行车计划：运营时间段为06:30～23:30，共17 h；期间发车间隔为525 s，共发车117对；列车车型为6B，4动2拖. 设置仿真参数如表2，逆变回馈装置容量与投资成本满足关系如图6所示.

表 2 仿真参数设置

Table 2. Simulation parameter setting

仿真参数	取值	仿真参数	取值
N	20	Z/MW	2×2.5
J	0.9	U_d0/V	1680
B	0.1	x_max/MW	3
G/次	100	U_i/V	1720
W_T1/（kW•h）	3954.17	U_br/V	1830

下载: 导出CSV

| 显示表格

图 6 逆变回馈装置价格与容量关系

Figure 6. Relationship between price and capacity of inverter feedback devices

下载: 全尺寸图片幻灯片

4.2 优化过程

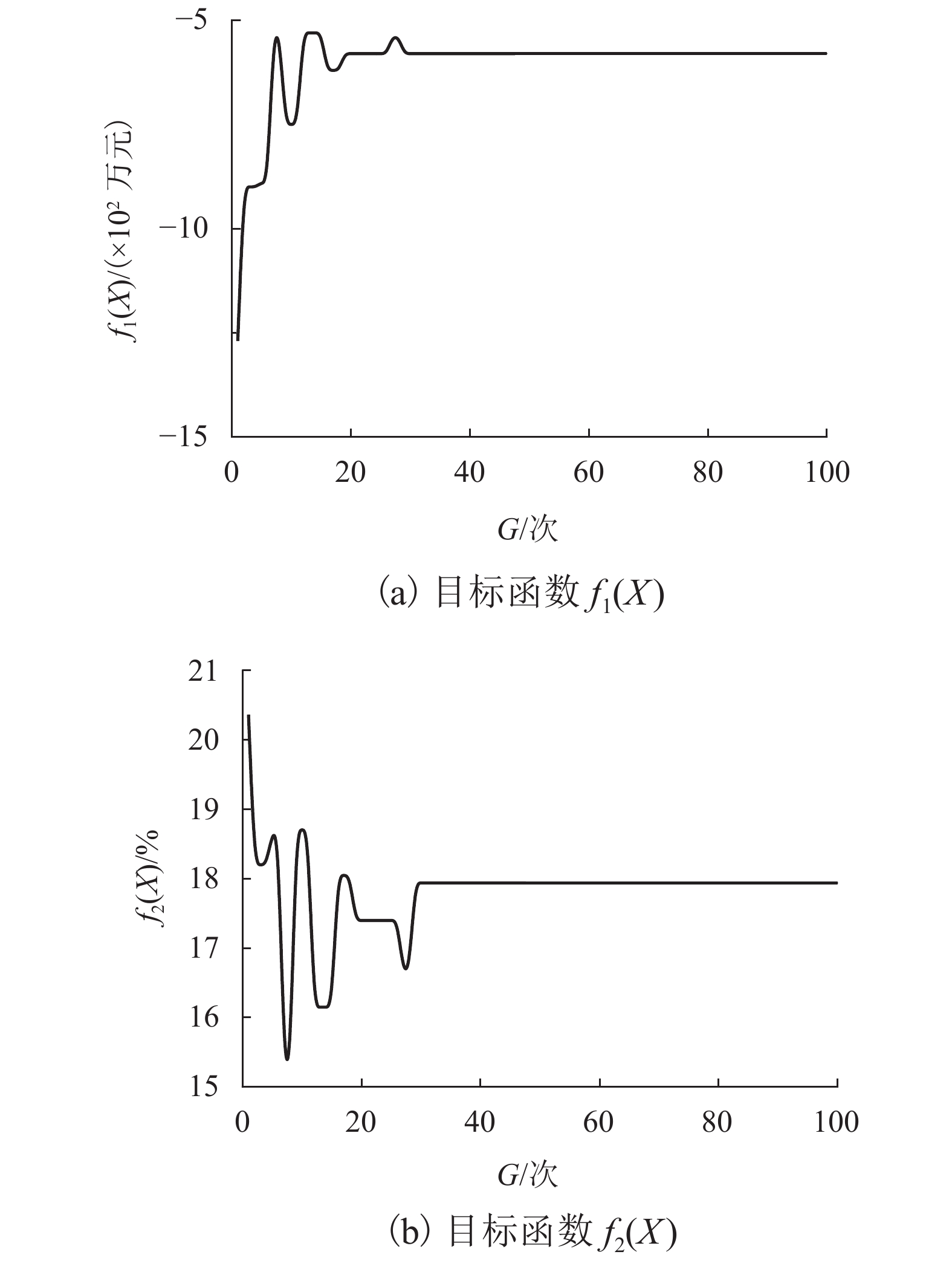
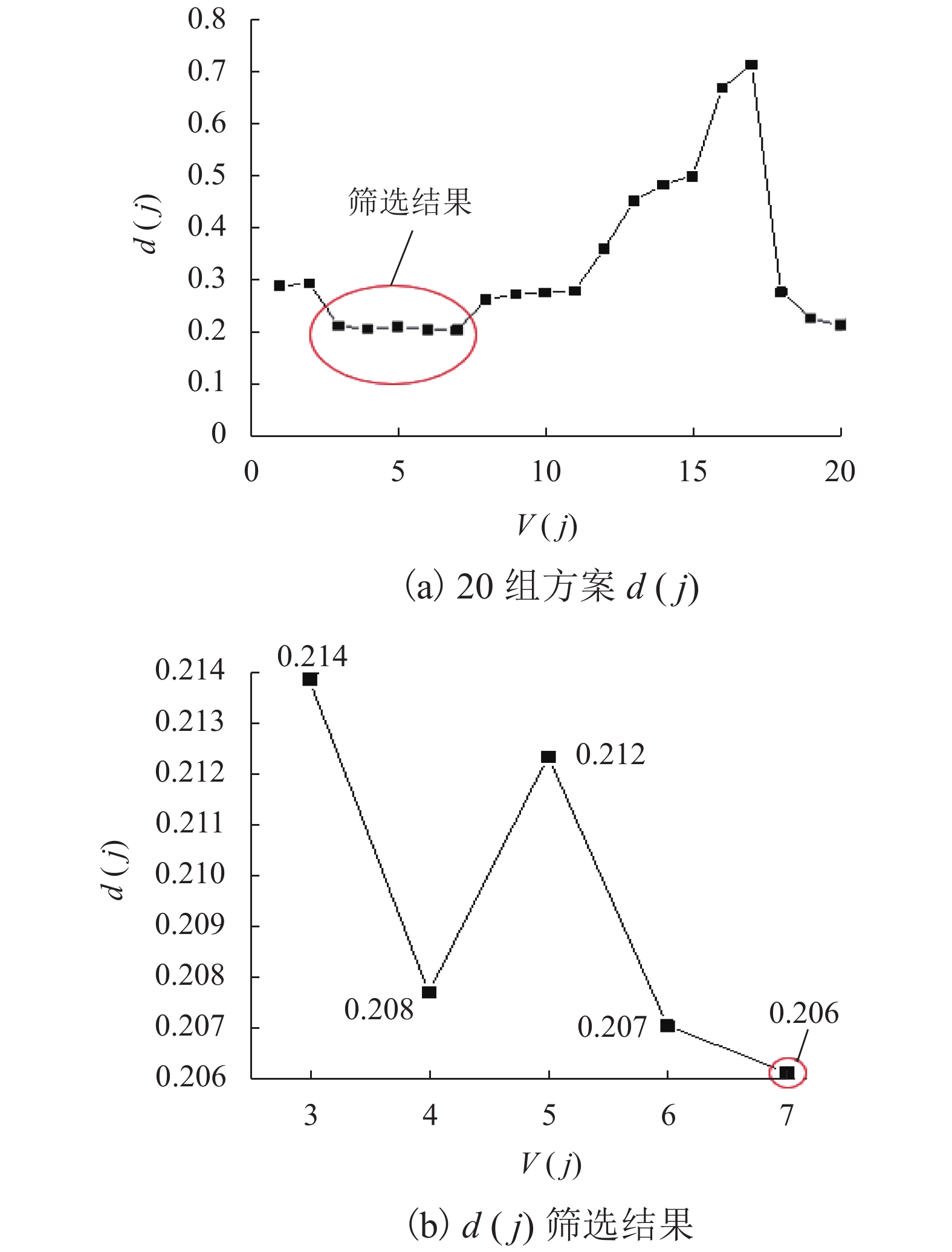
计算每次迭代Pareto解集的归一化相对理想距离并筛选出各次迭代的最优方案，其目标函数值收敛过程如图7，在迭代至30次左右时，最优方案的目标函数值基本收敛. 表3统计了收敛后目标函数的Pareto解集，图8（a）是Pareto收敛解集方案的归一化相对理想距离变化情况，图8（b）在方案3～7中筛选出d(j)最小的V(7)作为该线路逆变回馈装置定容选址的最优方案. 表4统计了V(7)的潮流计算结果，由式（5）可得系统级节能率为17.94%.

图 7 目标函数Pareto解集的最优方案变化过程

Figure 7. Change process of optimal scheme of Pareto solution set

下载: 全尺寸图片幻灯片

表 3 Pareto解集收敛结果

Table 3. Convergence results of Pareto solution set

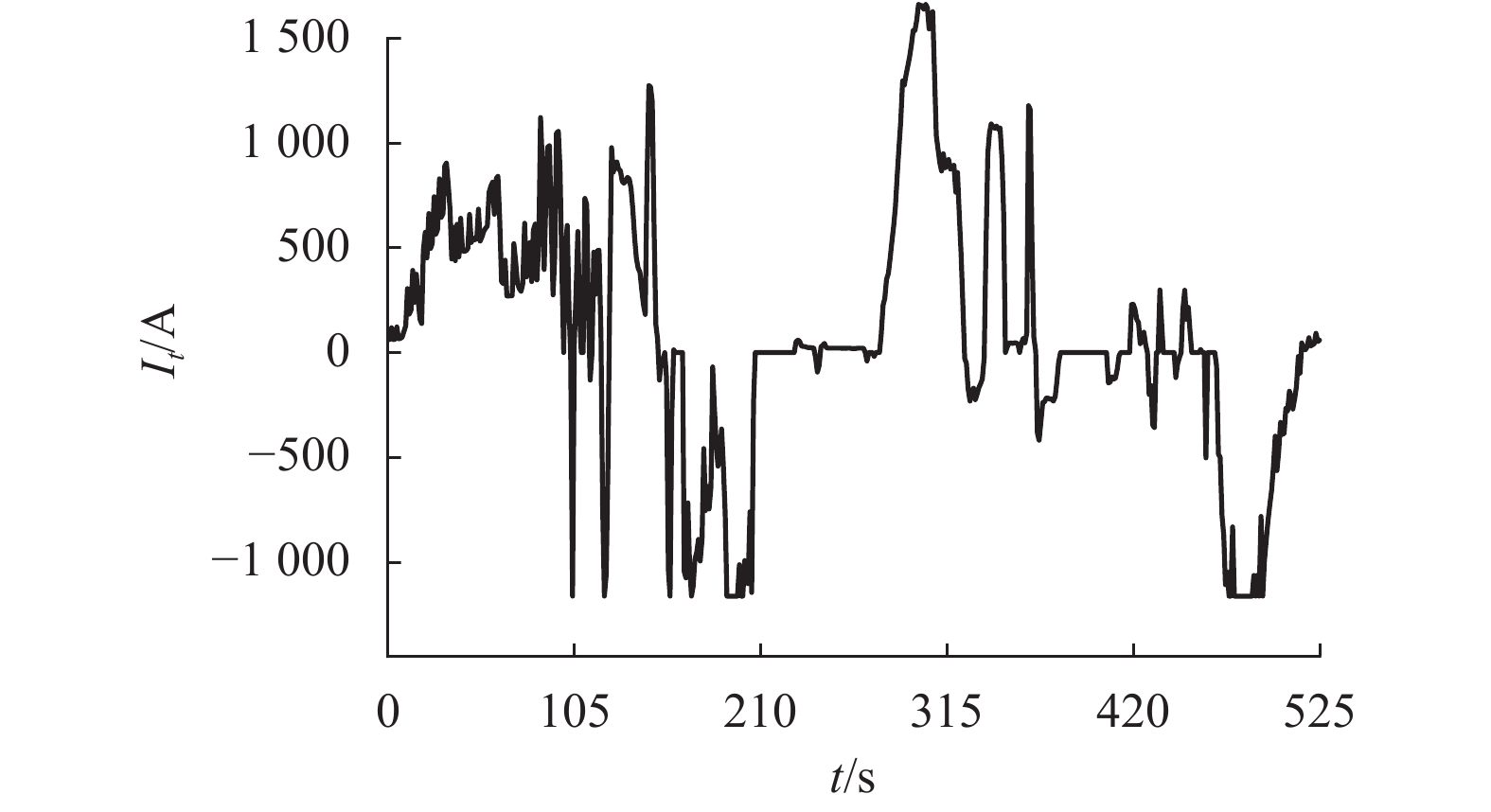
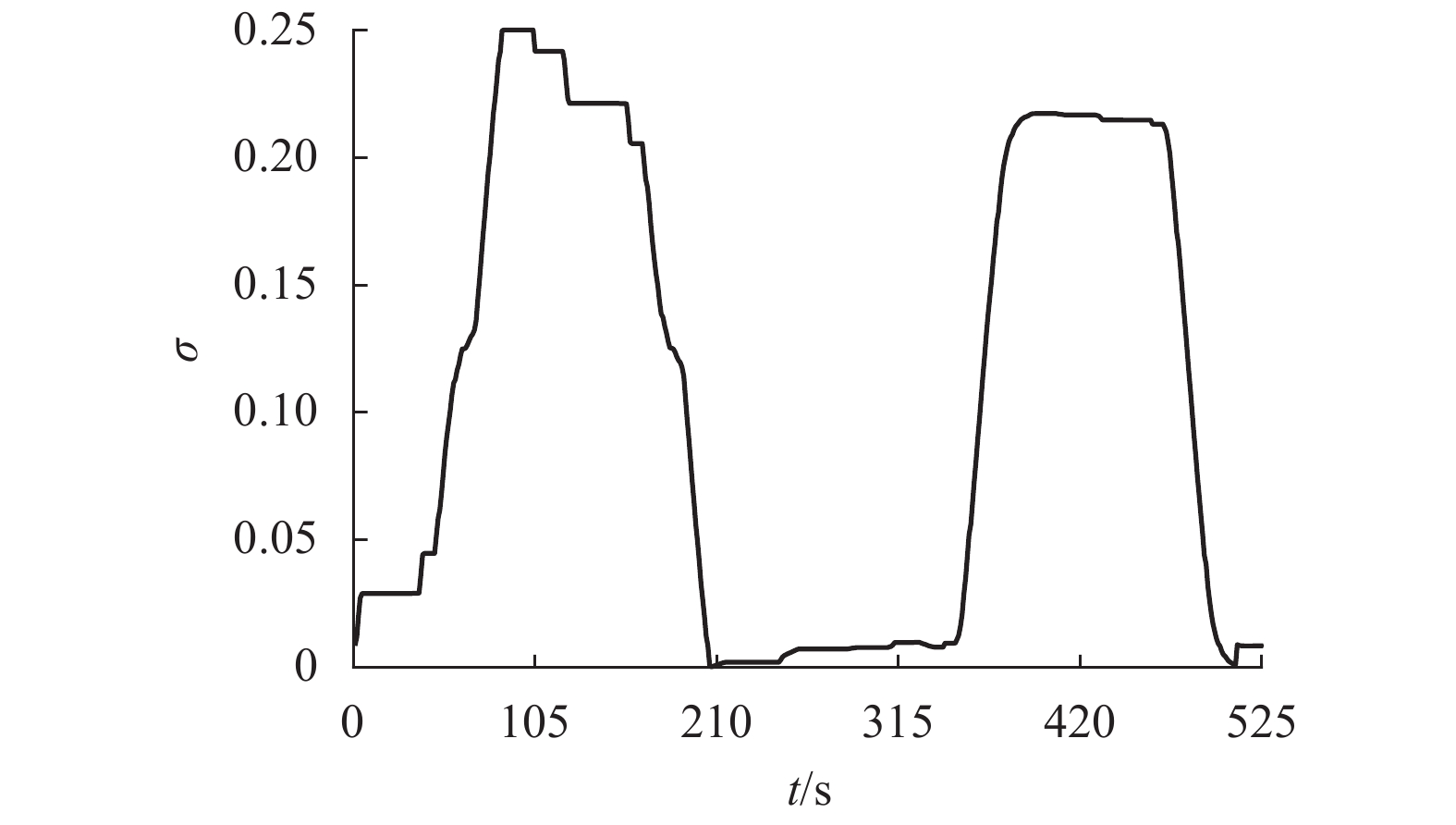
V(j)	f₁(X)/ （×10² 万元）	f₂(X)/%	V(j)	f₁(X)/ （×10² 万元）	f₂(X)/%
1	−3.30	9.54	11	−7.30	19.81
2	−3.60	9.55	12	−8.60	20.28
3	−4.00	14.03	13	−10.10	20.46
4	−4.20	14.53	14	−10.70	20.86
5	−5.30	16.15	15	−11.10	21.48
6	−5.40	16.66	16	−14.90	21.68
7	−5.80	17.94	17	−16.80	21.7
8	−6.70	17.79	18	−6.90	17.61
9	−6.90	17.95	19	−6.20	18.05
10	−7.10	18.85	20	−5.80	17.39

下载: 导出CSV

| 显示表格

为验证该优化方案的逆变回馈装置能满足周期性间歇工作制，以区间所2为例，统计牵引所直流电流如图9所示. 计算525 s发车间隔下该牵引所逆变回馈装置的工作占空比（σ），如图10所示.

图 9 区间所2直流侧电流

Figure 9. DC current of section traction substation 2

下载: 全尺寸图片幻灯片

图 10 区间所2逆变回馈装置工作占空比

Figure 10. Operating duty ratio of inverter feedback devices in section traction station 2

下载: 全尺寸图片幻灯片

4.3 优化方案对比

将筛选出的最优逆变回馈装置定容选址方案V(7)与该地铁工程实际安装逆变回馈装置方案V_a统计如表5，其目标函数值计算结果如表6，投资回报周期计算如式（14）.

表 5 逆变回馈装置方案对比

Table 5. Scheme comparison of inverter feedback devices MW

牵引所	V(7)，V_a	牵引所	V(7)，V_a
Sub1	0，2.0	Sub6	0，0
Sub2	2.0，0	区间所 1	0，0
Sub3	0，0	区间所 2	2.0，0
Sub4	1.5，0	Sub8	0，2.0
Sub5	0，0	Sub9	0，3.0

下载: 导出CSV

| 显示表格

表 6 不同优化方案目标函数值对比

Table 6. Comparison of objective function values of different optimization schemes

优化方案	f₁(X)/（×10²万元）	f₂(X)/%
V(7)	5.80	17.94
V_a	6.50	14.69

下载: 导出CSV

| 显示表格

图 8 归一化Pareto收敛解集

Figure 8. Normalized Pareto convergence solution set

下载: 全尺寸图片幻灯片

表 4 最优方案V(7)每小时潮流计算结果

Table 4. Hourly power flow calculated by optimal scheme V(7) kW•h

牵引所	W_T2	W_F	W_R
Sub1	275.97	0	45.66
Sub2	459.14	270.84
Sub3	499.08	0
Sub4	501.06	238.75
Sub5	473.03	0
Sub6	292.77	0
区间所 1	321.41	0
区间所 2	462.30	273.07
Sub8	416.51	0
Sub9	280.59	0
合计	3981.86	782.66	45.66

下载: 导出CSV

| 显示表格

$c = \frac{{ - {f_{\rm{1}}}(X)}}{{c_{\rm{m}}W D}} \times {10^6},$

(14)

式中：c为投资回报周期，a；c_m为地铁用电单价；D为线路全年运营天数；f₁(X)单位为10²万元.

从表6可以看出：本文所提逆变回馈装置优化方案V(7)对于该地铁工程实际配置方案V_a可以节省逆变回馈装置投资成本70万元，系统级节能率提高3.25%，按0.75元/（kW•h）电价计算，投资回报周期缩短236 d.

由于线路牵引所Sub7～Sub8站间距离长，列车制动频繁，优化算法搜索出在区间牵引所2装设逆变回馈装置的方案，且线路端头所处列车制动能量相对较少，安装逆变回馈装置节能效益不高，因此建议在该地铁线路Sub2和Sub4处的牵引所分别安装容量为2.0 MW和1.5 MW的逆变回馈装置，并在区间所2处安装容量为2.0 MW的逆变回馈装置.

5. 结　论

1）本文将NSGA-Ⅱ与城轨交直流混合潮流算法结合对广州某地铁线路逆变回馈装置定容选址多目标优化模型进行求解，迭代30次得到Pareto收敛解集. 采用基于信息熵的TOPSIS归一化目标函数值，通过比较d(j)筛选出在牵引所Sub2、Sub4和区间所2装设容量为2.0、1.5 MW和2.0 MW的逆变回馈装置优化方案.

2）将优化方案与该地铁工程实际逆变回馈装置配置方案进行对比，逆变回馈装置投资成本节省70万元，系统级节能率提高3.25%，投资回报周期相应缩短.

3）该算例采用B型列车按初期发车计划运行，发车间隔大，线路车辆稀疏，产生的制动能量较少，因此逆变回馈装置投入数量不需过多. 对于不同地铁线路，本文所述优化方法均能有效进行逆变回馈装置定容选址，提高再生制动能量利用率并缩短投资回报周期.

图 1 三相单级式光伏并网多逆变器系统结构

Figure 1. Structure diagram of three-phase single-stage photovoltaic grid-connected multi-inverter system

下载: 全尺寸图片幻灯片

图 2 系统控制框图

Figure 2. Block diagram of system control

下载: 全尺寸图片幻灯片

图 3 多逆变器戴维南等效模型

Figure 3. Thevenin equivalent model of multi-inverters

下载: 全尺寸图片幻灯片

图 4 模态分析法流程

Figure 4. Flow chart of modal analysis method

下载: 全尺寸图片幻灯片

图 5 多逆变器模态阻抗曲线

Figure 5. Multi-inverter modal impedance curve

下载: 全尺寸图片幻灯片

图 6 外界环境变化时模态分析结果

Figure 6. Modal analysis results when external environment changes

下载: 全尺寸图片幻灯片

图 7 输电距离变化时模态分析结果

Figure 7. Modal analysis results when transmission distance changes

下载: 全尺寸图片幻灯片

图 8 逆变器台数变化时节点电压频谱分析图

Figure 8. Spectrum analysis of node voltage when the number of inverters changes

下载: 全尺寸图片幻灯片

图 9 外界环境变化时节点电压频谱分析

Figure 9. Spectrum analysis of node voltage when external environment changes

下载: 全尺寸图片幻灯片

图 10 输电距离变化时节点电压频谱分析

Figure 10. Spectrum analysis of node voltage when transmission distance changes

下载: 全尺寸图片幻灯片

表 1 系统仿真参数

Table 1. Parameters of system simulation

参数	数值	参数	数值
光伏开路电压 U_oc/V	880	光伏 MPP 电压 U_m/V	700
光伏短路电流 I_sc/A	15.9	光伏 MPP 电流 I_m/A	14.7
电网电压 U_g/V	220	逆变器侧电感 L₁/mH	4
电网频率 f_g/Hz	50	网侧电感 L₂/mH	0.6
电网阻抗 L_g/mH	0.5	滤波电容 C/μF	10
开关频率 f_s/kHz	10	直流链电容 C_DC/μF	2 000
注：MPP—maximum power point.

下载: 导出CSV

表 2 逆变器台数变化时各节点参与因子

Table 2. Participation factor with variable inverter number

节点	n = 2		n = 4		n = 6
节点	f = 1 545 Hz	f = 2 230 Hz	f = 1 355 Hz	f = 2 230 Hz	f = 1 250 Hz	f = 2 230 Hz
1	0.082	0.063	0.032	0.026	0.017	0.014
2	0.418	0.437	0.218	0.224	0.149	0.152
3	0.500	0.500	0.565	0.083	0.833	0.040
4			0.190	0.569	0	0.091
5			0	0.098	0	0.415
6					0	0.046
7					0	0.325

下载: 导出CSV

表 3 不同输电距离下模态分析结果

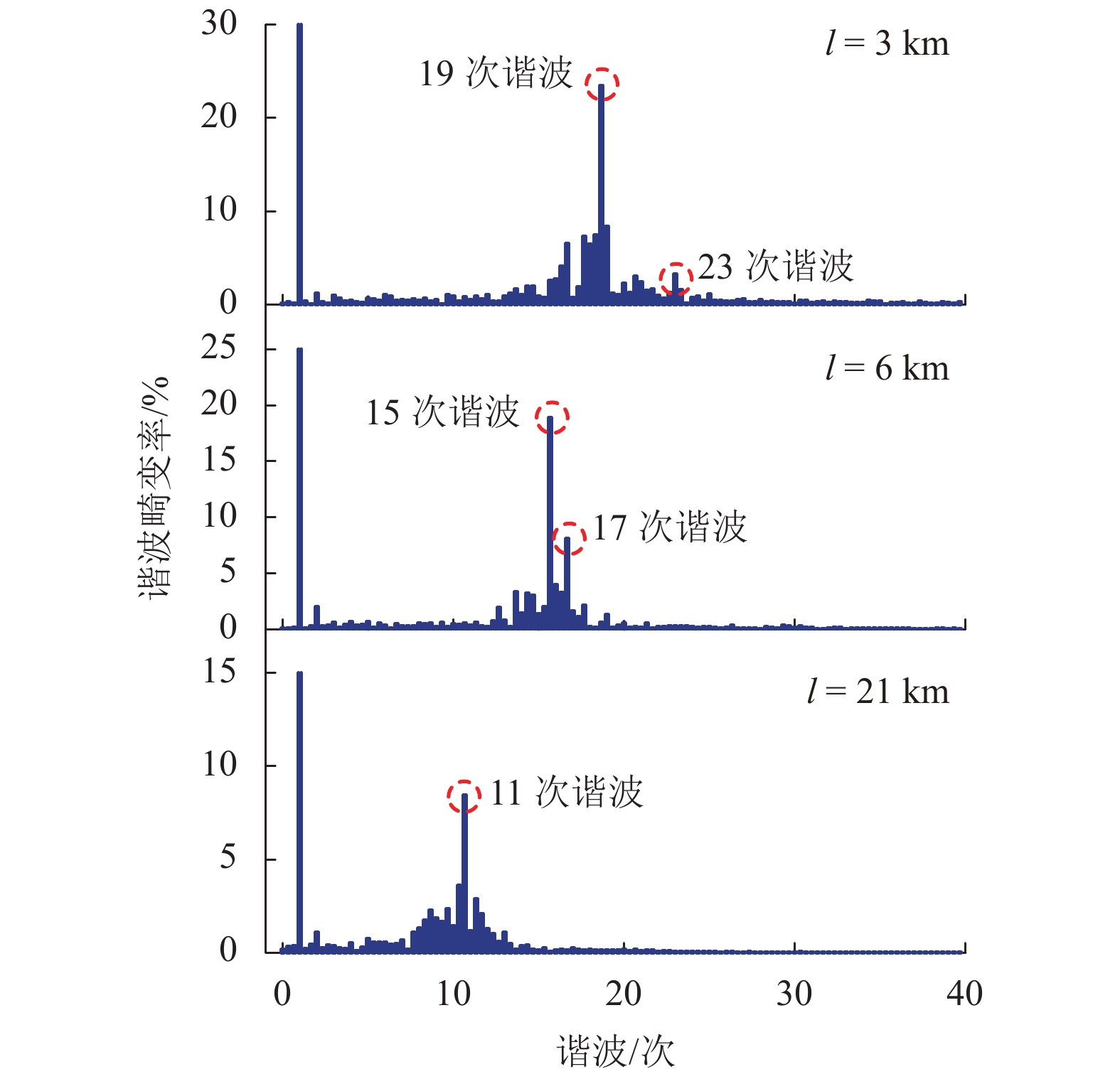
Table 3. Modal analysis results underdifferent transmission distances

l/km	f/Hz	模态阻抗/Ω
3	955	975.5
3	1 160	686.2
6	745	764.7
6	880	584.2
21	525	562.3
21	550	501.3

下载: 导出CSV

参考文献(17)

JALILI K, BERNET S. Design of LCL filters of active-front-end two level voltage-source converters[J]. IEEE Trans on Industrial Electronics, 2009, 56(5): 1674-1689. doi: 10.1109/TIE.2008.2011251

丁明,王伟胜,王秀丽,等. 大规模光伏发电对电力系统影响综述[J]. 中国电机工程学报,2014,34(1): 1-14.

DING Ming, WANG Weisheng, WANG Xiuli, et al. A review on the effect of large-scale PV generation on power systems[J]. Proceedings of the CSEE, 2014, 34(1): 1-14.

刘怀远,徐殿国,武健,等. 并网换流器系统谐振的分析、检测与消除[J]. 中国电机工程学报,2016,36(4): 1061-1074.

LIU Huaiyuan, XU Dianguo, WU Jian, et al. Analysis,detection and mitigation of resonance in grid-connected converter systems[J]. Proceedings of the CSEE, 2016, 36(4): 1061-1074.

张兴,余畅舟,刘芳,等. 光伏并网多逆变器并联建模及谐振分析[J]. 中国电机工程学报,2014,34(3): 336-345.

ZHANG Xing, YU Changzhou, LIU Fang, et al. Modeling and resonance analysis of multi-paralleled grid-tied inverters in PV systems[J]. Proceedings of the CSEE, 2014, 34(3): 336-345.

王振浩,孙玮澳,孙福军. 光伏系统并网的多逆变器并联交互影响分析[J]. 电力电子技术,2017,51(2): 29-32.

WANG Zhenhao, SUN Weiao, SUN Fujun. Interaction analysis of multiple paralleled inverters in photovoltaic system[J]. Power Electronics, 2017, 51(2): 29-32.

唐振东,杨洪耕,袁林. 弱电网下多逆变器并网控制通道间的交互影响分析[J]. 电网技术,2016,40(11): 3524-3531.

TANG Zhendong, YANG Honggeng, YUAN Lin. Analysis on interactive influences among control loops of multi inverters connected to weak-structured power system[J]. Power System Technology, 2016, 40(11): 3524-3531.

胡伟,孙建军,马谦,等. 多个并网逆变器间的交互影响分析[J]. 电网技术,2014,38(9): 2511-2518.

HU Wei, SUN Jianjun, MA Qian, et al. Analysis on interactive influences among multi grid-connected inverters[J]. Power System Technology, 2014, 38(9): 2511-2518.

HE J. Investigation and active damping of multiple resonances in a parallel-inverter-based microgrid[J]. IEEE Transactions on Power Electronics, 2013, 28(1): 234-246. doi: 10.1109/TPEL.2012.2195032

严干贵,常青云,黄亚峰,等. 弱电网接入下多光伏逆变器并联运行特性分析[J]. 电网技术,2014,38(4): 933-940.

YAN Gangui, CHANG Qingyun, HUANG Yafeng, et al. Analysis on parallel operational characteristics of multi photovoltaic inverters connected to weak-structured power system[J]. Power System Technology, 2014, 38(4): 933-940.

许德志,汪飞,毛华龙,等. 多并网逆变器与电网的谐波交互建模与分析[J]. 中国电机工程学报,2013,33(12): 64-71,187.

XU Dezhi, WANG Fei, MAO Hualong, et al. Modeling and analysis of harmonic interaction between multiple grid-connected inverters and the utility grid[J]. Proceedings of the CSEE, 2013, 33(12): 64-71,187.

孙振奥,杨子龙,王一波,等. 光伏并网逆变器集群的谐振原因及其抑制方法[J]. 中国电机工程学报,2015,35(2): 418-425.

SUN Zhenao, YANG Zilong, WANG Yibo, et al. The cause analysis and suppression method of resonances in clustered grid-connected photovoltaic inverters[J]. Proceedings of the CSEE, 2015, 35(2): 418-425.

XU W, HUANG Z Y, CUI Y, et al. Harmonic resonance mode analysis[J]. IEEE Transactions on Power Delivery, 2005, 20(2): 1182-1190. doi: 10.1109/TPWRD.2004.834856

仰彩霞, 刘开培, 李建奇, 等. 谐波谐振模态灵敏度分析[J]. 电工技术学报, 2011, 26（增刊1）: 207-212.

YANG Caixia, LIU Kaipei, LI Jianqi, et al. Modal sensitivity analysis for harmonic resonance[J]. Transac-tions of China Electrotechnical Society, 2011, 26（S1）: 207-212.

孙东,姚玉洁,刘玉林,等. 配电网谐波谐振改进模态分析方法的研究[J]. 电力电容器与无功补偿,2017,38(2): 105-110.

SUN Dong, YAO Yujie, LIU Yulin, et al. Study on improved mode analysis method of harmonic resonance in distribution grid[J]. Power Capacitor & Reactive Power Compensation, 2017, 38(2): 105-110.

艾欣,雷之力,崔明勇. 微电网谐波谐振的模态检测法研究[J]. 中国电机工程学报,2009,29(增刊1): 55-60.

AI Xin, LEI Zhili, CUI Mingyong. Study on modal survey method of the harmonic resonance in micro-grid[J]. Proceedings of the CSEE, 2009, 29(S1): 55-60.

谈萌,彭祥华,王同勋,等. 基于模态分析的半波长交流输电系统与风电场并网谐振研究[J]. 高电压技术,2018,44(1): 90-98.

TAN Meng, PENG Xianghua, WANG Tongxun, et al. Study on the harmonic resonance of grid-connected of wind farms and half-wavelength AC transmission system based on modal analysis method[J]. High Voltage Engineering,Electric Power Automation Equipment, 2018, 44(1): 90-98.

唐振东,杨洪耕. 基于模态分析的风电场并网谐波谐振研究[J]. 电力自动化设备,2017,37(3): 87-92,99.

TANG Zhendong, YANG Honggeng. Research on wind farm harmonic resonance based on modal analysis[J]. Electric Power Automation Equipment, 2017, 37(3): 87-92,99.

施引文献

附加材料(0)

访问统计