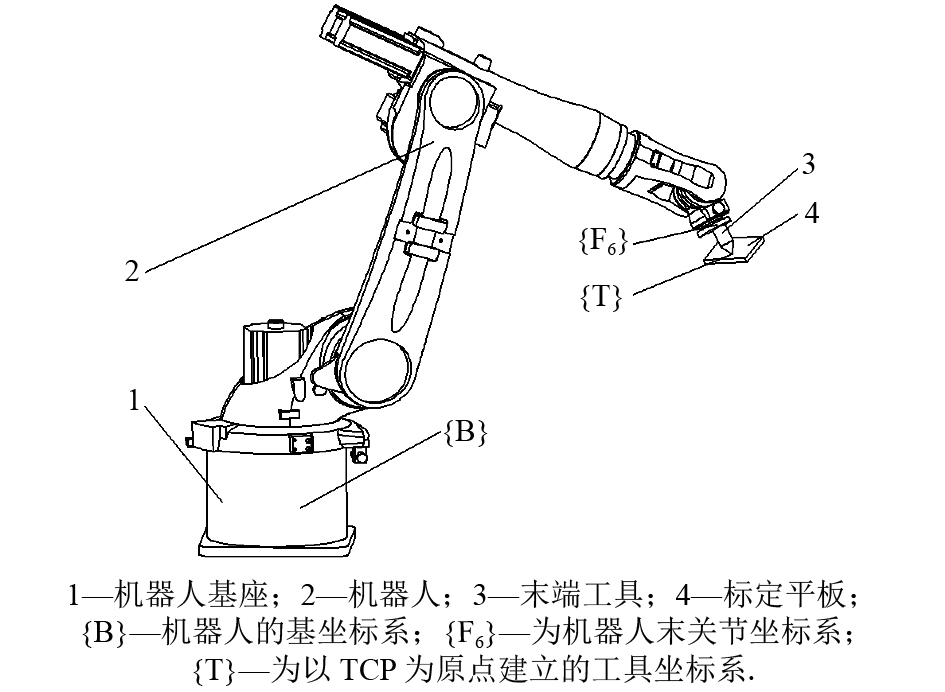
Method of Using Flat Plate as Calibration Tool for Robot Tool Center Point Calibration
-
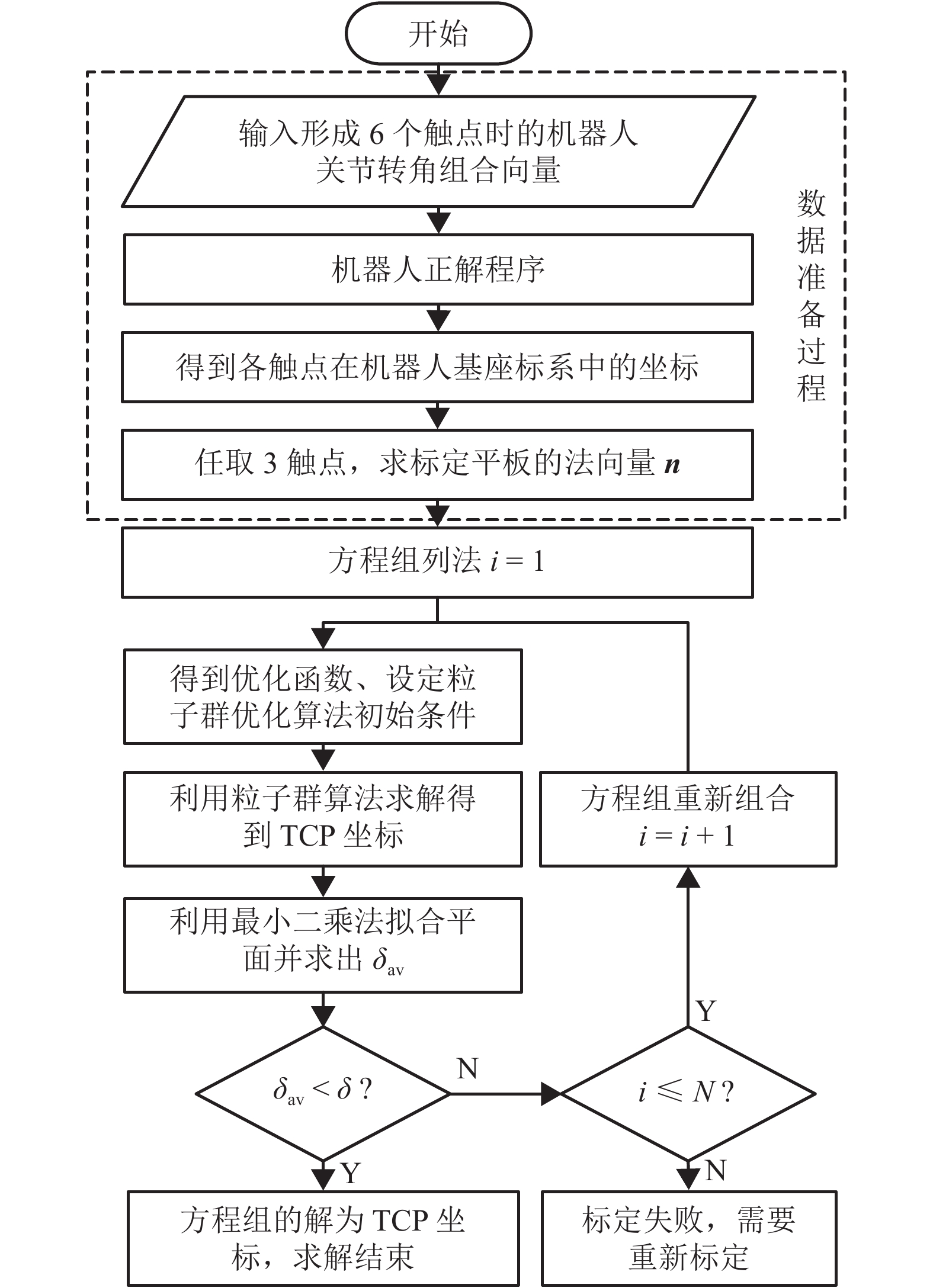
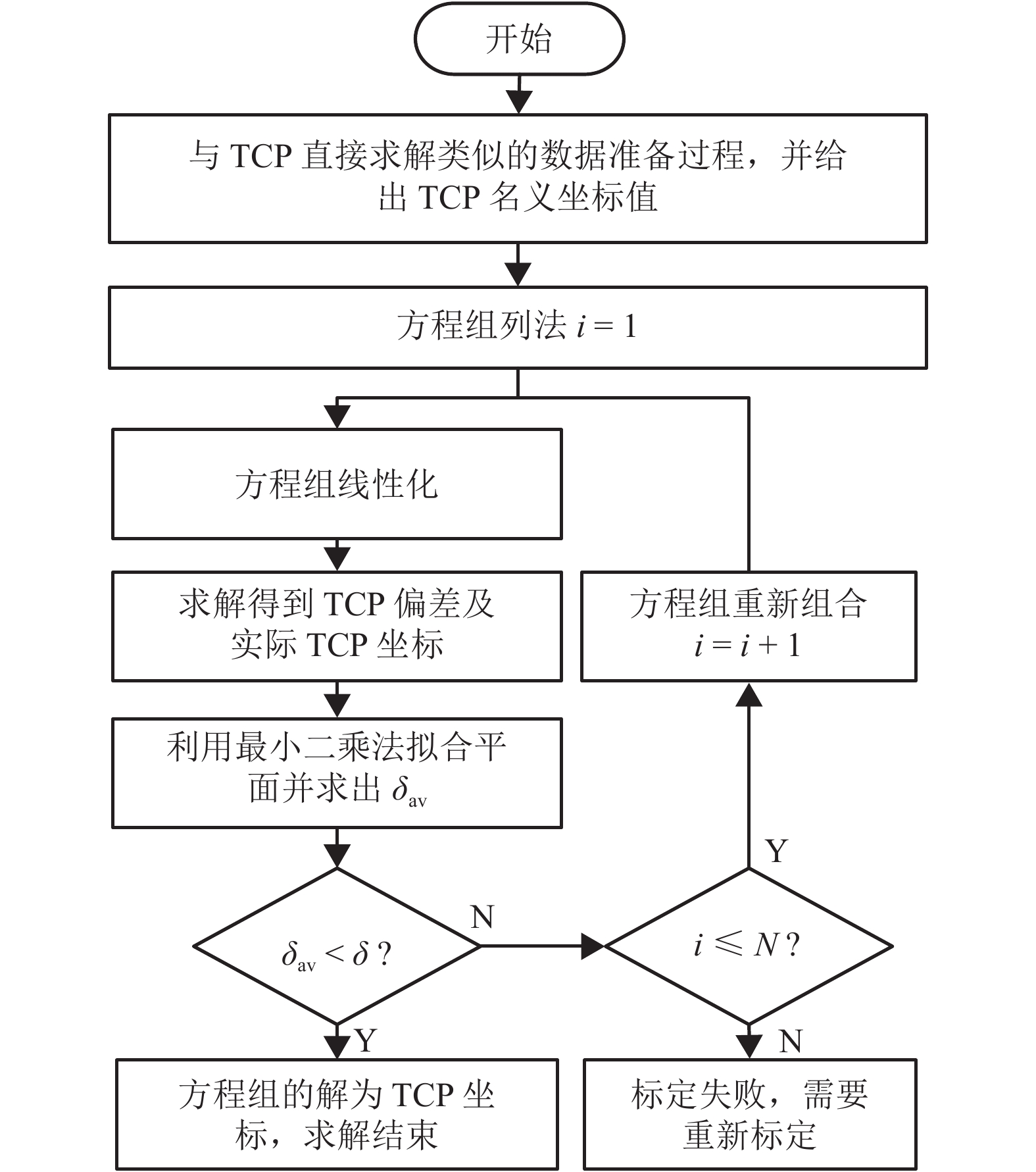
摘要: 为在工业现场便捷、准确地获取工具中心点（tool center point，TCP）参数，降低机器人末端工具的定位误差，提出了一种基于平板标定工具的机器人TCP标定方法. 利用机器人TCP与平板多次触碰所形成的空间触点应共面的约束条件，建立了机器人TCP参数标定模型；针对TCP名义参数未知和已知的情况，分别提出了基于粒子群算法的TCP直接求解和线性化的偏差求解算法. 通过数值仿真和标定试验验证所提出方法的可行性和准确性，结果表明：TCP直接求解和偏差求解算法求解的结果与传统四点标定法相比，误差分别在0.5 mm和1.0 mm以内.Abstract: To obtain the tool center point (TCP) parameters readily and accurately in the industrial field and reduce the positioning error of robot end tools, a robot TCP calibration method utilizing a flat plate as calibration tool is proposed. The robot TCP parameter calibration model is established under the constraint that the space contact points between the robot TCP and the flat should be coplanar. In the cases of unknown and known TCP nominal parameters, based on the particle swarm optimization (PSO) algorithm, the direct solution and linearization deviation solution algorithms for TCP are put forward respectively. The feasibility and accuracy of the proposed methods are verified by numerical simulation and calibration experiment. Compared with the traditional four-point calibration method, the error of TCP direct solution and deviation solution algorithm is less than 0.5 mm and 1.0 mm, respectively. The calibration method proposed is simple and easy to implement, and possesses high calibration efficiency and precision.
-
Key words:
- robot /
- TCP calibration /
- flat plate calibration /
- calibration algorithm
-
图 6 采用直接求解法得到的触点距各自拟合平面距离
Figure 6. Distance between contact points obtained by direct solution algorithm and fitting plane
图 7 采用TCP偏差算法计算的触点距各自拟合平面距离
Figure 7. Distance between contact points obtained by TCP deviation solution algorithm and fitting plane
表 1 TCP与标定平板上的6个点重合时机器人各关节转角
Table 1. Rotation angles of each robot joint when TCP coincides with the six points on flat plate
(°) $j$ $\theta _1^{(j)}$ $\theta _2^{(j)}$ $\theta _3^{(j)}$ $\theta _4^{(j)}$ $\theta _5^{(j)}$ $\theta _6^{\left( j \right)}$ 1 − − 4.592 3.883 40.894 93.418 2 −16.360 −69.026 3.156 3.766 39.137 133.812 3 −18.966 −72.777 7.720 15.494 51.803 170.749 4 −15.545 −72.356 10.098 30.897 27.092 129.078 5 −17.059 −76.277 13.533 30.244 33.174 28.440 6 −16.637 −72.496 8.437 33.510 43.938 50.159 
下载: 导出CSV
表 2 KUKA-KR5 arc六轴机械手D-H参数
Table 2. D-H parameters of KUKA-KR5 arc six-axis manipulator
i 连杆扭角
${\alpha _{i - 1}}$/（°）连杆长度
${{\rm{\alpha }}_{i - 1}}$/mm连杆偏距
${d_i}$/mm关节变量
${\theta _i}$/（°）关节变量
范围/（°）1 −180 0 −400 ${\theta _1}$ $ \pm 155$ 2 90 180 0 ${\theta _2}$ 65 ～ −180 3 0 600 0 ${\theta _3} - 90$ 158 ～ −15 4 90 40 −620 ${\theta _4}$ $ \pm 350$ 5 −90 0 0 ${\theta _5}$ $ \pm 130$ 6 90 0 −80 ${\theta _6}$ $ \pm 350$
下载: 导出CSV
表 3 带尖端的工具TCP与标定平板触碰10次机器人各关节转角
Table 3. Rotation angles of each robot joint when TCP of pointed tool touches flat plate for ten times
(°) $j$ $\theta _1^{(j)}$ $\theta _2^{(j)}$ $\theta _3^{(j)}$ $\theta _4^{(j)}$ $\theta _5^{(j)}$ $\theta _6^{\left( j \right)}$ 1 2.620 −97.869 106.089 −42.201 53.464 53.897 2 3.405 0 −98.277 109.043 −50.821 53.495 67.413 3 −8.362 −99.114 109.047 −42.222 43.170 54.233 4 −2.599 −95.747 107.063 −46.442 44.167 49.021 5 −19.339 −93.316 98.347 2.277 44.676 −6.206 6 −4.734 −81.491 80.254 −7.669 72.043 5.501 7 −16.592 −94.005 100.254 12.081 41.775 10.819 8 −15.702 −94.978 109.910 −13.487 16.745 10.065 9 −8.751 −87.764 92.296 −2.780 48.022 −17.819 10 −10.505 −86.340 87.267 5.413 60.768 −18.097
下载: 导出CSV
表 4 随机选取的五组点
Table 4. Randomly selected five sets of points
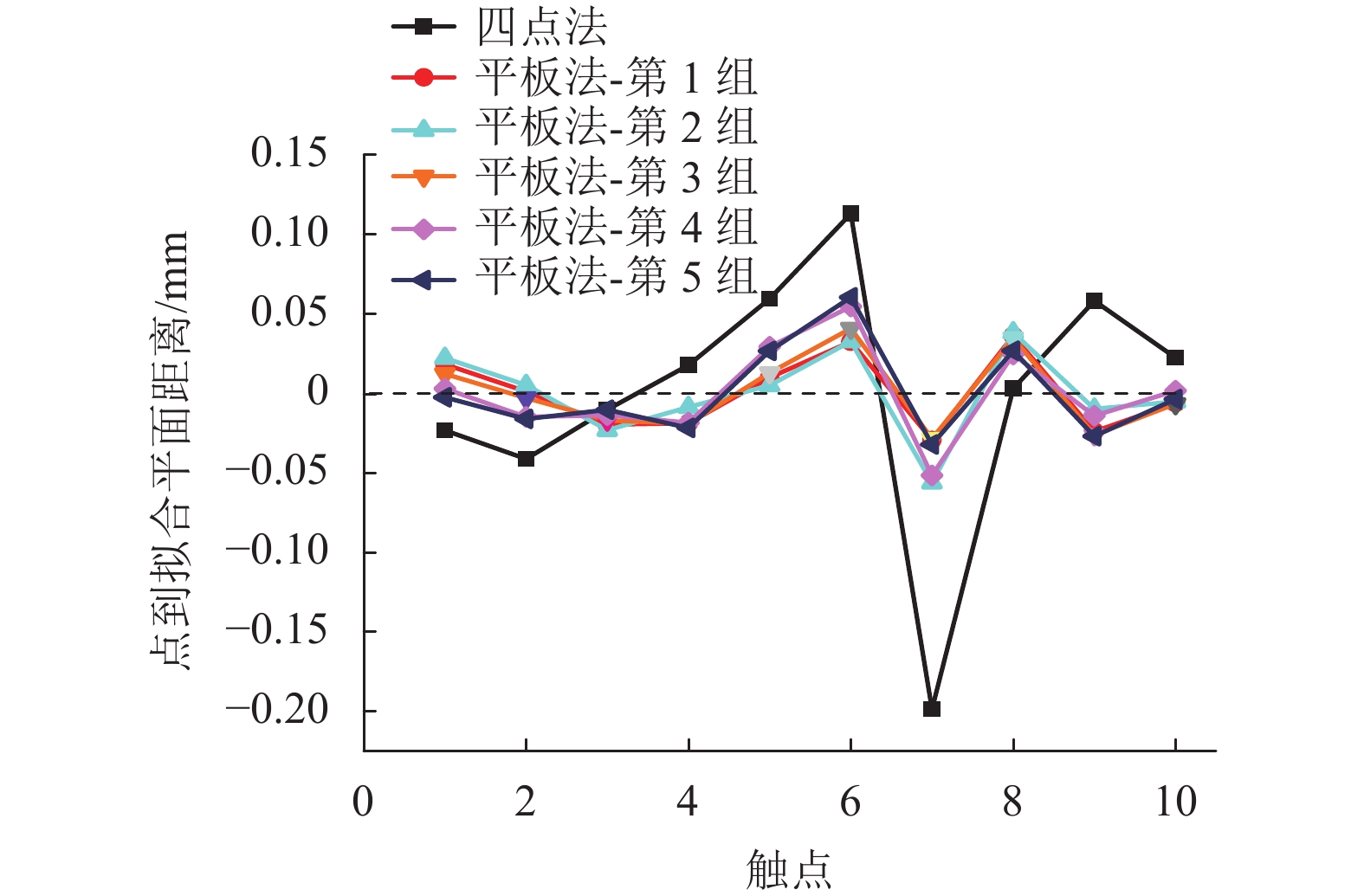
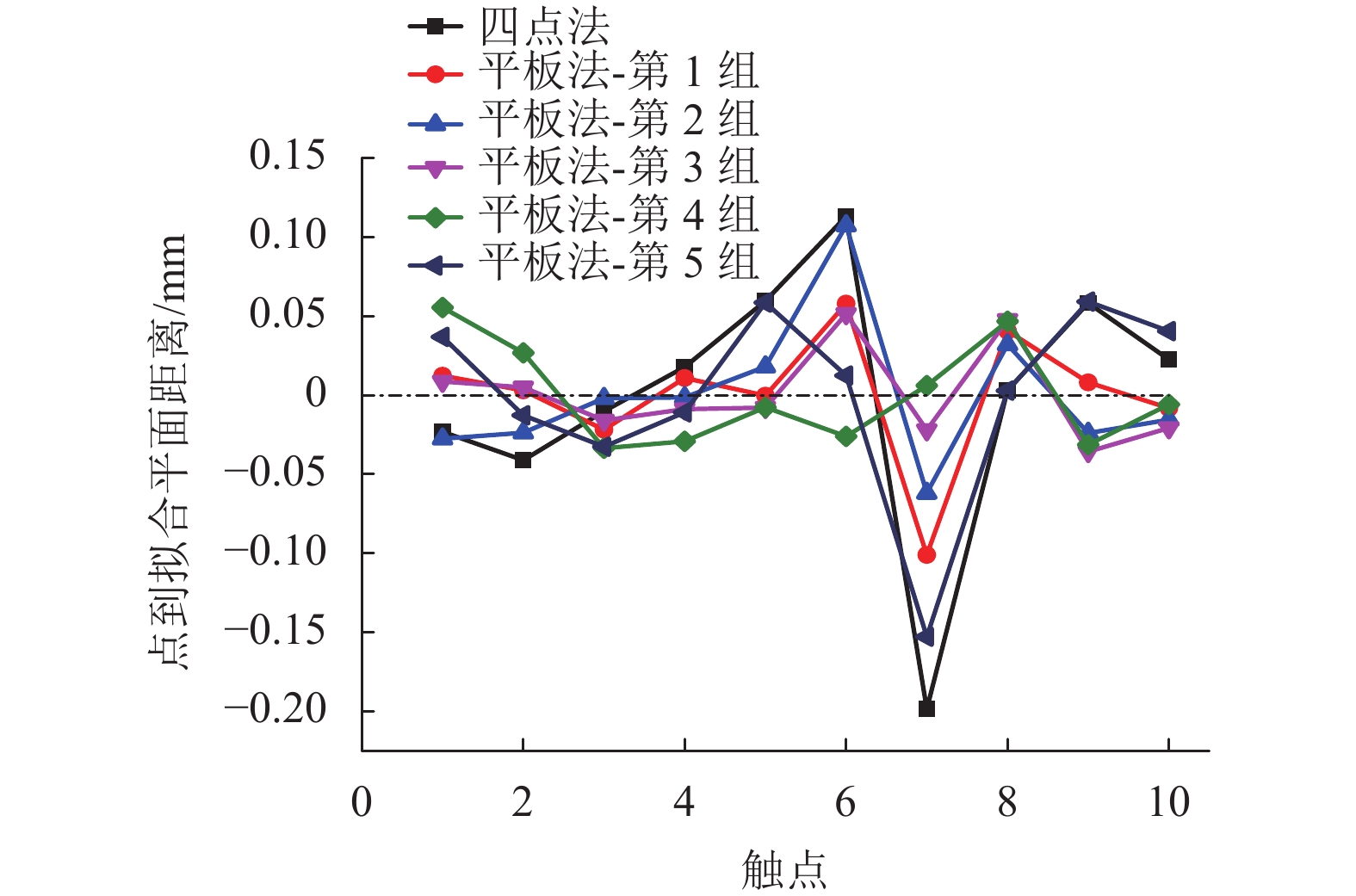
组数 点的组合 第1组 1，4，5，7，9，10 第2组 1，2，4，6，8，9 第3组 2，4，5，7，9，10 第4组 3，5，6，7，8，10 第5组 2，3，4，6，8，9
下载: 导出CSV
表 5 采用TCP直接求解算法计算的TCP数值及触点距拟合平面距离参数
Table 5. TCP value calculated by the TCP direct solution algorithm and distance from contact points to fitting plane
mm 算法 6PTCP ${\delta _{{\rm{av}}}}$ ${\delta _{{\rm{max}}}}$ ${\delta _{{\rm{min}}}}$ xyz 四点法 (77.051，17.549，424.403) 0.054 700 44 0.198 660 20 0.002 9713 34 平板法 第1组 (77.261，17.963，424.182) 0.019 577 00 0.036 198 00 0.001 1571 61 第2组 (77.302，17.892，424.212) 0.020 623 99 0.056 222 38 0.004 9632 50 第3组 (77.164，17.965，424.105) 0.020 089 88 0.040 741 77 0.002 9905 96 第4组 (77.174，17.916，424.232) 0.022 560 21 0.054 562 15 0.001 6164 12 第5组 (77.009，17.960，424.041) 0.022 746 09 0.060 353 03 0.002 5718 00
下载: 导出CSV
表 6 采用TCP偏差求解算法计算的TCP数值及触点距拟合平面距离参数
Table 6. TCP value calculated by TCP deviation solution algorithm and distance from contact points to fitting plane
mm 分组 d 6PTCP r ${\delta _{{\rm{av}}}}$/
× 10−2${\delta _{{\rm{max}}}}$/
× 10−2${\delta _{{\rm{min}}}}$/
× 10−21 ${\left[ {\begin{array}{*{20}{c}} {{\rm{ - 0}}{\rm{.961}}} \\ {{\rm{ - 0}}{\rm{.236}}} \\ {{\rm{ - 1}}{\rm{.043}}} \end{array}} \right]}$ ${\left[ {\begin{array}{*{20}{c}} {{\rm{ 77}}{\rm{.039}}} \\ {{\rm{17}}{\rm{.764}}} \\ {{\rm{423}}{\rm{.957}}} \end{array}} \right]}$ 2.638 10.116 0.046 2 ${\left[ {\begin{array}{*{20}{c}} {{\rm{ - 1}}{\rm{.639}}} \\ {{\rm{ - 0}}{\rm{.141}}} \\ {{\rm{ - 1}}{\rm{.617}}} \end{array}} \right]}$ ${\left[ {\begin{array}{*{20}{c}} {{\rm{76}}{\rm{.361}}} \\ {{\rm{17}}{\rm{.860}}} \\ {{\rm{423}}{\rm{.383}}} \end{array}} \right]}$ 3.140 10.719 0.157 3 ${\left[ {\begin{array}{*{20}{c}} {{\rm{ - 1}}{\rm{.179}}} \\ {{\rm{ - 0}}{\rm{.041}}} \\ {{\rm{ - 1}}{\rm{.357}}} \end{array}} \right]}$ ${\left[ {\begin{array}{*{20}{c}} {{\rm{76}}{\rm{.821}}} \\ {{\rm{17}}{\rm{.959}}} \\ {{\rm{423}}{\rm{.643}}} \end{array}} \right]}$ 2.242 5.130 0.479 4 ${\left[ {\begin{array}{*{20}{c}} {{\rm{ - 0}}{\rm{.160}}} \\ {{\rm{0}}{\rm{.060}}} \\ {{\rm{ - 0}}{\rm{.390}}} \end{array}} \right]}$ ${\left[ {\begin{array}{*{20}{c}} {{\rm{77}}{\rm{.840}}} \\ {{\rm{18}}{\rm{.059}}} \\ {{\rm{424}}{\rm{.609}}} \end{array}} \right]}$ 2.689 5.529 0.592 5 ${\left[ {\begin{array}{*{20}{c}} {{\rm{0}}{\rm{.341}}} \\ {{\rm{ - 0}}{\rm{.299}}} \\ {{\rm{0}}{\rm{.612}}} \end{array}} \right]}$ ${\left[ {\begin{array}{*{20}{c}} {{\rm{78}}{\rm{.341}}} \\ {{\rm{17}}{\rm{.701}}} \\ {{\rm{425}}{\rm{.612}}} \end{array}} \right]}$ 4.189 5.287 0.272
下载: 导出CSV
-
韩奉林,江晓磊,严宏志. 基于可测距平板工具的机器人TCP标定方法[J]. 计算机工程与应用,2016,52(18): 18-23. doi: 10.3778/j.issn.1002-8331.1512-0302HAN Fenglin, JIANG Xiaolei, YAN Hongzhi. Robotic TCP calibration method based on plane toolbar with distance measure function[J]. Computer Engineering and Applications, 2016, 52(18): 18-23. doi: 10.3778/j.issn.1002-8331.1512-0302 康存锋,王红伟,张鹏飞,等. 焊接机器人工具坐标系标定的研究与实现[J]. 北京工业大学学报,2016,42(1): 30-34.KANG Cunfeng, WANG Hongwei, ZHANG Pengfei, et al. Study and realization of tool coordinate frame calibration for welding robots[J]. Journal of Beijing University of Technology, 2016, 42(1): 30-34. CHENG F S. The method of recovering robot TCP positions in industrial robot application programs[C]//International Conference on Mechatronics and Automation. Harbin: [s.n.], 2007: 805-811. 李瑞峰,候琳琪,陶谦. 机器人末端工具参数自动标定方法[J]. 哈尔滨工业大学学报,1998,30(3): 74-76.LI Ruifeng, HOU Linqi, TAO Qian. Automatic calibration method for end-tool parameters of robot[J]. Journal of Harbin Institute of Technology, 1998, 30(3): 74-76. 宋月娥,吴林,田劲松,等. 弧焊机器人工具参数标定[J]. 焊接学报,2001,22(5): 1-4. doi: 10.3321/j.issn:0253-360X.2001.05.001SONG Yuee, WU Lin, TIAN Jingsong, et al. Cibration of tool parameters for arc welding robot[J]. Transactions of the China Welding Institution, 2001, 22(5): 1-4. doi: 10.3321/j.issn:0253-360X.2001.05.001 牛雪娟,刘景泰. 基于奇异值分解的机器人工具坐标系标定[J]. 自动化与仪表,2008(3): 1-4. doi: 10.3969/j.issn.1001-9944.2008.03.001NIU Xuejuan, LIU Jingtai. Robot tool control frame calibration based on SVD[J]. Automation & Instrumentation, 2008(3): 1-4. doi: 10.3969/j.issn.1001-9944.2008.03.001 刘成业,李文广,马世国,等. 一种机器人工具坐标系标定方法[J]. 山东科学,2012,25(1): 69-74. doi: 10.3976/j.issn.1002-4026.2012.01.015LIU Chengye, LI Wenguang, MA Shiguo, et al. A robot tool frame calibration method[J]. Shandong Science, 2012, 25(1): 69-74. doi: 10.3976/j.issn.1002-4026.2012.01.015 LI X, FUHLBRIGGE T A, SANG C, et al. Automatic offline program calibration in robotic cells[C]//IEEE, International Conference on Cyber Technology in Automation, Control, and Intelligent Systems. Hong Kong: IEEE, 2014: 585-590. HALLENBERG J. Robot tool center point calibration using computer vision[D]. Linköping: Linköping University, 2007. 刘常杰,班荣兴,郭寅,等. 基于双目视觉机器人TCP校准方法研究[J]. 红外与激光工程,2015,44(6): 1912-1917. doi: 10.3969/j.issn.1007-2276.2015.06.042LIU Changjie, BAN Rongxing, GUO Yin, et al. Calibration method of TCP based on stereo vision robot[J]. Infrared & Laser Engineering, 2015, 44(6): 1912-1917. doi: 10.3969/j.issn.1007-2276.2015.06.042 杜姗姗,周祥. 基于相机空间点约束的机器人工具标定方法[J]. 计算机应用,2015,35(9): 2678-2681. doi: 10.11772/j.issn.1001-9081.2015.09.2678DU Shanshan, ZHOU Xiang. Robot tool calibration method based on camera space point constraint[J]. Journal of Computer Applications, 2015, 35(9): 2678-2681. doi: 10.11772/j.issn.1001-9081.2015.09.2678 RUTHER M, LENZ M, BISCHOF H. The narcissistic robot: robot calibration using a mirror[C]//11th International Conference on Control Automation Roboticsand Vision. Singapore: IEEE, 2010: 169-174. SOUSA G B D, OLABI A, PALOS J, et al. 3D metrology using a collaborative robot with a laser triangulation sensor[J]. Procedia Manufacturing, 2017, 11: 132-140. doi: 10.1016/j.promfg.2017.07.211 CRAIG J J. Introduction to robotics mechanics and control[M]. Upper Saddle River: Pearson Education International, 2005: 48-70. 徐鹤鸣. 多目标粒子群优化算法的研究[D]. 上海: 上海交通大学, 2013. 刘志刚,曾嘉俊,韩志伟. 基于个体最优位置的自适应变异扰动粒子群算法[J]. 西南交通大学学报,2012,47(5): 761-768. doi: 10.3969/j.issn.0258-2724.2012.05.006LIU Zhigang, ZENG Jiajun, HAN Zhiwei. Adaptive mutation disturbance particle swarm optimization algorithm based on personal best position[J]. Journal of Southwest Jiaotong University, 2012, 47(5): 761-768. doi: 10.3969/j.issn.0258-2724.2012.05.006 -
下载:
点击查看大图
图(7) / 表(6)
计量
- 文章访问数: 639
- HTML全文浏览量: 257
- PDF下载量: 36
- 被引次数: 0