Structural Nonlinear Damage Identification Based on Autoregressive Conditional Heteroskedasticity Conversion Index
-
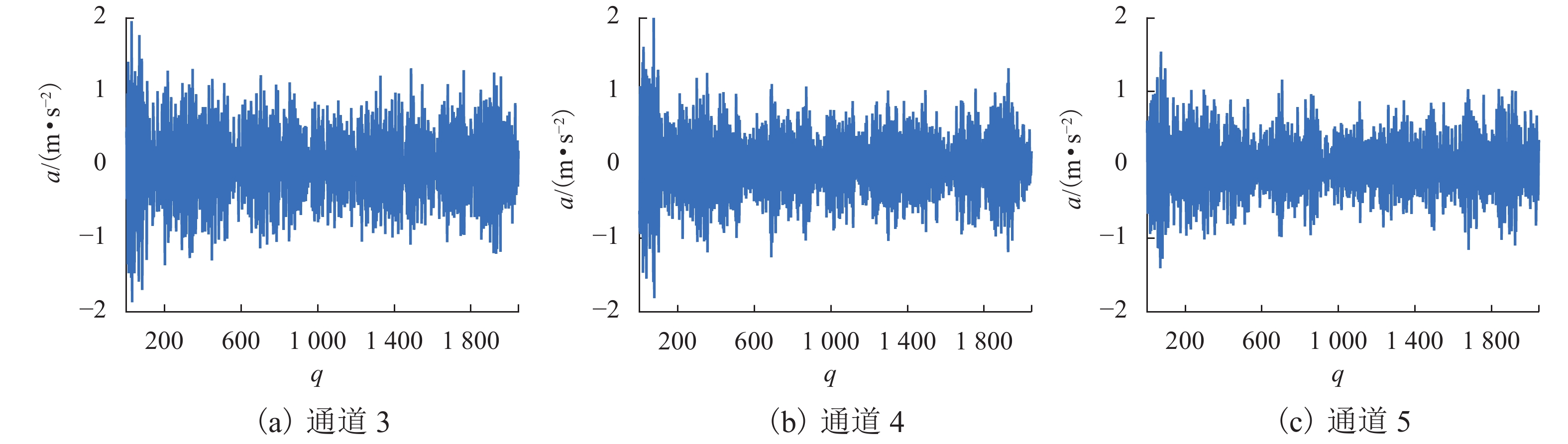
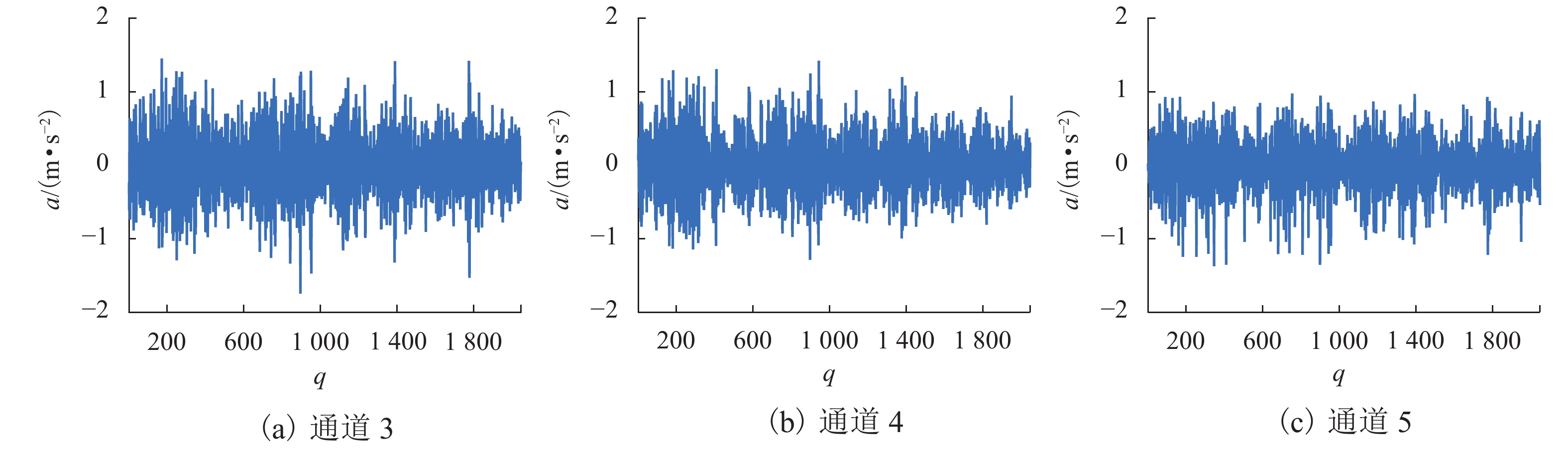
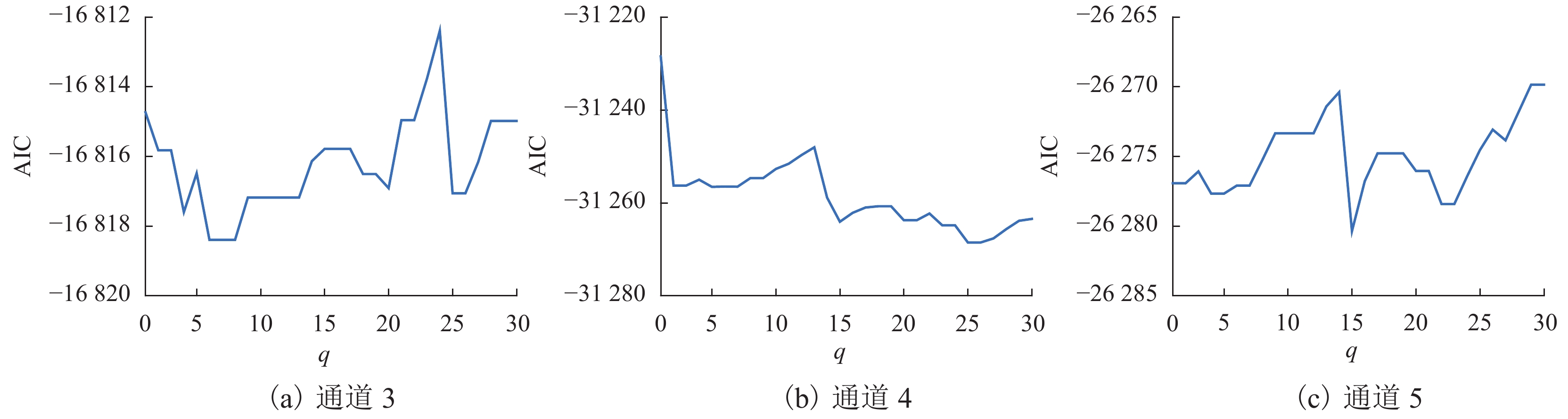
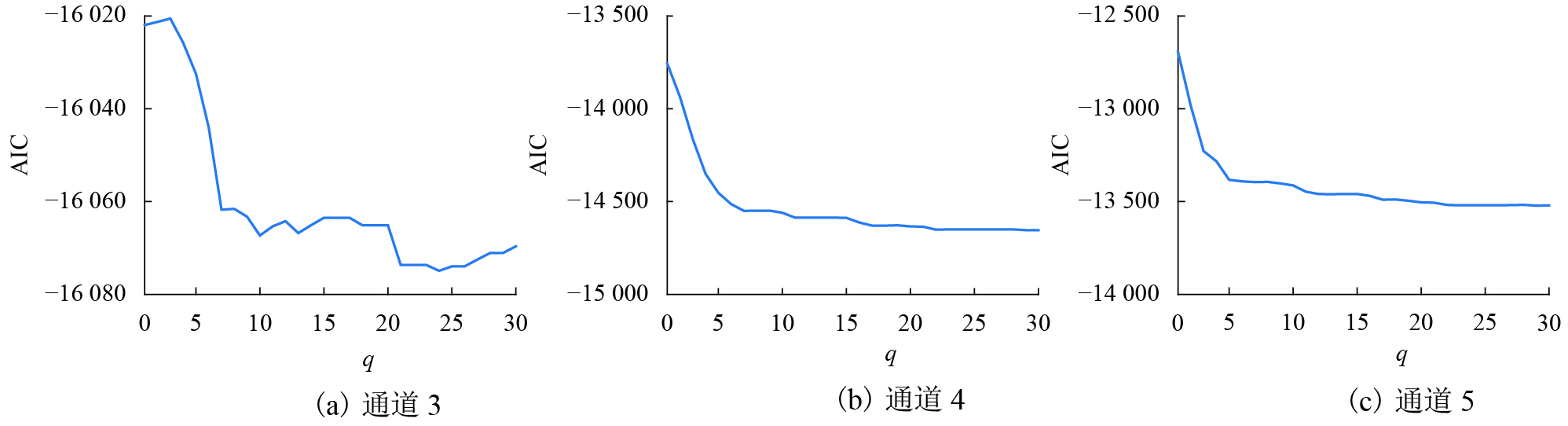
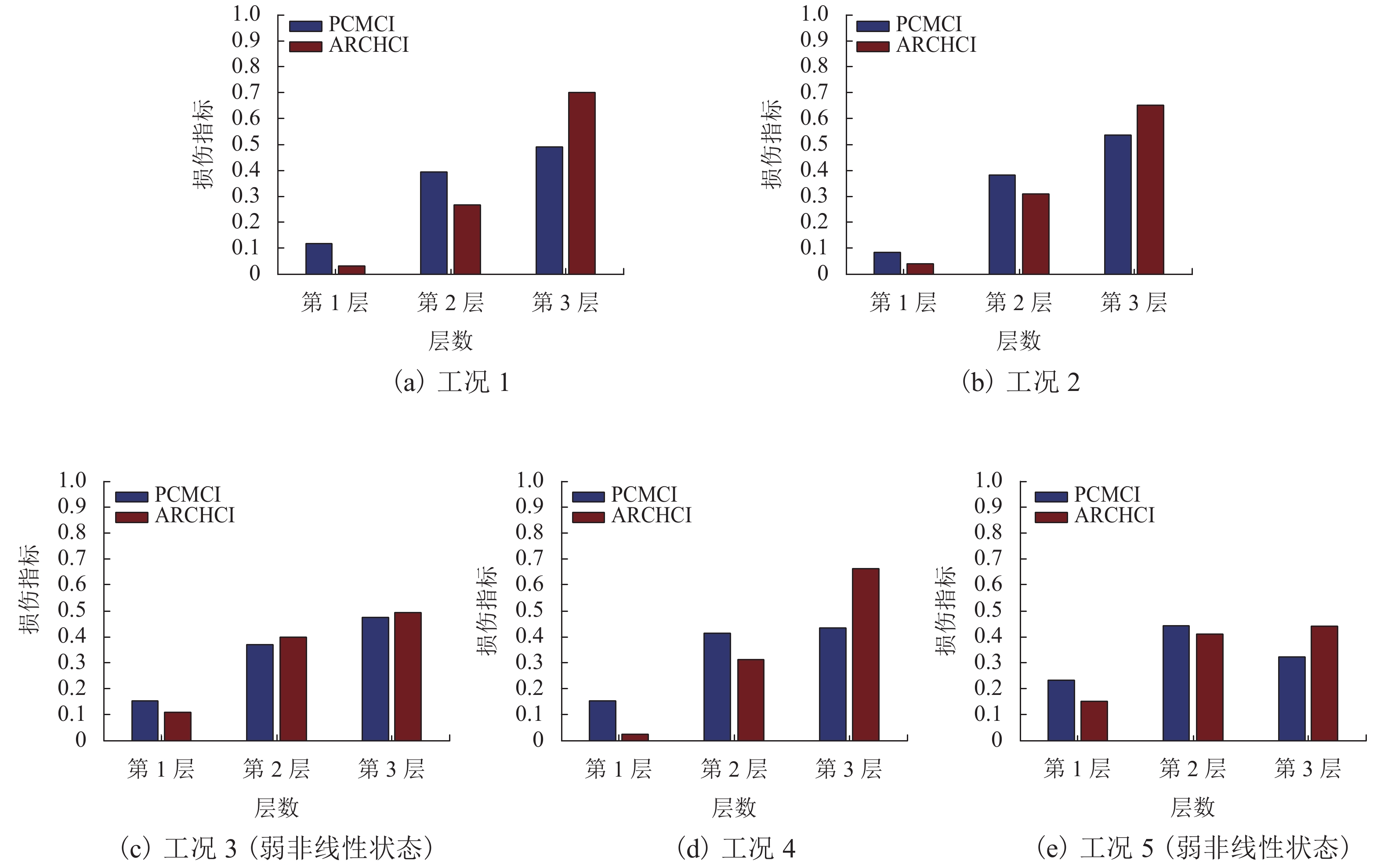
摘要: 为了解决时域非线性损伤的识别问题，基于自回归条件异方差（ARCH）模型的基本理论给出了ARCH模型建模的定阶方法及模型参数估计的极大似然估计法；分析了结构非线性损伤的特性，提出了基于ARCH模型的非线性损伤识别原理；考虑到基于自由度的损伤指标难于判断损伤位置，故提出了一种自回归条件异方差转换指标；在测量误差和模型误差的影响下，使用3层框架结构的非线性损伤试验来验证该损伤指标的有效性. 试验结果表明：非线性间隙距离为0.05 mm和0.10 mm时，损伤位置第3层的自回归条件异方差转换指标值比传统的倒谱测距转化指标值高21.7%以上；当非线性间隙距离为0.20 mm时，第3层的自回归条件异方差转换指标值比倒谱测距转化指标值高3.7%.Abstract: To solve the identification problem of time-domain nonlinear damage, the incorporation of an autoregressive conditional heteroskedasticity (ARCH) model with damage detection was proposed. First, the basic theory of ARCH model was described, and the order estimation and maximum likelihood parameter estimation of ARCH model were proposed. Then, the characteristics of nonlinear damage were analyzed, and a damage detection theory based on ARCH model was presented. Finally, it is difficult for the damage index based on degree of freedom to identify damage locations, an autoregressive conditional heteroskedasticity conversion index (ARCHCI) was proposed. A three-storey frame experiment was used to verify the effectiveness of the ARCHCI, the effect of measurement error and model error was also considered in the experiment. The results show that the ARCHCI value of the damaged third storey is at least 21.7% higher than that of the cepstrum metric conversion index when the nonlinear gap distances are 0.05 mm and 0.10 mm; the ARCHCI value of the third storey is 3.7% higher than that of the cepstrum metric conversion index when the gap distance is 0.20 mm.
-
图 4 基准状态下通道3～5的AIC-q的曲线关系
Figure 4. Relationships of AIC-q of Channel 3～5 in basic State
图 6 指标ARCHCI和PCMCI在不同工况下的损伤识别结果
Figure 6. Damage identification results of ARCHCI and PCMCI in different states
表 1 传感器通道的参数设置
Table 1. Parameter of sensor channels
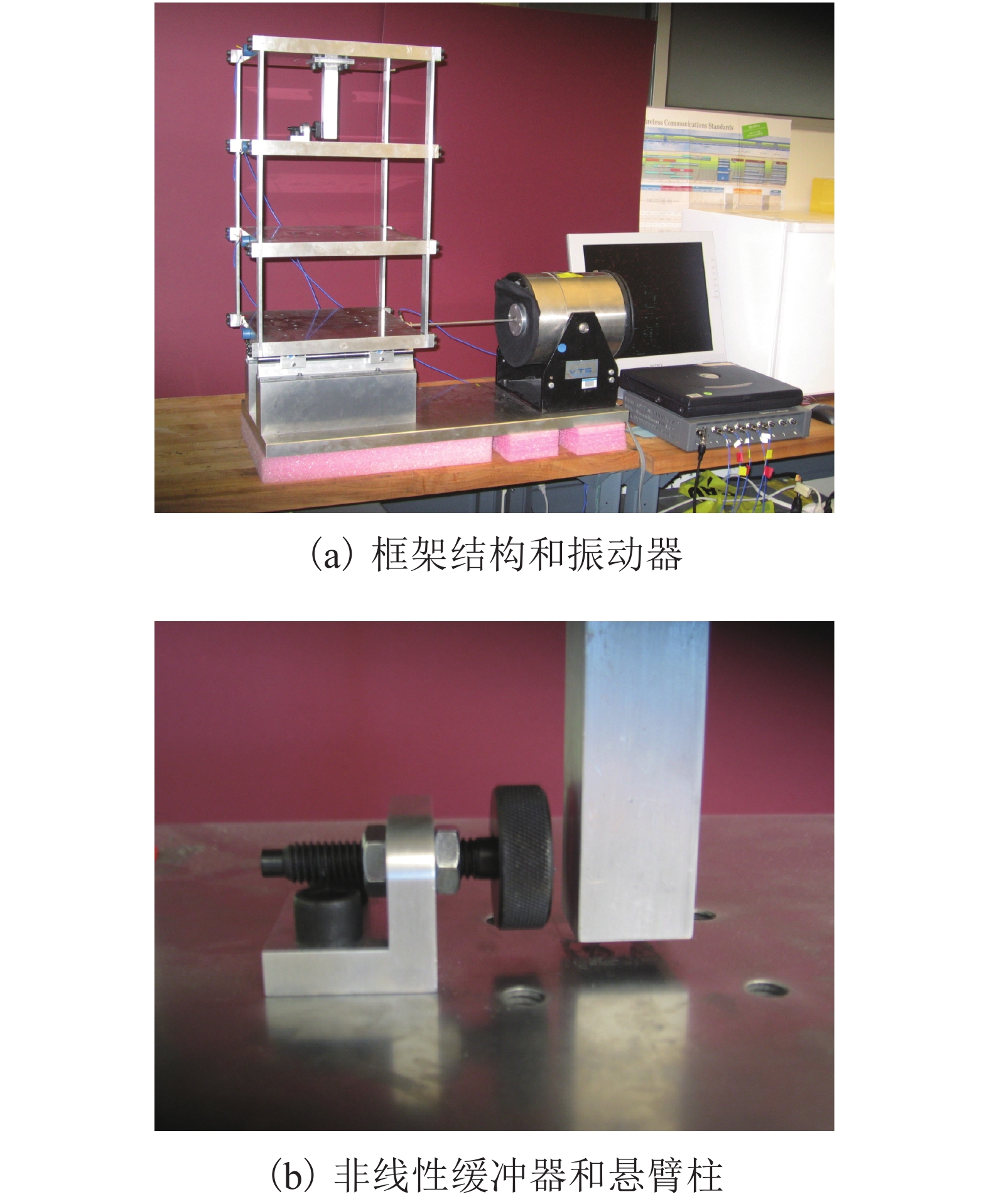
通道名称 传感器 型号 灵敏度 通道 1 测力传感器 PCB 208 C03 SN 22569 2.2 mV/N 通道 2 加速度计 PCB 336C SN 10099 1 000 mV/g 通道 3 加速度计 PCB 336C SN 10120 1 000 mV/g 通道 4 加速度计 PCB 336C SN 9916 1 000 mV/g 通道 5 加速度计 PCB 336C SN 10100 1 000 mV/g 
下载: 导出CSV
表 2 三层框架结构的非线性损伤工况
Table 2. Damaged state of the three-story frame structure
损伤工况 具体描述 工况 1 间隙 0.05 mm 工况 2 间隙 0.10 mm 工况 3 间隙 0.20 mm（弱非线性） 工况 4 间隙 0.10 mm +第 1 层附加质量 1.2 kg 工况 5 间隙 0.20 mm+第 1 层附加
质量 1.2 kg（弱非线性）
下载: 导出CSV
-
CARDEN E P. Vibration based condition monitoring:a review[J]. Structural Health Monitoring, 2004, 3(4): 355-377. doi: 10.1177/1475921704047500 VAN A H. International research projects on structural damage detection[J]. Key Engineering Materials, 2001(204/205): 97-112. 郭惠勇,李正良,彭川. 结构损伤动力识别技术的研究与进展[J]. 土木建筑与环境工程,2008,30(1): 140-145.GUO Huiyong, LI Zhengliang, PENG Chuan. Resear-ches and advances in structural damage dynamic identification technology[J]. Journal of Civil,Architec-tural and Environmental Engineering, 2008, 30(1): 140-145. 闫维明,马裕超,何浩祥,等. 双线性时频分布交叉项提取及损伤识别应用[J]. 振动、测试与诊断,2014,34(6): 1014-1021. doi: 10.3969/j.issn.1004-6801.2014.06.005YAN Weiming, MA Yuchao, HE Haoxiang, et al. Cross-term extraction from bilinear time-frequency distributions and application in structural damage detection[J]. Journal of Vibration,Measurement & Diagnosis, 2014, 34(6): 1014-1021. doi: 10.3969/j.issn.1004-6801.2014.06.005 张立涛,李兆霞,费庆国. 基于加速度时域信息的结构损伤识别方法研究[J]. 振动与冲击,2007,26(9): 138-141. doi: 10.3969/j.issn.1000-3835.2007.09.033ZHANG Litao, LI Zhaoxia, FEI Qingguo. Study on structural damage identification using acceleration data in time domain[J]. Journal of Vibration and Shock, 2007, 26(9): 138-141. doi: 10.3969/j.issn.1000-3835.2007.09.033 KOPSAFTOPOULOS F P, FASSOIS S D. Vibration based health monitoring for a lightweight truss structure:experimental assessment of several statistical time series methods[J]. Mechanical Systems & Signal Processing, 2010, 24(7): 1977-1997. DAS S, SAHA P, PATRO S K. Vibration-based damage detection techniques used for health monitoring of structures:a review[J]. Journal of Civil Structural Health Monitoring, 2016, 6(3): 477-507. doi: 10.1007/s13349-016-0168-5 FASSOIS S D, SAKELLARIOU J S. Time-series methods for fault detection and identification in vibrating structures[J]. Philosophical Transactions of the Royal Society A Mathematical Physical & Engineering Sciences, 2007, 365(1851): 411-448. ZHENG H, MITA A. Localized damage detection of structures subject to multiple ambient excitations using two distance measures for autoregressive models[J]. Structural Health Monitoring, 2009, 8(3): 207-222. doi: 10.1177/1475921708102145 ZHENG H, MITA A. Damage indicator defined as distance between ARMA models for structural health monitoring[J]. Structural Control & Health Monitoring, 2008, 15(7): 992-1005. 吴令红,熊晓燕. 基于时间序列分析的结构损伤检测[J]. 煤矿机电,2009(4): 71-72. doi: 10.3969/j.issn.1001-0874.2009.04.026WU Linghong, XIONG Xiaoyan. Structural damage detection based on time series analysis[J]. Colliery Mechanical & Electrical Technology, 2009(4): 71-72. doi: 10.3969/j.issn.1001-0874.2009.04.026 刘毅,李爱群,丁幼亮,等. 基于时间序列分析的结构损伤特征提取与预警方法[J]. 应用力学学报,2008,25(2): 253-257.LIU Yi, LI Aiqun, DING Youliang, et al. Structural damage feature extraction and alarming using time series analysis[J]. Chinese Journal of Applied Mechanics, 2008, 25(2): 253-257. CHEN L, YU L. Structural nonlinear damage identifi-cation algorithm based on time series ARMA/GARCH model[J]. Advances in Structural Engineering, 2013, 16(9): 1597-1609. doi: 10.1260/1369-4332.16.9.1597 YU L, ZHU J H. Nonlinear damage detection using higher statistical moments of structural responses[J]. Structural Engineering and Mechanics, 2015, 54(2): 221-237. doi: 10.12989/sem.2015.54.2.221 CHENG J J, GUO H Y, WANG Y S. Structural nonlinear damage detection method using ar/arch model[J]. International Journal of Structural Stability and Dynamics, 2017, 17(8): 1750083.1-1750083.27. 李玮峰,段征宇,郭高华. 基于ARCH模型簇的路径行程时间可靠性分析[J]. 交通运输系统工程与信息,2014,14(4): 186-193. doi: 10.3969/j.issn.1009-6744.2014.04.027LI Weifeng, DUAN Zhengyu, GUO Gaohua. Route travel time reliability analysis based on arch model cluster[J]. Journal of Transportation Systems Enginee-ring and Information Technology, 2014, 14(4): 186-193. doi: 10.3969/j.issn.1009-6744.2014.04.027 RÉBILLAT M, HAJRYA R, MECHBAL N. Nonlinear structural damage detection based on cascade of Hammerstein models[J]. Mechanical Sys-tems & Signal Processing, 2014, 48(1/2): 247-259. XING Z, MITA A. Locating the damaged storey of a building using distance measures of low-order AR models[J]. Smart Structures & Systems, 2010, 6(9): 991-1005. -
下载:
点击查看大图
图(6) / 表(2)
计量
- 文章访问数: 544
- HTML全文浏览量: 315
- PDF下载量: 11
- 被引次数: 0