Multiscale Modeling of Transition Section with Continuously Welded Rail Based on Homogenization Method
-
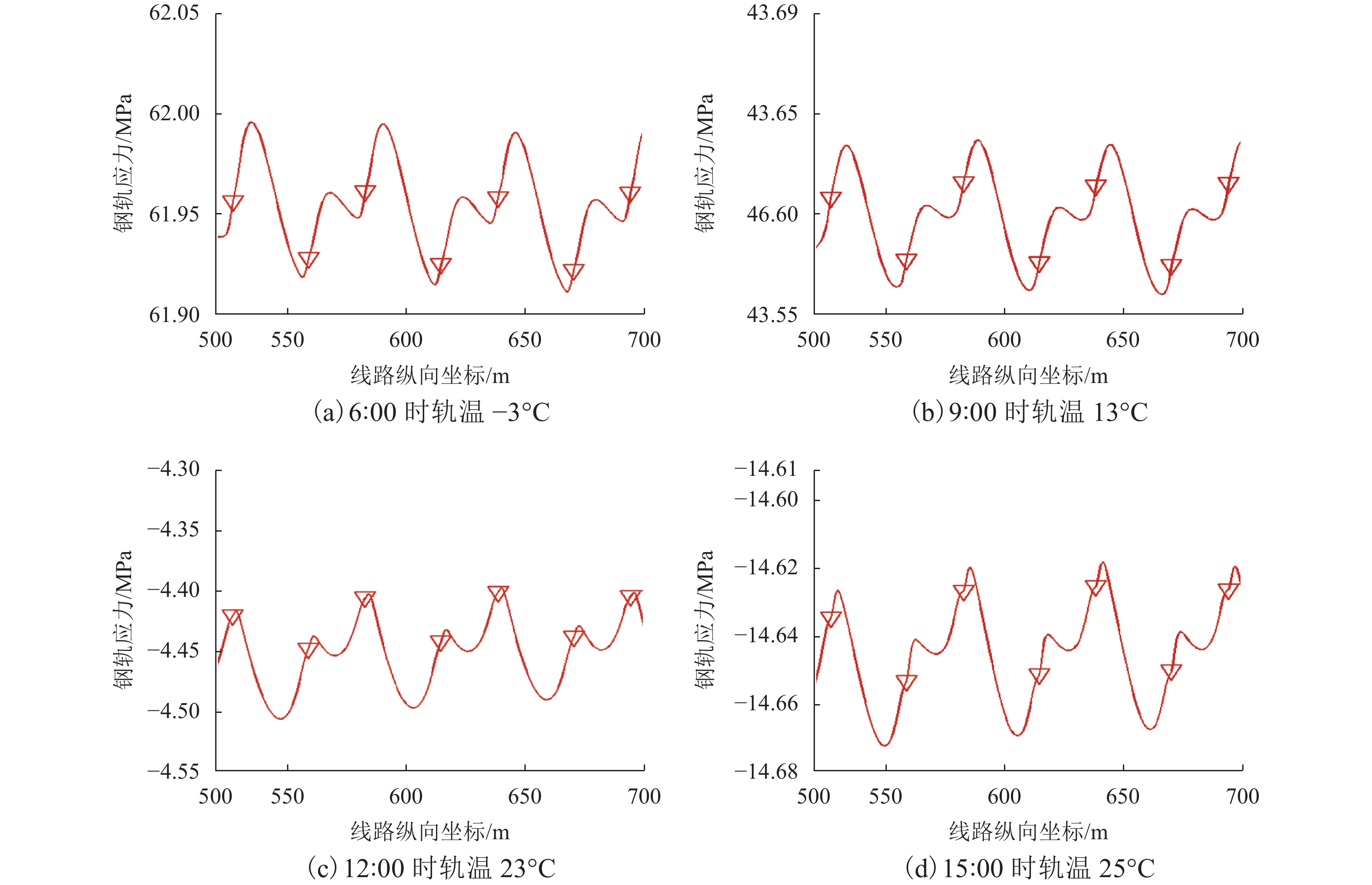
摘要: 为分析无缝线路过渡段的钢轨应力分布问题，提出了针对路桥过渡段及周围延伸线路的多尺度建模方法. 针对过渡段两端的桥梁和路基所具有的周期性延伸的特点，采用渐进均匀化方法计算周期性结构的宏观等效性能，建立过渡段周围区段的宏观尺度模型. 对于过渡段内部，则采用小尺度建立包含关键细节的局部精细化模型. 不同尺度的局部模型之间按变形状态一致的原则建立边界耦合条件，构成完整的长线路模型. 两端线路的宏观均匀化模型为过渡段的局部状态分析提供完整的边界条件，同时通过对整体线路分层求解，避免了常规有限元模型的高维矩阵运算. 仿真试验表明：采用均匀化方法的多尺度模型比传统有限元模型的计算规模更小，因此求解速度更快；模型结果与京沪高速铁路宿州东站新汴河大桥过渡段的现场实测结果变化趋势一致，在主端刺、桥台支座处的轨道变形与实测数据的相关系数达到0.8.Abstract: To analyse the stress distribution of continuously welded rails at transition sections under the effect of temperature, a multiscale model of the transition section with two-terminal rail lines is proposed. Owing to the structural regularity of the two-terminal bridge and the subgrade extending from the transition section, a simplified macro-scale line model is built based on the macro-equivalent properties of a typical structural unit, which can be evaluated by the asymptotic homogenisation method. As for the transition section, a minor-scale model is built to involve the key details. These sub-models of different scales are connected to each other based on the interface consistency conditions. The homogenised model of the two-terminal extension segments provides a useful boundary condition for the central transition section in the process of solving the multiscale model. Further, the solutions of different-scale models are obtained by model decomposition, which avoids high-dimension matrix operations for the single-scale model. The simulation results show that the multiscale model with the asymptotic homogenisation method has fewer computations than the traditional finite model, which consequently has a faster calculating speed. Comparing the simulation results of the termination cutting off and abutments with measurements from the Xinbianhe bridge of the Beijing–Shanghai high speed railway, the average correlation is approximately 0.8, and the varying trend of simulation results is consistent with the measured data.
-
Key words:
- homogenization method /
- railroad engineering /
- finite element method /
- static analysis
-
图 2 考虑滑移特性的阻力-位移关系曲线
Figure 2. Resistance-displacement relationship of slide characteristics
图 3 延伸线路的宏观模型和单元内部结构
Figure 3. Line model in coarse scale and the internal structure of periodic unit
图 5 现场24 h监测数据与仿真结果的比较
Figure 5. Comparisons of the site monitoring data and the simulation results for 24 hours
图 6 主端刺处钢轨响应的极限分布状态
Figure 6. Distribution form of the rail stress and displacement at the termination cutting off
图 7 不同温度下普通桥梁段各单元的钢轨应力分布形态
Figure 7. Distribution form of the rail stress in the girder spans at various temperatures
图 8 采用均匀化法和有限元法计算钢轨应力结果的比较
Figure 8. Comparison of rail stress for homogenisation method and finite element method
表 1 部分轨道结构参数
Table 1. Parameters of the transition section
参数 计算值 参数 计算值 钢轨 CHN60 地基模量/MPa 120 扣件最大纵向
阻力/kN无载6.5 扣件最大弹性
位移/mm无载0.5 端刺刚度/
（MN•mm–1）100 固结机构/
（MN•mm–1）103 滑动层摩擦
因数0.3 滑动层位移
上限/mm0.5 滑动层纵向
刚度/（N•m–1）13 × 103 滑动层垂向
刚度/（N•m–1）1.25 × 109 桥墩顶纵向水平
刚度/（kN•mm–1）20 桥台顶纵向水平
刚度/（kN•mm–1）150 
下载: 导出CSV
-
BAEZA L, OUYANG H. A railway track dynamics model based on modal substructuring and a cyclic boundary condition[J]. Journal of Sound Vibration, 2011, 330(1): 75-86 doi: 10.1016/j.jsv.2010.07.023 李兆霞,李爱群,陈鸿天,等. 大跨桥梁结构以健康监测和状态评估为目标的有限元模拟[J]. 东南大学学报(自然科学版),2003,33(5): 562-572 doi: 10.3321/j.issn:1001-0505.2003.05.007LI Zhaoxia, LI Aiqun, CHEN Hongtian, et al. Finite element modeling for health monitoring and condition assessment of long-span bridges[J]. Journal of Southeast University (Natural Science Edition), 2003, 33(5): 562-572 doi: 10.3321/j.issn:1001-0505.2003.05.007 GARCIA-PALACIOS J, SAMARTIN A, MELIS M. Analysis of the railway track as a spatially periodic structure[J]. Journal of Rail and Rapid Transit, 2012, 226(2): 113-123 doi: 10.1177/0954409711411609 吴佰建,李兆霞,汤可可. 大型土木结构多尺度模拟与损伤分析-从材料多尺度力学到结构多尺度力学[J]. 力学进展,2007,37(3): 321-335 doi: 10.3321/j.issn:1000-0992.2007.03.001WU Baijian, LI Zhaoxia, TANG Keke. Multi-scale modeling and damage analyses of large civil structure-multi-scale mechanics from material to structure[J]. Advances in Mechanics, 2007, 37(3): 321-335 doi: 10.3321/j.issn:1000-0992.2007.03.001 BENSOUSSAN A, LIONS J L, PAPANICOLAU G. Asymptotic analysis for periodic structures[M]. Ameterdam: North-Holland Publishing Company, 1978: 12-35 FISH J, CHEN W. Higher-order homogenization of initial boundary-value problem[J]. Journal of Engineering Mechanics, 2001, 127(12): 1223-1230 doi: 10.1061/(ASCE)0733-9399(2001)127:12(1223) FISH J, CHEN W, NAGAL G. Non-local dispersive model for wave propagation in heterogeneous media:one-dimensional case[J]. International Journal for Numerical Methods in Engineering, 2002, 54(54): 331-346 CUI J Z, SHIN T M, WANG Y L. The two-scale analysis method for bodies with small periodic configurations[J]. Structural Engineering and Mechanics, 1996, 7(6): 601-614 崔俊芝,曹礼群. 基于双尺度渐进分析的有限元算法[J]. 计算数学,1998,20(1): 89-101CUI Junzhi, CAO Liqun. Finite element method based on two-scale asymptotic analysis[J]. Mathematica Numerica Sinica, 1998, 20(1): 89-101 XU Q Y, ZHOU X L, ZENG Z P. Mechanics model of additional longitudinal force transmission between bridges and continuously welded rails with small resistance fasteners[J]. Journal of Central South University of Technology, 2004, 11(3): 336-339 doi: 10.1007/s11771-004-0069-3 高亮. 高速铁路无缝线路关键技术研究与应用[M]. 北京: 中国铁道出版社, 2012: 111-121 LI Z G, WU T X. Modelling and analysis of force transmission in floating-slab track for railways[J]. Journal of Rail and Rapid Transit, 2008, 222(1): 45-57 doi: 10.1243/09544097JRRT145 WAGNER G J, LIU W K. Coupling of atomistic and continuum simulations using a bridging scale decomposition[J]. Journal of Computational Physics, 2003, 190(1): 249-274 doi: 10.1016/S0021-9991(03)00273-0 BERGAN P G, HORRIGMOE G, BRAKELAND B, et al. Solution techniques for non-linear finite element problems[J]. International Journal for Numerical Methods in Engineering, 1978, 12(11): 1677-1696 doi: 10.1002/(ISSN)1097-0207 -
下载:
点击查看大图
计量
- 文章访问数: 443
- HTML全文浏览量: 197
- PDF下载量: 15
- 被引次数: 0