Analysis of Entire Load-Deformation Process of Shear Span Ratio Not More Than 2.0: Reinforcement of Concrete Squat Walls by Strut-Tie Model
-
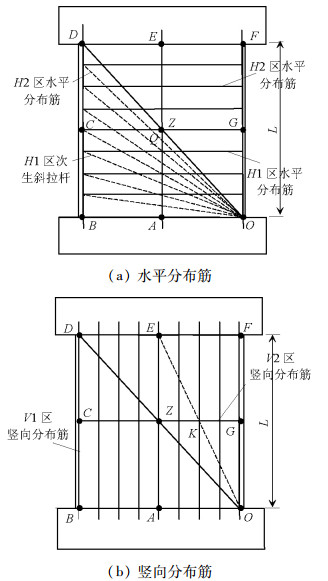
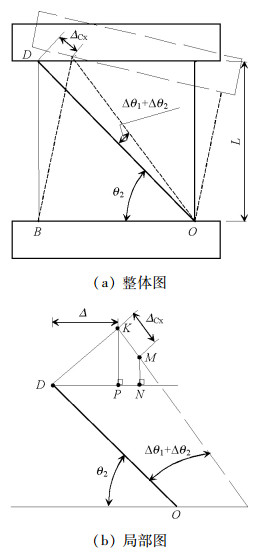
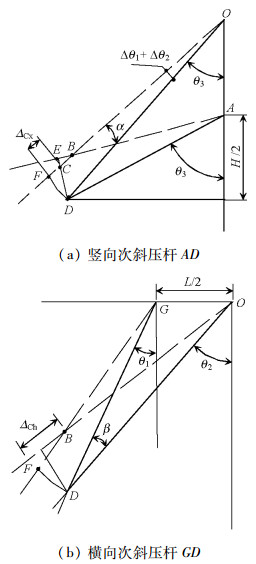
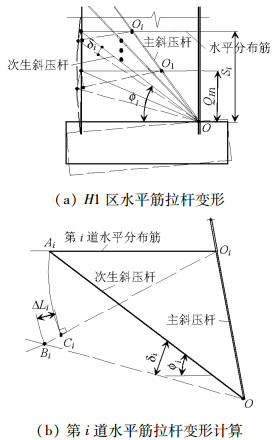
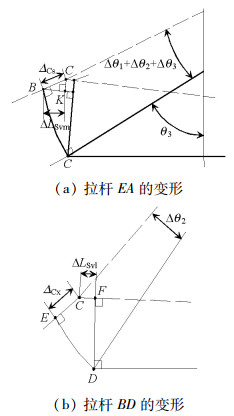
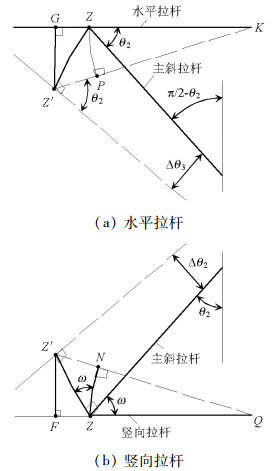
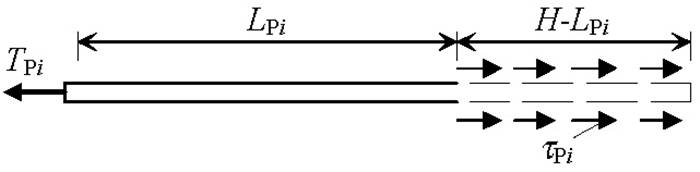
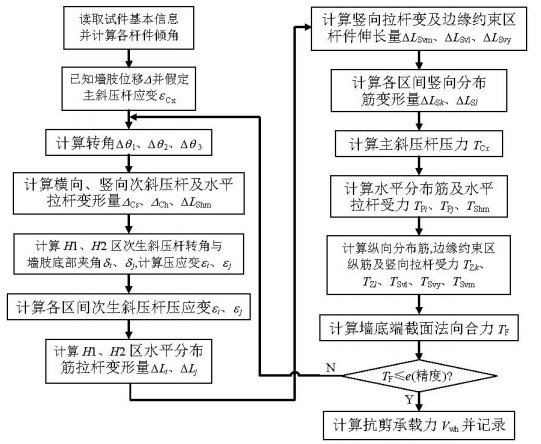
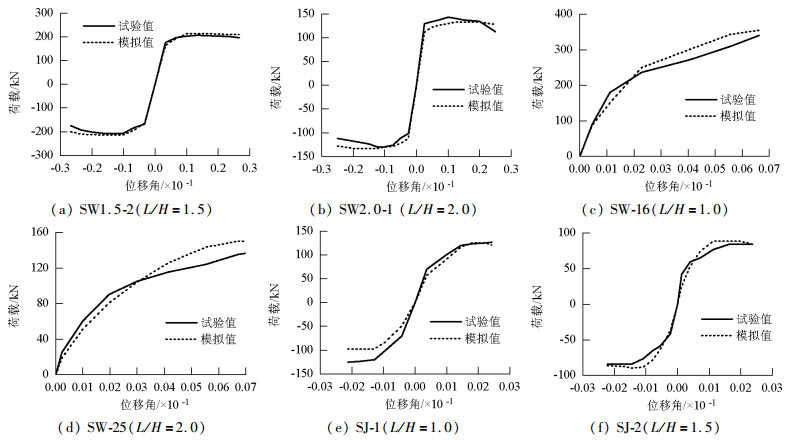
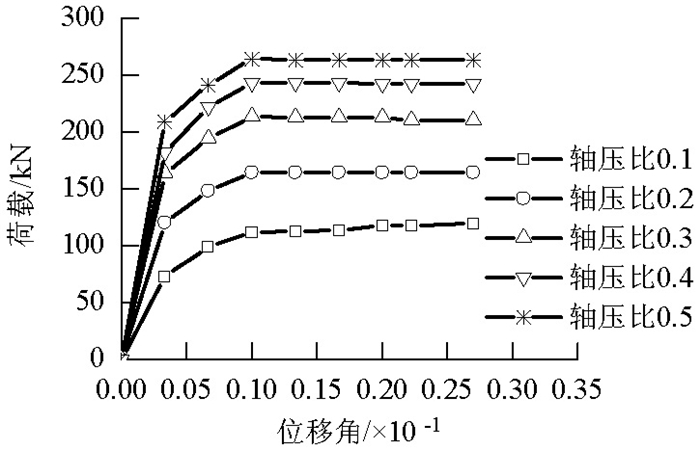
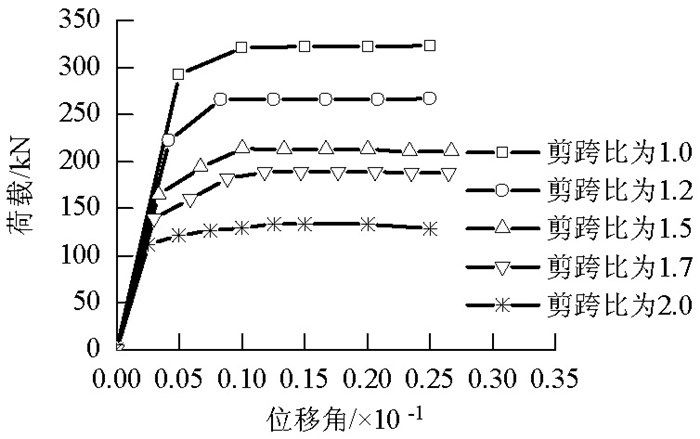
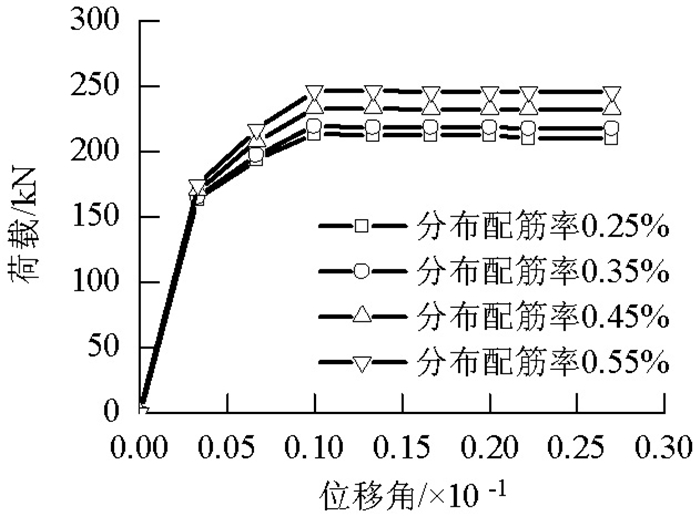
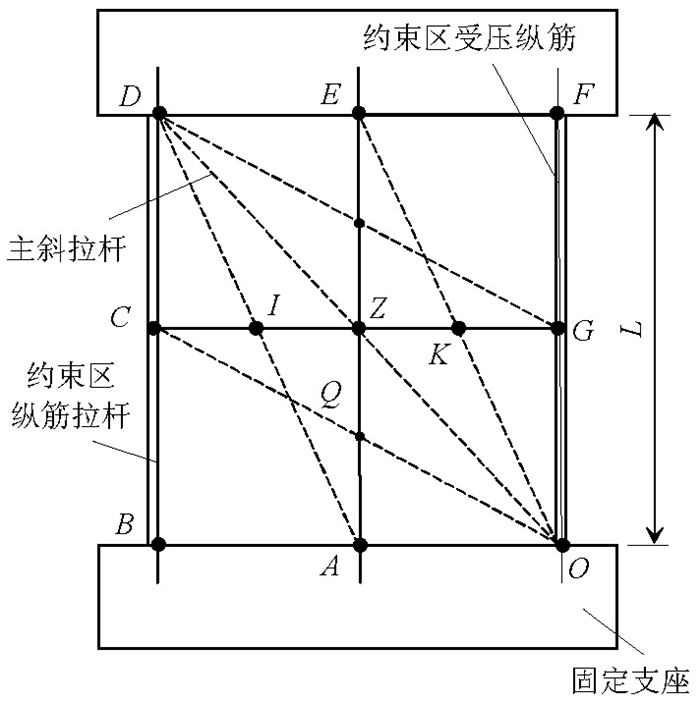
摘要: 为实现以剪切为主的（剪跨比不大于2.0）钢筋混凝土剪力墙力-位移全过程计算，在拉压杆模型基础上通过合理化假定提出了考虑变形协调的改进拉压杆模型.模型由对角斜向混凝土压杆、混凝土次斜压杆、混凝土次生斜压杆、水平拉杆、竖向拉杆及墙肢分布筋拉杆等组成，定量确定了模型中对角斜压杆及次斜压杆变形与墙端位移间的关系，建立了各杆件之间的变形协调条件、物理方程和平衡方程等计算式.此外应用该模型分析了轴压比，剪跨比及墙肢分布配筋率三种参数对剪力墙力-位移骨架曲线的影响.研究结果表明：与6片剪力墙试验结果对比，该模型能够较好地模拟剪跨比不大于2.0、以剪切受力特征为主的钢筋混凝土剪力墙力-位移骨架曲线；当轴压比由0.1依次增至0.5时，峰值承载力最大增量为27%；剪跨比由1.0依次增至2.0时，峰值承载力最大减少30%；分布配筋率由0.25%依次增至0.55%时，峰值承载力最大增量为6%；相比于其余两个参数，配筋率对墙肢承载能力的影响最小.Abstract: An improved strut-and-tie model that considers the deformation compatibility for determining the load-deformation relationship for squat walls (shear span ratio smaller than 2.0) is proposed. The model originates from the strut-and-tie model, and it includes a diagonal concrete strut, secondary concrete struts, subsidiary concrete struts, horizontal tie, vertical tie, and shear reinforcement ties. A definite equation between the deformation of two types of concrete struts (a diagonal concrete strut and four secondary concrete struts) and the displacement of the squat walls is given. The relationship among compatibility, equilibrium, and constitutive laws in each of the strut and ties was established. In addition, parameter analyses were conducted using the improved strut-and-tie model to study the effect of axial load, aspect ratio, and distribution reinforcement ratio on the skeleton curves of shear walls. The results show that, compared with the test results for six shear walls, the forces and deformations of the predicted members' are in reasonable agreement with test results. During parameter analysis, when the axial load ratio increases from 0.1 to 0.5, the maximum growth of load-carrying capacity is 27%. As the aspect ratio of shear walls increases from 1.0 to 2.0, the load-carrying capacity decreases 30%. As the distribution reinforcement ratio increases from 0.25% to 0.55%, the load-carrying capacity increases only 6%. The effect of the distribution reinforcement ratio on load-carrying capacity is not significant compared with the other two parameters.
-
图 2 钢筋混凝土剪力墙分布筋拉压杆模型
Figure 2. Strut-tie model for horizontal and vertical reinforcement of RC squat wall
图 14 不同分布配筋率下的P-β骨架曲线
Figure 14. P-β skeleton curves for different distribution reinforcement ratios
表 1 试件各级位移迭代次数
Table 1. Iterations of specimens in each displacement level
次 试件编号 1级位移 2级位移 3级位移 4级位移 5级位移 6级位移 7级位移 8级位移 SW1.5-2 16 16 14 15 18 19 23 22 SW2.0-1 17 18 18 17 19 19 20 24 SW-16 19 18 20 19 18 19 — — SW-25 18 18 20 20 21 20 19 23 SJ-1 18 17 19 19 20 18 — — SJ-2 19 17 19 20 18 18 16 — 注:“—”表示试件未进行该位移级加载. 
下载: 导出CSV
表 2 各参数变化对抗剪承载力的影响
Table 2. Effects of parameters on shear strength
轴压比 承载力/kN 剪跨比 承载力/kN 配筋率/% 承载力/kN 0.1 119.20 1.0 323.32 0.25 212.69 0.2 164.32 1.2 266.21 0.35 218.56 0.3 212.00 1.5 212.69 0.45 232.25 0.4 242.28 1.7 188.52 0.55 246.15 0.5 254.80 2.0 133.15 — —
下载: 导出CSV
-
伍云天, 唐欢, 林雪斌.内置冷弯薄壁型钢桁架高强混凝土剪力墙抗震性能试验研究[J].建筑结构学报, 2014, 35(11):44-52. http://d.old.wanfangdata.com.cn/Periodical/jzjgxb201411006WU Yuntian, TANG Huan, LIN Xuebin. Experimental study on seismic behavior of high strength concrete shear walls with embedded cold-formed and thin-walled steel truss[J]. Journal of Building Structures, 2014, 35(11):44-52. http://d.old.wanfangdata.com.cn/Periodical/jzjgxb201411006 刘成清, 韦小丹, 倪向勇.短肢剪力墙抗震性能理论分析与数值模拟[J].西南交通大学学报, 2016, 51(4):690-696. doi: 10.3969/j.issn.0258-2724.2016.04.013LIU Chengqing, WEI Xiaodan, NI Xiangyong. Seismic performance of short-pier shear walls:theoretical analysis and numerical simulation[J]. Journal of Southwest Jiaotong University, 2016, 51(4):690-696. doi: 10.3969/j.issn.0258-2724.2016.04.013 SCHLAICH J, SCHAFER K, JENNEWEIN M.Toward a consistent design of structural concrete[J]. PCI J, 1987, 32(3):75-150. GUPTA A, RANGAN B V. High-strength concrete(HSC) structural wall[J]. ACI Structure Journal, 1998, 95(2):194-204. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0230054457/ KASSEMW, ELSHEIKH A. Estimation of shear strength of structural shear walls[J]. Journal of Structural Engineering, 2010, 136(10):1215-1224. doi: 10.1061/(ASCE)ST.1943-541X.0000218 LEONARDO M, MASSONE.Shear response estimate for squat reinforced concrete walls via a single plane model[J]. Earthquakes and Structures, 2014, 7(5):647-665. doi: 10.12989/eas.2014.7.5.647 初明进.冷弯薄壁型钢混凝土剪力墙抗震性能研究[D].北京: 清华大学, 2010. SCHLAICH J, SCHAFER K.Design and detailing of structural concrete using strut-and-tie models[J]. Structural Engineer, 1991, 69(6):113-125. HWANG S J, FANG W H, LEE H J, et al. Analytical model for predicting shear strength of squat walls[J]. Journal of Structural Engineering, 2001, 127(1):43-50. doi: 10.1061/(ASCE)0733-9445(2001)127:1(43) FRANK J, VECCHIO M, COLLINS P. The modified compression-field theory for reinforced concrete elements subjected to shear[J]//Journal Proceedings.1986, 83(2): 219-231. HWANG S J, LEE H J. Analytical model for predicting shear strengths of interor reinforced concrete beam-column joints for seismic resistance[J]. ACI Structural Journal, 2000, 97(1):35-44. HWANG S J, LU WY, LEE H C.Shear strength prediction for deep beams[J]. ACI Structural Journal, 2000, 97(3):367-376. doi: 10.1016-j.jcsr.2006.02.007/ HWANG S J, LEE H J. Strength prediction for discontinuity regions by softened strut-and-tie model[J]. Journal of Structural Engineering, 2002, 128(12):1519-1526. doi: 10.1061/(ASCE)0733-9445(2002)128:12(1519) YU H W, HWANG S J.Evaluation of softened truss model for strength prediction of reinforced concrete squat walls[J].Journal of Engineering Mechanics, 2005, 131(8):839-846. doi: 10.1061/(ASCE)0733-9399(2005)131:8(839) KASSEM W.Shear strength of squat walls:A strut-and-tie model and closed-form design formula[J]. Engineering Structures, 2014, 11(27):430-438. http://www.sciencedirect.com/science/article/pii/S0141029614007159 刘霞, 张鹄志, 易伟建, 等.钢筋混凝土开洞深梁拉压杆模型方法与经验方法试验对比研究[J].建筑结构学报, 2013, 34(7):139-147. http://d.old.wanfangdata.com.cn/Periodical/jzjgxb201307017LIU Xia, ZHANG Huzhi, YI Weijian, et al. Testing comparison between strct-and-tie model method and empirical method for RC deep beams with openings[J]. Journal of Building Structures, 2013, 34(7):139-147. http://d.old.wanfangdata.com.cn/Periodical/jzjgxb201307017 田建勃, 史庆轩, 王南, 等.基于软化拉-压杆模型的小跨高比钢板-混凝土组合连梁受剪承载力分析[J].工程力学, 2016, 33(5):142-149. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QKC20162016061700006530TIAN Jianbo, SHI Qingxuan, WANG Nan, et al. Shear strength of plate-reinforced coposite coupling beams with small span-to-depth ratio using softened strut-and-tie model[J]. Engineering Mechanics, 2016, 33(5):142-149. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QKC20162016061700006530 BALI I, HWANG S J.Strength and deflection prediction of double-curvature reinforced concrete squat walls[J]. Structural Engineering and Mechanics, 2007, 27(4):501-521. doi: 10.12989/sem.2007.27.4.501 YOUNG M Y. Nonlinear strut-tie model approach for structural concrete[J]. ACI Structural Journal, 2000, 97(4):581-590. http://scitation.aip.org/getabs/servlet/GetabsServlet?prog=normal&id=JCCEE5000014000002000127000001&idtype=cvips&gifs=Yes 曹万林, 殷伟帅, 杨兴民.双向单排配筋中高剪力墙抗震性能试验研究[J].地震工程与工程振动, 2009, 29(1):103-108. http://d.old.wanfangdata.com.cn/Periodical/dzgcygczd200901014CAO Wanlin, YIN Weishuai, YANG Xingmin. Experimental study on seismic performance of medium-height RC shear wall with bi-directional single row of steel bars[J]. Journal of Earthquake Engineering and Engineering Vibration, 2009, 29(1):103-108. http://d.old.wanfangdata.com.cn/Periodical/dzgcygczd200901014 曹万林, 孙天兵, 杨兴民.双向单排配筋混凝土高剪力墙抗震性能试验研究[J].世界地震工程, 2008, 24(3):14-19. http://d.old.wanfangdata.com.cn/Periodical/sjdzgc200803003CAO Wanlin, SUN Tianbing, YANG Xingmin. Experimental study on seismic performance of high-rise shear wall with bi-directional single row of steel bars[J]. World Earthquake Engineering, 2008, 24(3):14-19. http://d.old.wanfangdata.com.cn/Periodical/sjdzgc200803003 LEFAS I D, KOTSOVOS M D, AMBRASEYS N N. Behavior of reinforced concrete structural walls:Strength, deformation characteristics, and failure mechanism[J]. Structural Journal, 1990, 87(1):23-31. http://d.old.wanfangdata.com.cn/Periodical/jzjgxb201804011 李兵, 李宏男.不同剪跨比钢筋混凝土剪力墙拟静力试验研究[J].工业建筑, 2010, 40(9):32-36. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK201002133491LI Bing, LI Hongnan. Research on quasi-static test of reinforced concrete shear walls with different shear-span ratio[J]. Industrial Construction, 2010, 40(9):32-36. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK201002133491 -
下载:
点击查看大图
图(14) / 表(2)
计量
- 文章访问数: 430
- HTML全文浏览量: 149
- PDF下载量: 61
- 被引次数: 0