Dynamic Robertson's Platoon Dispersion Model in Connected Vehicle Environment
-
摘要: 传统Robertson车队离散模型参数估计是基于历史数据,不能很好地反映交通流的动态变化特征,为解决这一问题,构建了车联网环境下的动态Robertson车队离散模型.考虑到车联网环境下车辆的行程时间数据易于获得,基于此可对Robertson模型的相关参数进行实时动态估计建立动态Robertson车队流量离散模型.通过实际调查数据,分析了上游交叉口车辆离去流率与下游交叉口车辆到达流率的关系,并将文中模型与静态Robertson模型、实际观测数据进行了比较分析.结果表明,文中动态模型更能反映交通流的车队离散规律,与静态Robertson模型相比,平均预测均方误差减少了30.68%.Abstract: The parameters of the traditional Robertson's platoon dispersion model are based on historical data, and thus, it cannot provide a good reflection of the dynamic characteristics of traffic flow. In order to solve this problem, a connected vehicle environment is considered, and the travel time of the vehicles is easily obtained. The relevant parameters of the Robertson's model can be estimated in real time based on this. Then, a dynamic Robertson's platoon dispersion model was proposed. Later, the relationship between the arrival flow rate of the downstream intersection and the departing flow rate of the upstream intersection was analysed using the proposed model with field collected data, and compared with those obtained using the traditional Robertson's model and actual data. The results show that the proposed model can better describe the law of dispersion in traffic flow, and the mean squared error of prediction is reduced by approximately 30.68%, compared with the traditional Robertson's model.
-
Key words:
- traffic engineering /
- connected vehicles /
- platoon dispersion /
- dynamic parameters /
- signal control
- 致谢: 西南交通大学优秀博士学位论文培育项目(D-YB201708).
-
表 1 行程时间相关参数
Table 1. Parameters of travel time
时段 车辆数/辆 公交比例/% 行程时间均值/s 行程时间标准差/s 1 264 19.41 54.38 16.92 2 623 12.20 53.94 15.44 3 550 11.92 52.28 13.69 4 411 8.41 50.29 12.08 表 2 Robertson模型参数估计表
Table 2. Parameters of Robertson's model
时段 Ta/s F α β 1 54.38 0.06 0.43 0.70 2 53.94 0.06 0.38 0.72 3 52.28 0.07 0.34 0.75 4 50.29 0.08 0.30 0.77 表 3 两种模型均方误差表
Table 3. Mean squared error of two models
模型 时段1 时段2 时段3 时段4 平均值 静态 0.003 4 0.005 7 0.004 0 0.004 6 0.004 4 本文 0.003 0 0.003 3 0.004 0 0.001 9 0.003 1 改进百分比/% -9.82 -42.60 0 -58.83 -30.68 -
ROESS R P, PRASSAS E S, MCSHANE W R. Traffic engineering:united states edition[M]. Englewood Cliffs:Prentice-Hall, 2010:5-10. JIANG Yangsheng, YAO Zhihong, LUO Xiaoling, et al. Heterogeneous platoon flow dispersion model based on truncated mixed simplified phase-type distribution of travel speed[J]. Journal of Advanced Transportation, 2016, 50:2160-2173. doi: 10.1002/atr.v50.8 BONNESON J, PRATT M, VANDEHEY M. Predicting arrival flow profiles and platoon dispersion for urban street segments[J]. Transportation Research Record:Journal of the Transportation Research Board, 2010, 2173:28-35. doi: 10.3141/2173-04 JIANG Yangsheng, YAO Zhihong, DING Xiao, et al. Mixed platoon flow dispersion model based on truncated mixed phase distribution of speed//Transportation Research Board of the National Academies.[2016-05-17]. https://pubsindex.trb.org/view/2016/C/1393937. JIANG Yi, LI Shuo, SHAMO D E. A platoon-based traffic signal timing algorithm for major-minor intersection types[J]. Transportation Research Part B Methodological, 2006, 40(7):543-562. doi: 10.1016/j.trb.2005.07.003 PACEY G M. The progress of a bunch of vehicles released from a traffic signal[R]. London: Transport and Road Research Laboratory, 1956. GRACE M J, POTTS R B. A theory of the diffusion of traffic platoons[J]. Operations Research, 1964, 12(2):255-275. doi: 10.1287/opre.12.2.255 ROBERTSON D I. TRANSYT: a traffic network study tool[R]. London: Transport and Road Research Laboratory, 1969. https://trid.trb.org/view/115048 HILLIER J A, ROTHERY R. The synchronization of traffic signals for minimum delay[J]. Transportation Science, 1967, 1(2):81-94. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=10.1177/1545968308323779 SEDDON P A. Another look at platoon dispersion 3:the recurrence relationship[J]. Traffic Engineering and Control, 1972, 13(10):442-444. HUNT P, ROBERTSON D, BRETHERTON R, et al. SCOOT: a traffic responsive method of coordinating signals[R]. London: Transport and Road Research Laboratory, 1981. https://trid.trb.org/view/179439 HALL M, WILLUMSEN L G. SATURN:a simulation-assignment model for the evaluation of traffic management schemes[J]. Traffic Engineering & Control, 1980, 21(4):81-94. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0227422397/ LIEBERMAN E B, ANDREWS B. TRAFLO:a new tool to evaluate transportation system management strategies[J]. Transportation Research Record:Journal of the Transportation Research Board, 1980, 772:9-15. http://trid.trb.org/view/167530 TRACZ M. The prediction of platoon dispersion based on rectangular distribution of journey time[J]. Traffic Engineering & Control, 1975, 16(11):25-36. https://trid.trb.org/view.aspx?id=45341 POLUS A. A study of travel time and reliability on arterial routes[J]. Transportation, 1979, 8(2):141-151. doi: 10.1007/BF00167196 YU Lei. Calibration of platoon dispersion parameters on the basis of link travel time statistics[J]. Transportation Research Record:Journal of the Transportation Research Board, 2000, 1727:89-94. doi: 10.3141/1727-11 BIE Yiming, LIU Zhiyuan, MA Dongfang, et al. Calibration of platoon dispersion parameter considering the impact of the number of lanes[J]. Journal of Transportation Engineering, 2013, 139(2):200-207. doi: 10.1061/(ASCE)TE.1943-5436.0000443 PAUL B, MITRA S, MAITRA B. Calibration of Robertson's platoon dispersion model in non-lane based mixed traffic operation[J]. Transportation in Developing Economies, 2016, 2(2):1-14. doi: 10.1007/s40890-016-0016-7 姚志洪, 蒋阳升, 吴云霞, 等.基于速度服从混合PH分布的车队离散模型[J].交通运输系统工程与信息, 2016, 16(3):133-140. doi: 10.3969/j.issn.1009-6744.2016.03.020YAO Zhihong, JIANG Yangsheng, WU Yunxia, et al. Platoon dispersion model based on mixed phase distribution of speed[J]. Journal of Transportation Systems Engineering and Information Technology, 2016, 16(3):133-140. doi: 10.3969/j.issn.1009-6744.2016.03.020 姚志洪, 沈旅欧, 巫威眺, 等.基于行程时间分布的异质交通流车队离散模型[J].中国公路学报, 2016, 29(8):134-142, 151. doi: 10.3969/j.issn.1001-7372.2016.08.016YAO Zhihong, SHEN Lüou, WU Weitiao, et al. Heterogeneous traffic flow platoon dispersion model based on travel time distribution[J]. China Journal of Highway and Transport, 2016, 29(8):134-142, 151. doi: 10.3969/j.issn.1001-7372.2016.08.016 巫威眺, 沈旅欧, 靳文舟.基于速度截断分布和流量的车队离散模型[J].西南交通大学学报, 2014, 49(1):126-133. doi: 10.3969/j.issn.0258-2724.2014.01.020WU Weitiao, SHEN Lüou, JIN Wenzhou. Platoon flow dispersion model based on truncated normal distribution of speed[J]. Journal of Southwest Jiaotong University, 2014, 49(1):126-133. doi: 10.3969/j.issn.0258-2724.2014.01.020 LEE J, PARK B. Development and evaluation of a cooperative vehicle intersection control algorithm under the connected vehicles environment[J]. IEEE Transactions on Intelligent Transportation Systems, 2012, 13(1):81-90. doi: 10.1109/TITS.2011.2178836 FENG Yiheng. Intelligent traffic control in a connected vehicle environment[D]. Arizona: The University of Arizona, 2015. TIAPRAPRASERT K, ZHANG Yunlong, WANG Xiubin, et al. Queue length estimation using connected vehicle technology for adaptive signal control[J]. IEEE Transactions on Intelligent Transportation Systems, 2015, 16(4):2129-2140. doi: 10.1109/TITS.2015.2401007 FENG Yiheng, HEAD K L, KHOSHMAGHAM S, et al. A real-time adaptive signal control in a connected vehicle environment[J]. Transportation Research Part C Emerging Technologies, 2015, 55:460-473. doi: 10.1016/j.trc.2015.01.007 YU Lei. Real-time calibration of platoon dispersion model to optimizethe coordinated traffic signal timings in ATMS networks[R]. Houston: Center for TransportationTraining and Research, Texas Southern University, Houston, 1999. 期刊类型引用(1)
1. 闻磊,林玮平,张涛. 流量控制功能在车联网后装市场流量运营需求度分析. 广东通信技术. 2018(10): 10-12+44 .  百度学术
百度学术其他类型引用(6)
-





 下载:
下载:
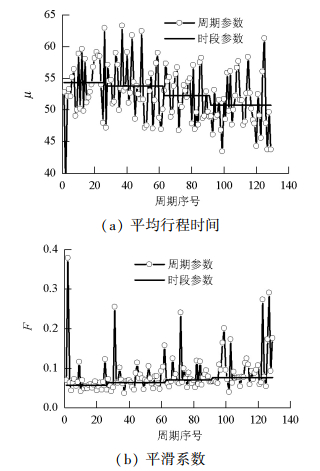
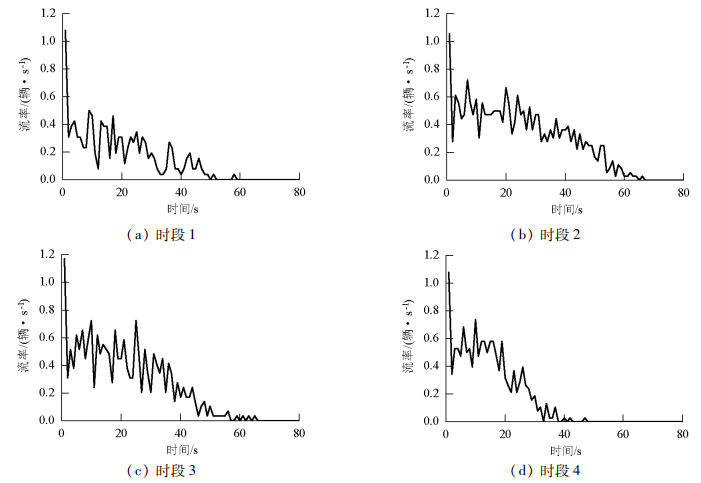
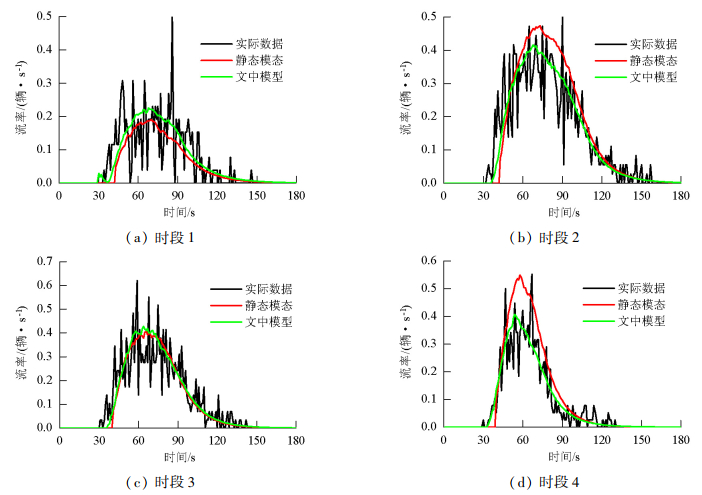
 百度学术
百度学术
