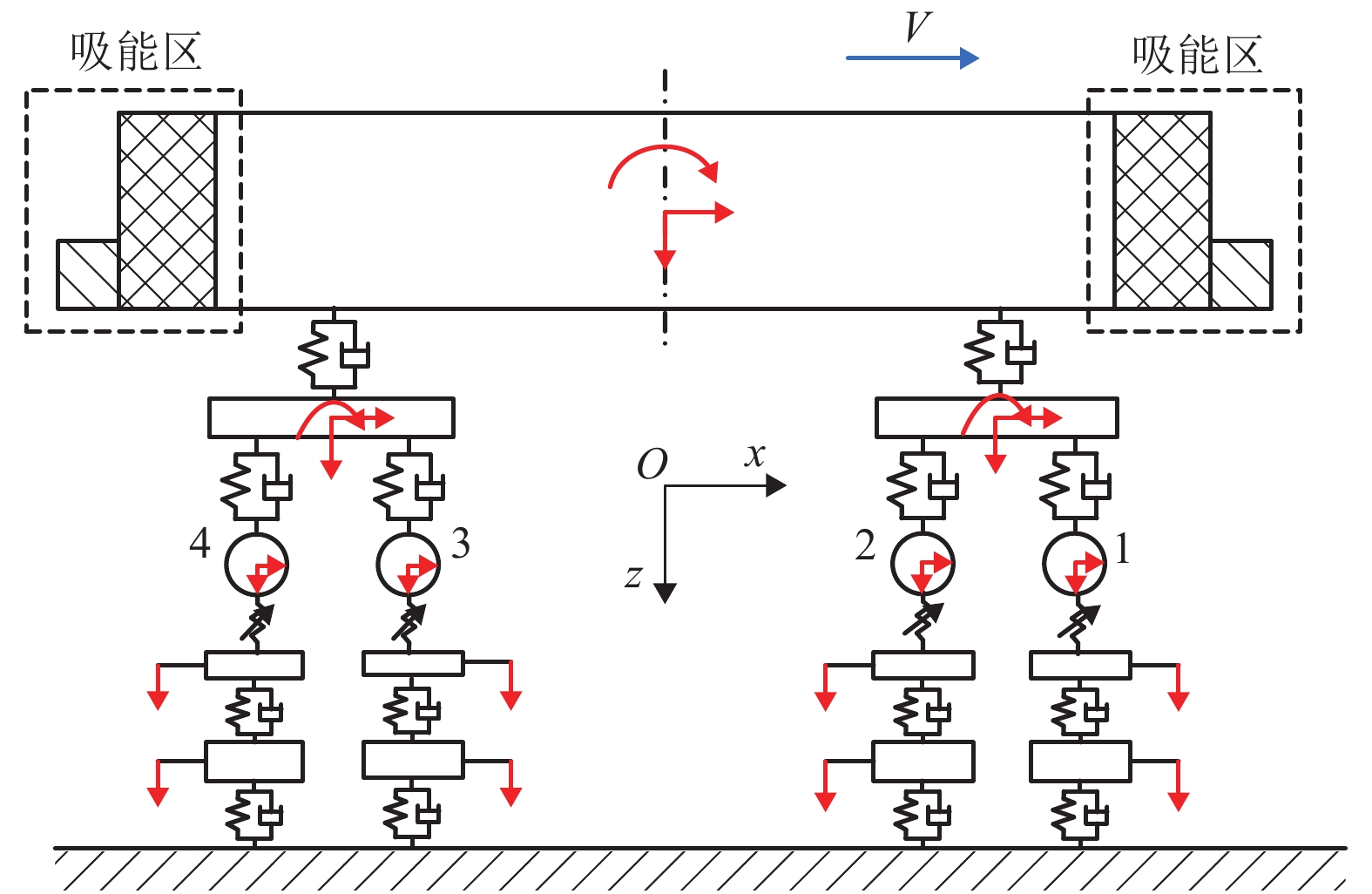
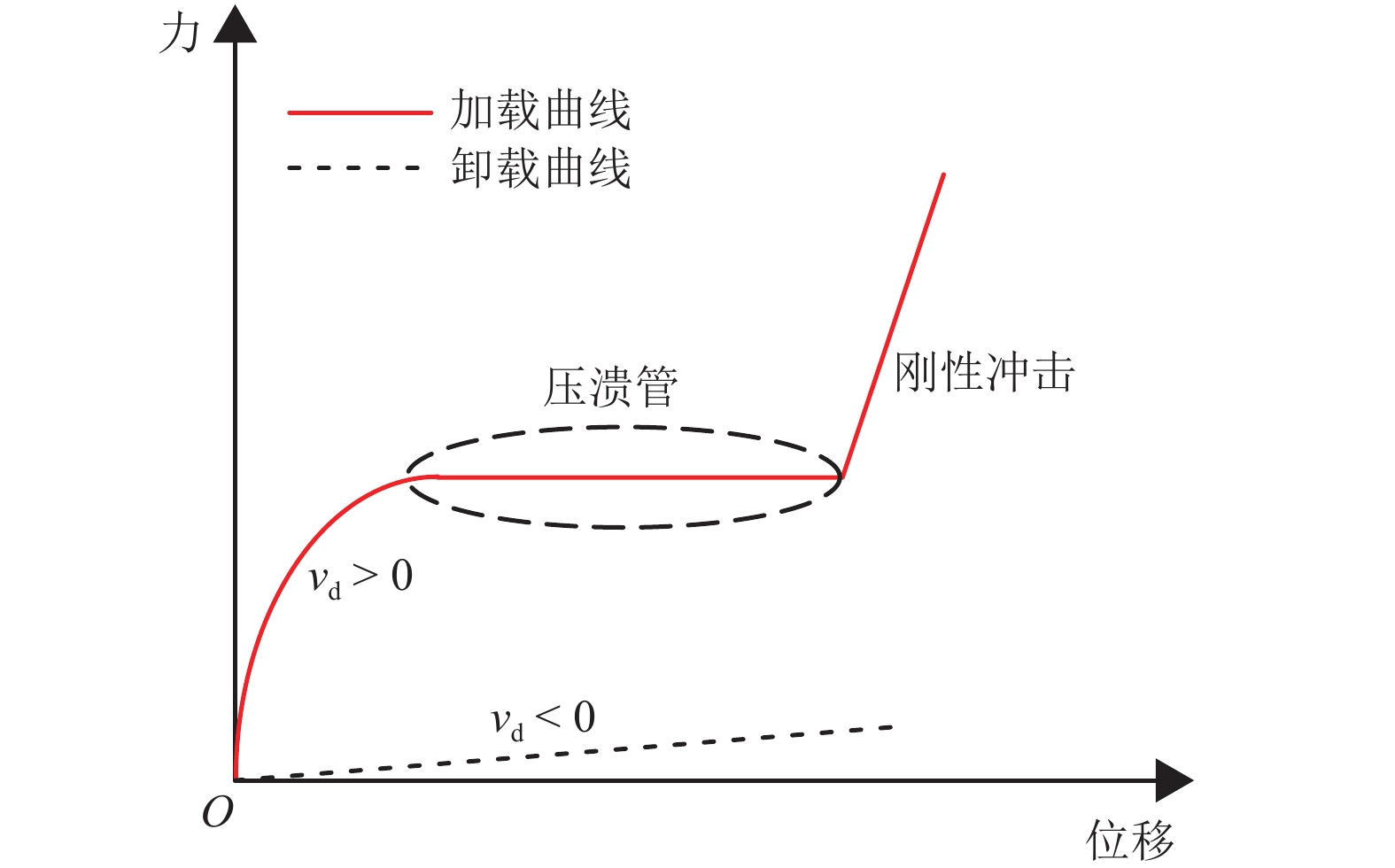
Overriding Behavior in Train Collisions Based on Moving Vehicle-Track Coupled Model
-
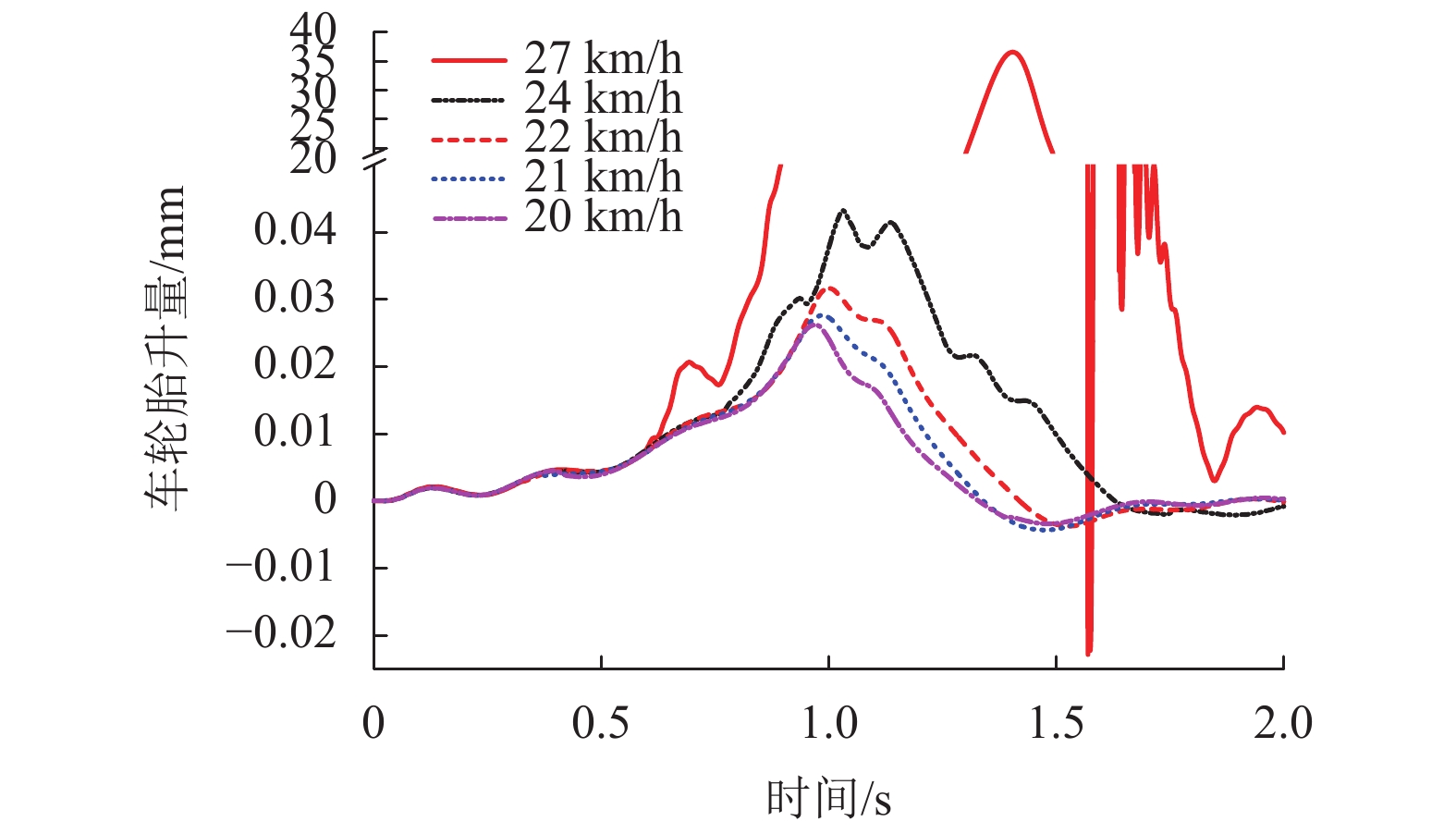
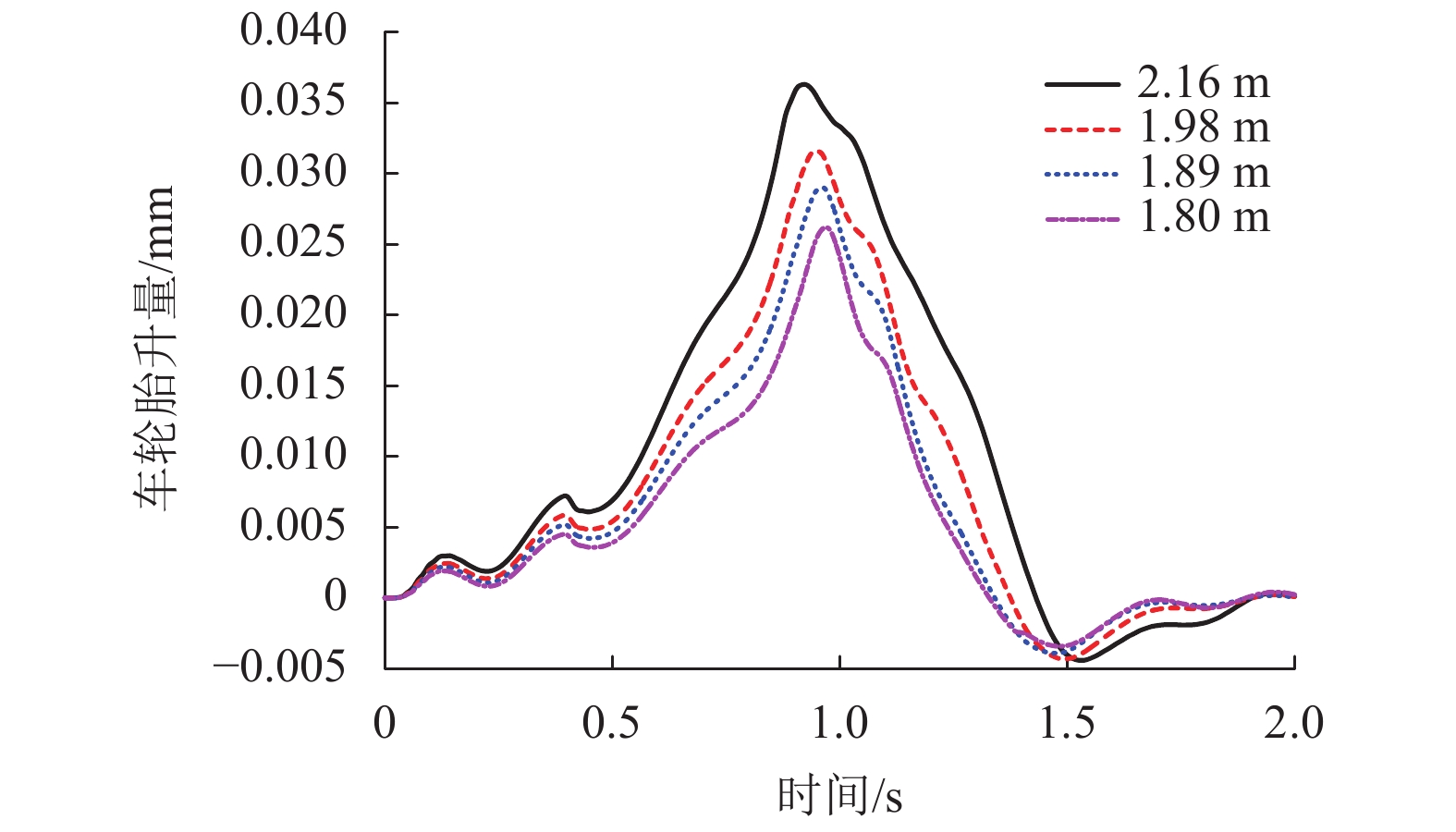
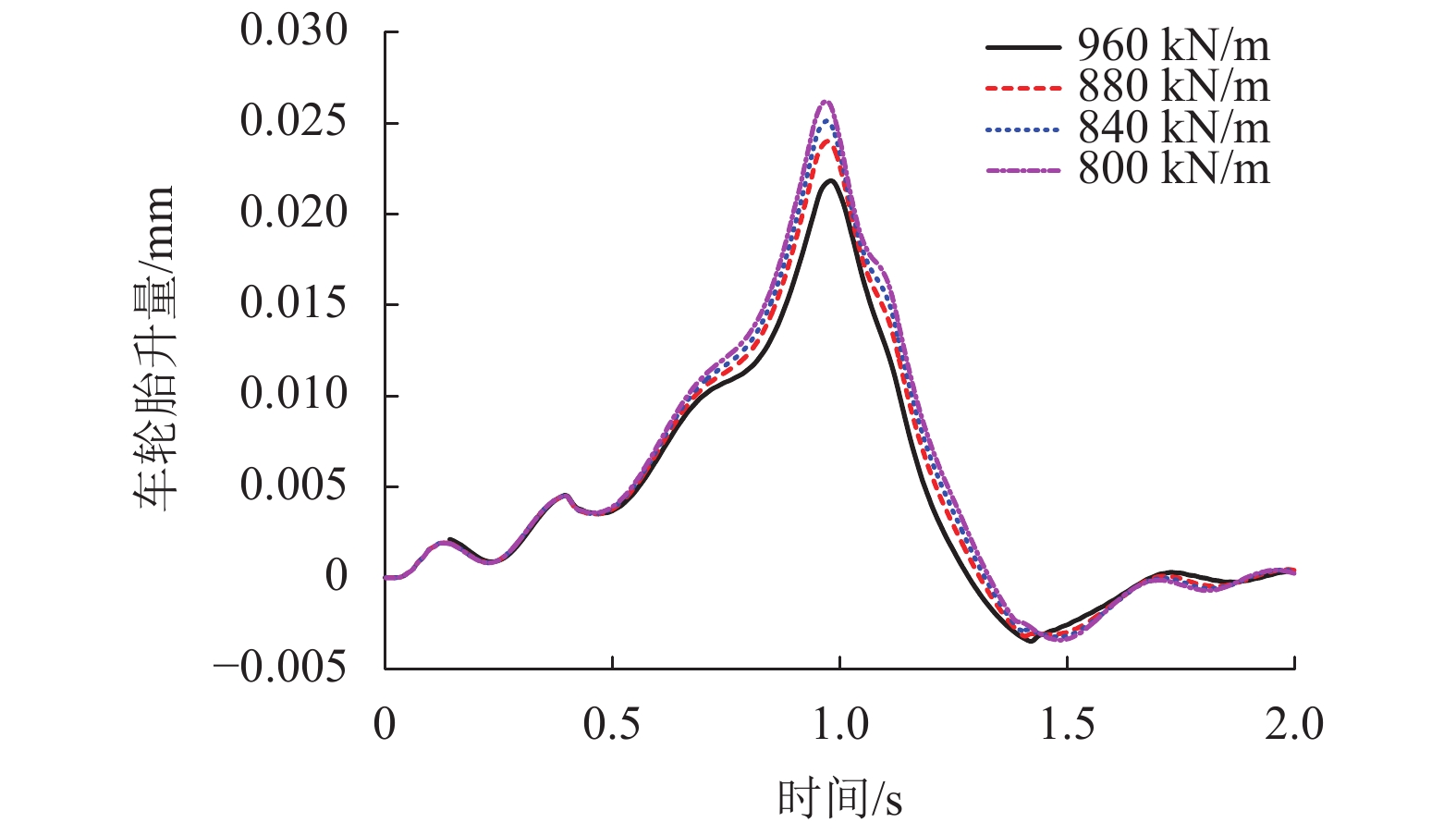
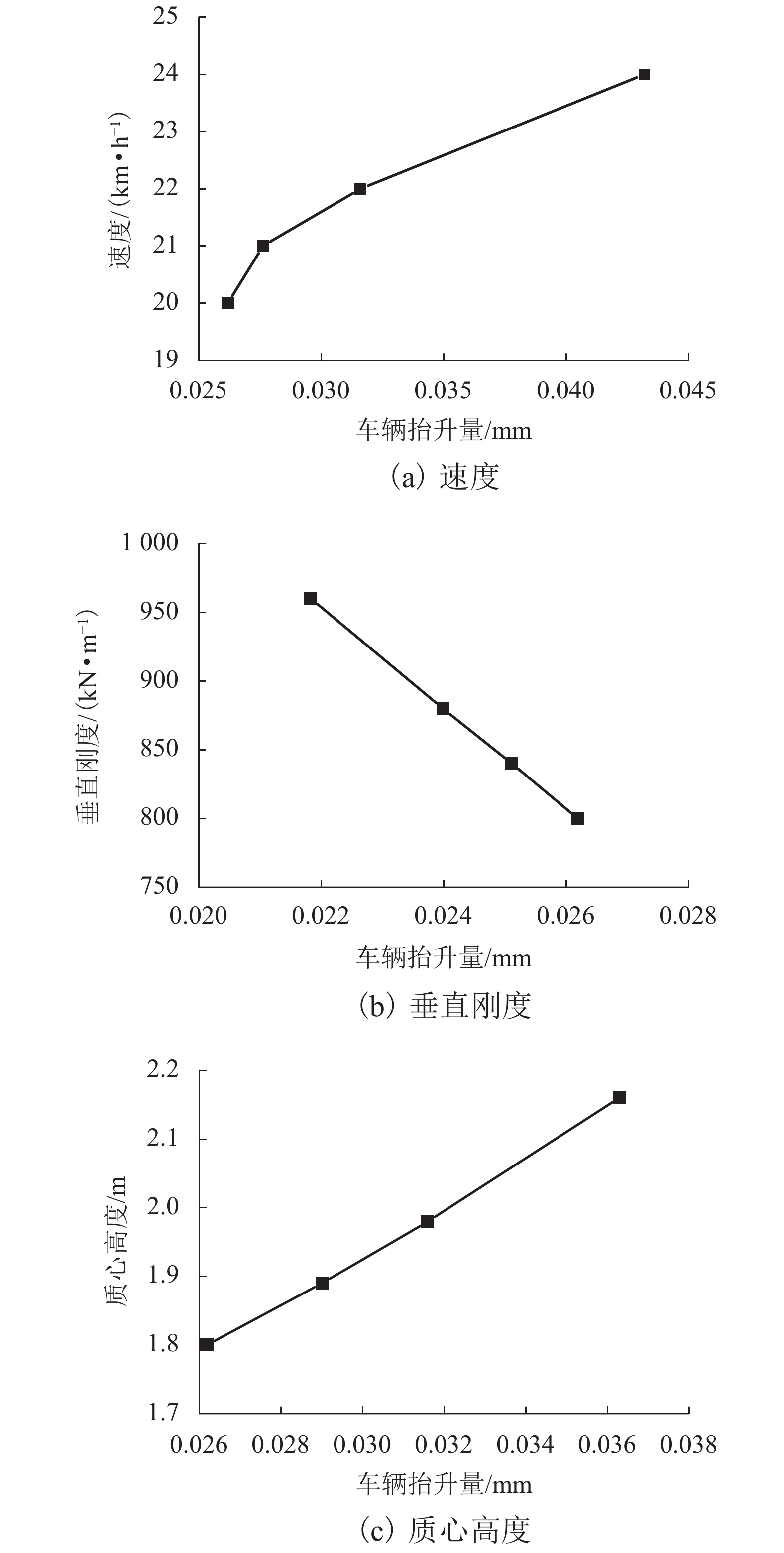
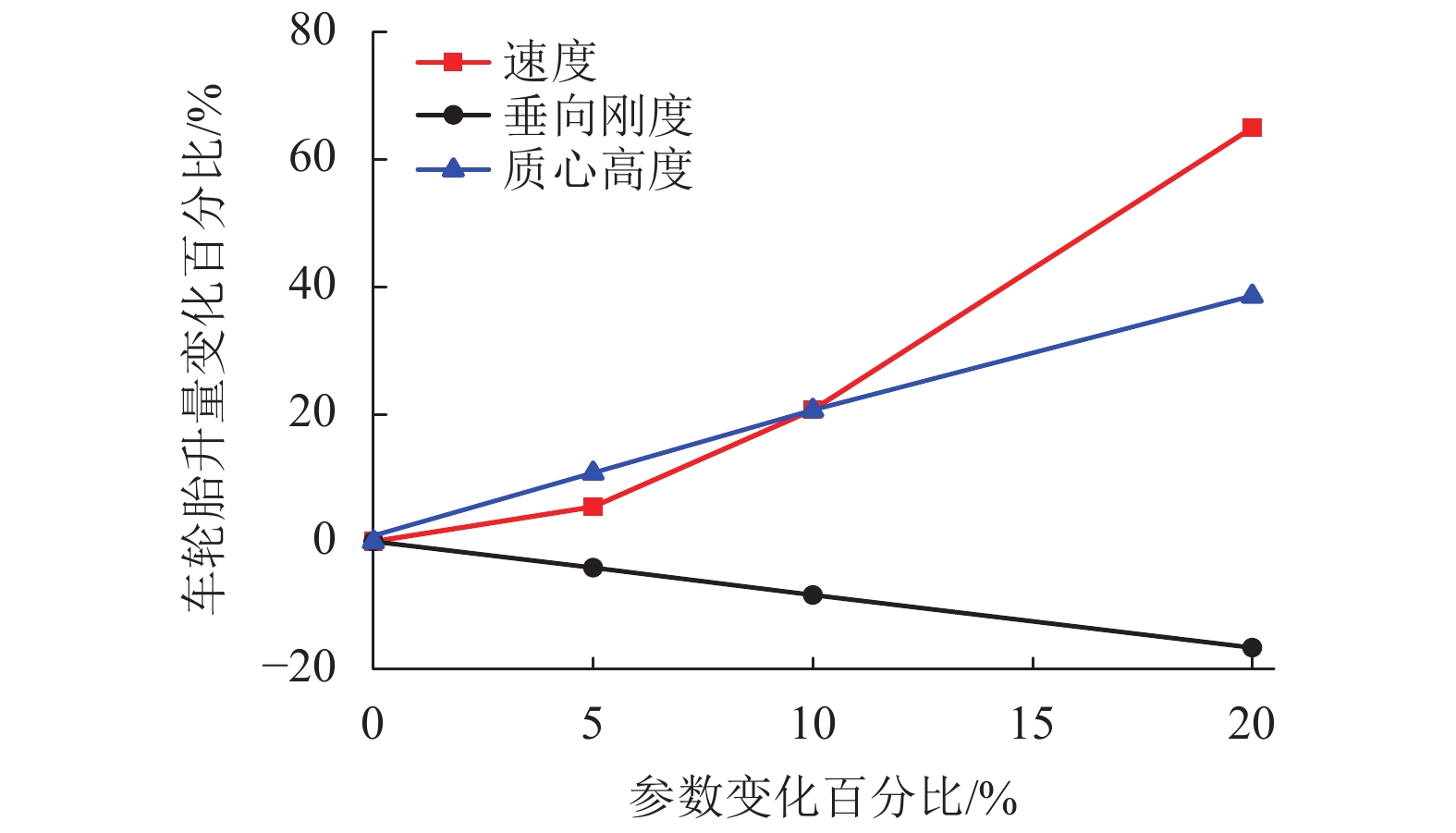
摘要: 为了揭示车辆参数对列车碰撞爬车行为的影响规律，首先基于车轨耦合的基本思路，建立车辆模型和移动轨道模型，用非线性轮轨接触模型耦合车辆模型和移动轨道模型；非线性钩缓装置模型用于连接相邻的两个车辆模型；然后通过模拟两同型列车低速正面碰撞，获得了不同参数情况下车辆和轨道的动态响应；最后用车轮抬升量作为车辆碰撞爬车指标，分析了车轮抬升量对碰撞速度、车体质心高度和二系垂向刚度的灵敏度和相对灵敏度. 结果表明：在其他条件不变的情况下，当碰撞速度增大至27 km/h时，车轮抬升量陡增至36.5 mm；质心高度增大20%时，车轮抬升量增加41%；二系垂向刚度增大20%时，车轮抬升量减小16.6%；车轮抬升量随碰撞速度和质心高度的增大而增大，而随着二系垂向刚度的增大而减小；车轮抬升量对碰撞速度的灵敏度是非线性的；质心高度和二系垂向刚度的相对灵敏度分别为205%和−83%.Abstract: In order to reveal how vehicle parameters affect the overriding behavior in train collisions, the vehicle model and the moving track model were developed in view of the basic ideas of vehicle-track interaction. The nonlinear wheel-rail contact model was applied in coupling both models. A nonlinear coupler model was used to connect two adjacent vehicle models. Then the head-on collision of two identical trains was simulated at low speeds. The dynamic responses of vehicles and tracks were obtained with different parameters. Finally, the wheel lift was taken as the vehicle-collision overriding index. The sensitivity and the relative sensitivity were analyzed in terms of wheel lift to impact speed, to height of car body mass center and to vertical stiffness of secondary suspensions. The results indicate that when other conditions remain unchanged, the wheel lift rapidly increases to 36.5 mm if the impact speed increases to 27 km/h. The wheel lift increases by 41% when the height of mass center increases by 20%. However, it decreases by 16.6% if the vertical stiffness of secondary suspension increases by 20%. In other words, the wheel lift increases with the impact speed and height of mass center, and decreases with the vertical stiffness. The sensitivity of wheel lift to impact speed is nonlinear. The relative sensitivities of wheel lift to height of mass center and vertical stiffness are 205% and −83%, respectively.
-
Key words:
- railway vehicle /
- collision /
- dynamics /
- overriding /
- sensitivity analysis
-
图 6 二系垂向刚度对车轮抬升量的影响
Figure 6. Influence of vertical stiffness of secondary suspensions on wheel lift
表 1 车辆模型的自由度
Table 1. Degrees of freedom of vehicle model
组件 自由度 组件数量/个 自由度数量/个 x z β 车体 有 有 有 1 3 构架 有 有 有 2 6 轮对 有 有 无 4 8 总计 7 17 
下载: 导出CSV
表 2 移动轨道模型的自由度
Table 2. Degrees of freedom of moving track model
组件 自由度 组件数量/个 自由度数量/个 x z β 钢轨 无 有 无 4 4 轨枕 无 有 无 4 4 总计 8 8
下载: 导出CSV
表 3 钩缓装置模型的参数
Table 3. Parameters of coupler model
变量 数值 变量 数值 端部 中间 端部 中间 δc/m 0.573 0.473 kul/（N•m−1） 1 × 104 1 × 104 f0/N 1 × 106 1 × 106 kr/（N•m−1） 1 × 108 1 × 108 al/m−1 50 20
下载: 导出CSV
表 4 可变参数
Table 4. Variable parameters
参数 大小 初值 105% 110% 120% 速度/（km•h−1） 20 21 22 24 质心高度/m 1.80 1.89 1.98 2.16 垂向刚度/（kN•m−1） 800 840 880 960
下载: 导出CSV
-
BAYKASOGLU C, SUNBULOGLU E, BOZDAG S E, et al. Railroad passenger car collision analysis and modifications for improved crashworthiness[J]. International Journal of Crashworthiness, 2011, 16(3): 319-329. doi: 10.1080/13588265.2011.566475 WANG W, HECHT M, ZHOU H, et al. A simulation method of crashworthy train set[C]//IEEE International Conference on Computer Science and Automation Engineering. Shanghai: IEEE, 2011: 297-301. XIE S, ZHOU H. Research on the crashworthy structures of subway vehicles[J]. International Journal of Crashworthiness, 2014, 19(6): 555-566. doi: 10.1080/13588265.2013.832471 KIRKPATRICK S W, SCHROEDER M, SIMONS J W. Evaluation of passenger rail vehicle crashworthiness[J]. International Journal of Crashworthiness, 2001, 6(1): 95-106. doi: 10.1533/cras.2001.0165 FANG H, SOLANKI K, HORSTEMEYER M F. Numerical simulations of multiple vehicle crashes and multidisciplinary crashworthiness optimization[J]. International Journal of Crashworthiness, 2005, 10(2): 161-171. doi: 10.1533/ijcr.2005.0335 LU G. Collision behaviour of crashworthy vehicles in rakes[J]. Proceedings of the Institution of Mechanical Engineers, Part F:Journal of Rail and Rapid Transit, 1999, 213(3): 143-160. doi: 10.1243/0954409991531100 LU G. Energy absorption requirement for crashworthy vehicles[J]. Proceedings of the Institution of Mechanical Engineers, Part F:Journal of Rail and Rapid Transit, 2002, 216(1): 31-39. doi: 10.1243/0954409021531665 DIAS J P, PEREIRA M S. Analysis and design for train crashworthiness using multibody models[J]. Vehicle System Dynamics, 2004, 40: 107-120. DIAS J P, PEREIRA M S. Optimization methods for crashworthiness design using multibody models[J]. Computers & Structures, 2004, 82(17/18/19): 1371-1380. SUN Y Q, COLE C, DHANASEKAR M, et al. Modelling and analysis of the crush zone of a typical Australian passenger train[J]. Vehicle System Dynamics, 2012, 50(7): 1137-1155. doi: 10.1080/00423114.2012.656658 卢毓江,肖守讷,朱涛,等. 列车纵向−垂向碰撞动力学耦合模型建模与研究[J]. 铁道学报,2014,36(12): 6-13. doi: 10.3969/j.issn.1001-8360.2014.12.002LU Yujiang, XIAO Shoune, ZHU Tao, et al. Construction of dynamic coupling model of longitudinal-vertical train crash[J]. Journal of the China Railway Society, 2014, 36(12): 6-13. doi: 10.3969/j.issn.1001-8360.2014.12.002 翟婉明. 车辆−轨道耦合动力学[M]. 4版. 北京: 科学出版社, 2015: 107-127. CHAAR N, BERG M. Simulation of vehicle-track interaction with flexible wheelsets, moving track models and field tests[J]. Vehicle System Dynamics, 2006, 44(S): 921-931. 杨超,肖守讷,朱涛. 非线性铁路车辆系统的时间积分算法[J]. 交通运输工程学报,2016,16(1): 88-94. doi: 10.3969/j.issn.1671-1637.2016.01.011YANG Chao, XIAO Shoune, ZHU Tao. Time integration algorithm in nonlinear railway vehicle system[J]. Journal of Traffic and Transportation Engineering, 2016, 16(1): 88-94. doi: 10.3969/j.issn.1671-1637.2016.01.011 吴庆,罗世辉,魏冲锋,等. 货运机车车钩缓冲装置动力学仿真模型[J]. 交通运输工程学报,2012,12(3): 37-43. doi: 10.3969/j.issn.1671-1637.2012.03.008WU Qing, LUO Shihui, WEI Chongfeng, et al. Dynamics simulation models of coupler systems for freight locomotive[J]. Journal of Traffic and Transportation Engineering, 2012, 12(3): 37-43. doi: 10.3969/j.issn.1671-1637.2012.03.008 European Commission. 2010 Railway applications —crashworthiness requirements for railway vehicle bodies: EN 15227: 2008 + A1[S]. Brussels: European Committee for Standardization, 2010. 杨超,肖守讷,阳光武,等. 一类非耗散的显式时间积分方法[J]. 振动工程学报,2015,28(3): 441-448.YANG Chao, XIAO Shoune, YANG Guangwu, et al. Non-dissipative explicit time integration methods of the same class[J]. Journal of Vibration Engineering, 2015, 28(3): 441-448. -
下载:
点击查看大图
图(8) / 表(4)
计量
- 文章访问数: 495
- HTML全文浏览量: 226
- PDF下载量: 17
- 被引次数: 0