Numerical Study on Wave-Induced Oscillatory Liquefaction in Anisotropic Seabed
-
摘要: 为探究各向异性海床在波浪作用下的瞬态液化问题,采用有限元法求解RANS (reynolds averaged navier-stokes)方程及k-ε湍流模型进行数值造波,通过求解Biot多孔弹性方程获得海床瞬态响应,进而建立了波浪-各向异性海床耦合作用的二维数值仿真模型. 在完成对新建模型的验证后,基于此模型系统地研究了波浪及海床特性对各向异性海床瞬态液化的影响. 研究结果表明:海床瞬态液化深度随波高、周期增大而增大,随海床饱和度增大而减小;当海床垂向渗透系数在一定范围内时,海床最大液化深度随垂向渗透系数增大而减小,超出该范围时,海床垂向渗透系数对海床最大液化深度的影响不明显;海床瞬态液化深度对水平方向渗透系数的改变不敏感.Abstract: In order to investigate the wave-induced oscillatory liquefaction in an anisotropic seabed, a two-dimensional numerical model for wave-seabed interactions is proposed. The reynolds-averaged navier-stokes (RANS) equation with the standard k-ε turbulence model was used to describe wave motions, and Biot’s poro-elastic equation was taken as the governing equation for the seabed model. After validation with previous experimental data and analytical solution, the proposed model was further applied to investigate the effects of wave and soil characteristics on anisotropic soil liquefaction. Numerical results show that the maximum liquefaction depth increased with an increase in wave height and wave period, and decreased with the degree of soil saturation. When the value of the soil permeability coefficient along the vertical direction is within a certain range, the effects of soil permeability along the vertical direction on the wave-induced soil response are obvious. Beyond the range, however, the effects of soil permeability along the vertical direction are almost negligible. Besides, the wave-induced oscillatory liquefaction depth in an anisotropic seabed is not sensitive to the variations in soil permeability along the horizontal direction.
-
Key words:
- liquefaction /
- RANS equation /
- Biot’s equation /
- seabed /
- anisotropy
-
汽车声学包是指具有降低车内声压尤其是空气声声压的声学部件组合,对车内声学特征分布具有重要的影响[1]. 前围声学包作为隔离动力总成噪声的主要结构件,是整个声学包的重要部件之一,其吸隔声性能的优劣直接影响到整车NVH (noise, vibration and harshness)性能水平[2]. 好的声学包设计不仅能够降低车内噪声水平,提升车内声学品质,而且能够促进车辆轻量化与降低成本. 因此,对汽车声学包性能提升进行研究具有重要的现实与工程意义.
早期由于技术手段限制(有限元等方法在中高频噪声计算准确性较低[3]),汽车声学包设计和开发主要基于实际样车与声学包部件,并通过试验与对标车进行比较. 随着统计能量分析(SEA)理论的发展并达到工程化[4],加上大型商业软件如VAONE的推广,使得车内中高频噪声的分析得到了一定的改善. 唐荣江等[5]针对车辆前围结构特点,依据有限元-统计能量分析(FE-SEA)模型建模原则,通过在表面创建声腔来确保能量在模型中的正确传递路径,其仿真结果与测试结果误差小于1.6 dB(A),验证了FE-SEA方法的有效性. Lee等[6]基于SEA方法建立了某纯电动汽车整车模型,分析了对车内噪声贡献较大的多个声学包子系统,并通过正交试验设计方法对其进行吸隔声优化,优化之后的驾驶员头部声腔声压级降低了1.1 dB(A). SEA方法在声学包工程设计中的有效性毋庸置疑,然而为构建SEA模型所需要的声学包材料参数(如孔隙率、黏性特征长度、热力特征长度等)和特性参数(如模态密度、内损耗因子、耦合损耗因子等)的准确值获取较难[7],导致基于SEA方法的仿真模型在简化与调参后仅能在部分特定工况与试验结果存在可比性,而在其他工况下模型置信度欠佳[8].
虽然准确的声学包材料参数和特性参数较难获得,但是声学包吸隔声性能及其组成部件的厚度和面积等参数就相对容易获取[9]. 在大数据与智能化的背景下,数据驱动为声学包的开发提供了更多的技术手段(如近似模型),可利用易获取的试验数据之间的模糊映射关系来代替声振动力学之间的精确推导关系[10],从而指导声学包的正向开发. 贺岩松等[11]以车辆声学包材料和厚度作为设计参数,驾驶员耳旁声压级、声学包总重量与价格作为设计目标,基于Kring方法建立了设计参数与目标的近似模型,其拟合准确度达到0.95,能够较好地指导声学包优化设计. 另外,Schaefer等[12]采用神经网络方法构建了前围声学包材料类型参数与成本和重量映射关系,在不降低原始吸隔声性能的条件下,通过粒子群算法对声学包进行了优化,优化后的声学包总重量降低了15.21%,同时,成本下降了9.7%. 然而,对于近似模型,其拟合与预测准确度可类比于仿真模型的建模与求解准确度,传统的浅层网络面对复杂问题拟合能力有限[13],因此,为了获得准确的声学包吸隔声性能预测结果,需要提升近似模型的预测性能. 深度信念网络(DBNs)作为深度学习的经典算法之一,以其优异的特征提取能力,在信息检索、图像识别等问题上获得很好的应用[14-15],因此,该方法可为声学包吸隔声性能预测提供借鉴.
由于汽车声学包匹配的非规则性以及噪声传递特性的复杂性,直接构建底层设计参数与声学包吸隔声性能的近似模型可能存在欠拟合的风险[16]. 声学包设计参数匹配与其整体隔声性能属于弱相关关系,而对与之相关的声包部件或路径的传递损失或插入损失关联度较高,若绕过声包部件或路径的吸隔声特性直接建立近似模型则会导致设计参数与目标间关联的复杂度剧增,从而影响预测精度. 因此,直接建立声学包设计参数与吸隔声性能的映射模型欠佳,究其原因在于没有考虑车辆声振传递特性与声学包正向开发流程[1]. 实际上,声学包性能对于驾乘人员来说是显性的,但是其成因却是隐性的,即声学包并不是一个完全意义上的独立结构,而是由众多子系统和零部件所组成[17]. 因此,对于前围声学包系统,其性能、重量与成本的多目标优化不能仅局限于声学包系统性能(上层)与设计参数(底层)两个层面,而需对隐藏于系统之下的子系统性能进行匹配分析. 因此,本文将从噪声传递关系与层级目标分解角度出发,提出前围声学包吸隔声性能、重量与成本多目标预测与优化方法,并将其应用于具体车型的前围声学包进行验证,可为今后声学包的分析与优化研究提供参考.
1. 研究方法
1.1 基于层级分解的前围声学包多目标优化模型
根据声学包噪声传递关系与正向开发流程,前围声学包系统可划分为系统级、子系统级与零部件级,据此可建立前围声学包多层级目标分解架构如图1所示. 其中,顶层设计目标为前围系统的吸隔声性能、重量和成本,而与之相连的相邻下层级设计变量为各子系统的性能指标(前围钣金传递损失、内前围隔音垫插入损失、外前围隔音垫插入损失、前风挡传递损失)与工艺指标(前围覆盖率、前围泄漏量),其中工艺指标与声学包性能、重量和成本都有关系[18],与子系统级相连的底层设计变量为零部件的材料组成、分块厚度及分块面积. 图1所示的多层级目标分解架构具备如下两个优点:1) 按照层级进行设计目标与设计变量的梯度分解有利于降低设计难度,并且符合声学包的开发流程;2) 中间层级(子系统层)的存在降低了整个优化模型的非线性度与复杂度,从而能够更容易地避开局部最优解. 另外,图1所示的多层级目标分解架构的层级之间并非全连接关系,这主要取决于层级之间的整体相关性与层级之内的局部协调性,反映在前围声学包正向设计流程与声振传递关系中,从而可实现各层级设计变量间的解耦或部分解耦,进一步降低了复杂问题的非线性,有利于提升分析与计算的准确度.
依据图1所示的分解架构,将其进行数值化建模,建立声学包多目标的预测模型与优化模型如下:
{min{y[L]k},k=1,2,⋯,K,L=1,2,⋯,L0,y[L]k=f[L]k(x[L + 1]1,x[L + 1]2,⋯,x[L+1]R),s.t.cm[L](x[L])=0,m=1,2,⋯,M,gn[L](x[L])⩽0,n=1,2,⋯,N,var.x[L+1]r∈X[L+1]r,r=1,2,⋯,R1,⋯,R2,⋯,R3,⋯,R,y[L]k∈Y[L]k, (1) 式中:L0为层级分解架构总层数;L为层级分解架构中的拟计算层级;
$ {y}_{k}^{[L]} $ 为相邻上层级设计目标;K为该层级设计目标个数;$ {x}_{r}^{[L+1]} $ 为相邻下层级的设计变量;R为该层级设计变量个数;$ {X}_{r}^{[L + 1]} $ 为第r个设计变量在第L + 1层的可行域区间;$ {Y}_{k}^{\left[L\right]} $ 为第k个设计变量在第L层的可行域区间;$ {f}_{k}^{[L]} $ 为预测模型,用以描述设计变量与设计目标间的映射关系,因此,$ {f}_{k}^{[L]} $ 模型的精准度对预测结果的准确性非常重要,对此本文提出SVR-DBNs (support vector regression- deep belief networks)方法作为前围声学包的预测模型,其具体计算过程将在下一小节进行介绍;$ {c}_{m}^{[L]} $ 和$ {g}_{n}^{[L]} $ 为约束条件.由于层级目标的分解,整个预测模型可看作前向传递的计算过程,而优化模型则可看作反向传递的求解过程,其中的多层级目标是与传统优化模型的不同之处. 基于该模型,即可对前围声学包的性能、重量和成本进行多目标优化设计.
1.2 SVR-DBNs方法提出
1.2.1 DBNs模型
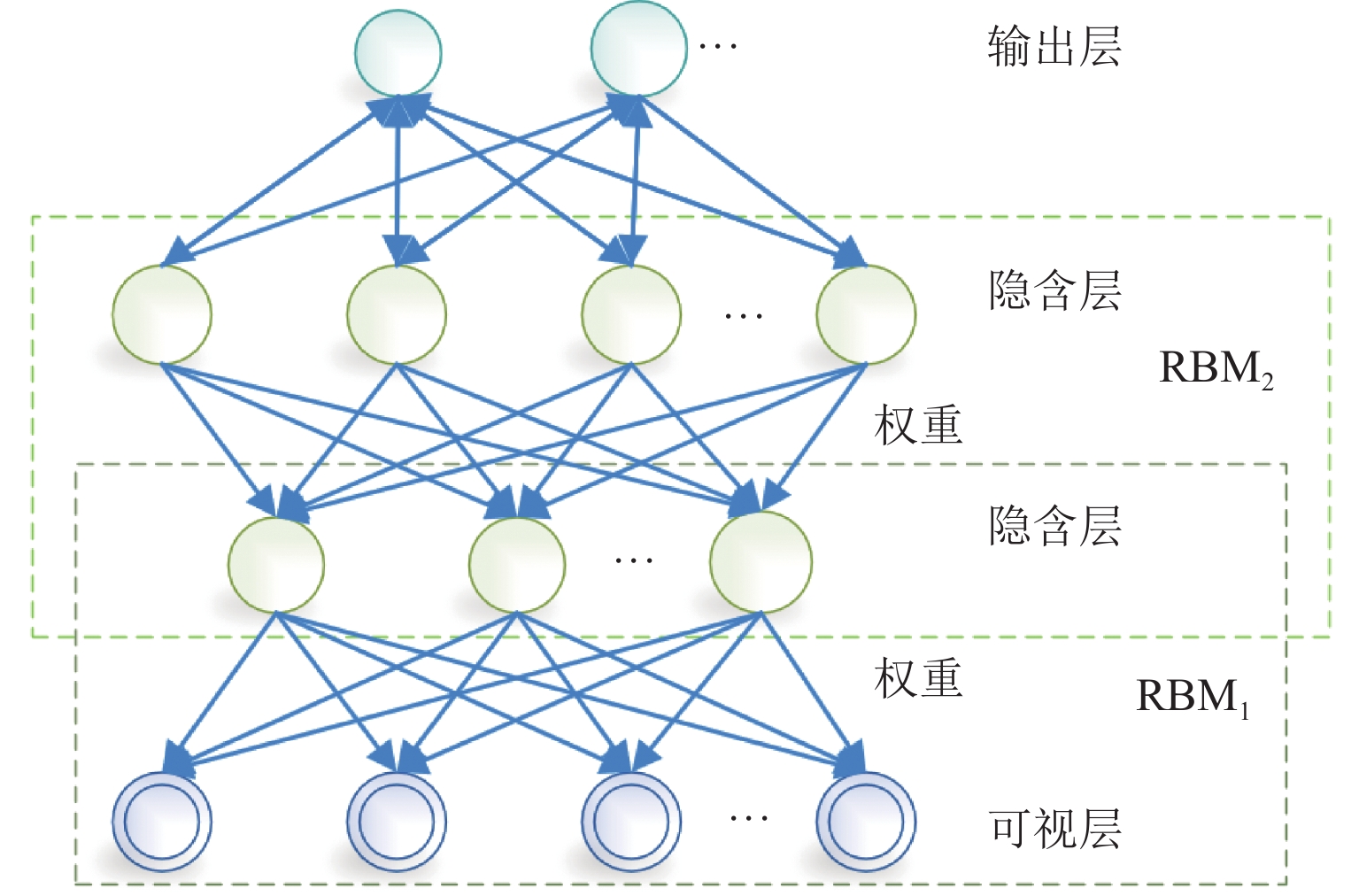
DBNs是由多个受限玻尔兹曼机(RBM)堆叠而成的网络结构[19],其核心是采用逐层贪婪学习算法去优化网络的连接权重与偏置,从而提取数据特征同时进行模式识别,DBNs的拓扑结构如图2所示. RBM为DBNs的重要组成单元[20],是一种特殊类型的马尔可夫随机场,由一层二元随机可见单元(通常是Bernoulli或Gauss)v∈{0,1}v和一层二元随机隐藏单元(通常是Bernoulli)h∈{0,1}h组成. RBM不同层内单元之间的连接受到限制,即所有可见节点都连接到隐藏节点,而可见-可见或隐藏-隐藏节点内没有连接.
在RBM模型中,不同层中各单元间的权重和偏置概率分布通过能量函数来定义. 对于Bernoulli-Bernoulli RBM,能量函数为
E(v,h;θ)=−V∑i=1H∑j=1wijvihj−V∑i=1aivi−H∑j=1bjhj, (2) 式中:θ=(wij, ai, bj)为模型参数;vi和hj分别为可见单元i和隐藏单元j输入参数;wij为vi和hj之间的权重;ai 和 bj 为偏置;V和H分别为可见和隐藏单元的数量.
给定能量函数和模型参数,可见单元和隐藏单元的联合分布为
p(v,h;θ)=exp(−E(v,h;θ))∑v∑hexp(−E(v,h;θ)). (3) 因为可见-可见或隐藏-隐藏单元连接不存在,可见单元vi和隐藏单元hj的概率是独立的. 因此,条件分布p(h|v)和p(v|h)可以通过式(4)和式(5)进行计算.
p(hj=1|v;θ)=σ(V∑i=1wijvi+bj), (4) p(vi=1|h;θ)=σ(H∑j=1wijhj+ai), (5) 式中:
$\sigma \left(x\right)={1 + \mathrm{e}\mathrm{x}\mathrm{p}\;x}^{-1}$ .RBM 模型参数θ可以通过最速梯度下降或共轭梯度下降来估计,以最大化训练数据的似然概率. wij、ai和bj的更新规则为
{△wij=ε(⟨vihj⟩data−⟨vihj⟩model),△ai=ε(⟨vi⟩data−⟨vi⟩model),△bj=ε(⟨hj⟩data−⟨hj⟩model), (6) 式中:ε为学习率,范围为0~1;
$\langle {{\text{•}}}\rangle_{{\rm{data}}} $ 为对训练数据的期望;$\langle {{\text{•}}}\rangle_{{\rm{model}}} $ 为对模型定义分布的相同期望,实际中通常利用比对散度的方法来求解[21].此外,在模型训练中为了提升计算效率,还可采用分批训练的方式[22].
DBNs由多个RBM堆积而成, 其底层将接收实值数据,第一个 RBM 模型是Gauss-Bernoulli RBM,其余的 RBM 模型是Bernoulli-Bernoulli RBM. DBNs的学习过程包括预训练和微调两个阶段. 在预训练阶段,DBNs将自下而上逐层贪婪地训练. 每个RBM 都是单独训练的,层之间的权重和层的偏差是固定的并保存以供进一步分析. 此过程可以表示为
p(hj(l−1)=1|h(l);θ(l))=σ(V∑i=1w(l)ijv(l)i+bj(l−1)), (7) 式中: l 为层数,底层单元的状态为h(0).
根据式(6),Gauss-Bernoulli RBM也有类似的形式. 此学习过程将一直持续至最后一个隐藏层. 预训练阶段简化了深度架构的优化过程,并允许使用多参数来提高特征融合能力防止过度拟合. 在微调阶段,通过反向传播算法使用标记的输入数据对DBNs模型的权重和偏差将进行更新.
1.2.2 SVR-DBNs改进模型
常规的DBNs 模型是将线性回归作为顶层结构,所提取的特征与目标非线性较强的情况下可能导致预测效果欠佳. 因此,在本文中,提出了一种新的DBN回归模型SVR-DBNs,该模型使用支持向量回归 (SVR) 层代替DBN结构顶部的线性回归层,提升了模型应对强非线性问题的处理能力. SVR-DBNs以与一般 DBNs相同的方式执行预训练阶段和微调阶段. 不同之处在于,较低层的权重和偏差为通过反向传播来自顶部SVR层的梯度来学习,因此,应该根据倒数第二层的激活来区分SVR目标,故SVR-DBNs可适应维度更高、非线性更复杂的问题计算. 在实践中,L2-SVR 被广泛用于解决回归问题,若令目标为 f (w)并用倒数第二个激活h替换输入x;那么,L2-SVR的反向传播误差为
∂f(w)∂hn|k=2=w−2Cwtn(max(1−wThntn,0)), (8) 式中:w为SVR超平面的法向量;
$ {{\boldsymbol{h}}}_{n} $ 为第n个隐含层单元;$ C > 0\mathrm{为} $ 常数,控制样本满足约束的程度;tn∈{−1, + 1}.反向传播算法的学习过程与一般的DBNs相同. 图3为SVR-DBNs的训练过程,其中
$ ,{e}_{\mathrm{m}\mathrm{a}\mathrm{x}}\mathrm{为} $ 人为设置的误差迭代最大值,例如10−6;l0为设置的迭代停止数目.1.3 基础数据获取
测试结果能够真实反映前围声学包的吸隔声效果,本文所用数据在长安汽车研究院的混响室-半消声室内进行收集,如图4所示,测试方法参考国际汽车工程师学会标准SAE J1400—2017[23],激励声源为总能量120 dB的白噪声. 为收集构建近似模型所需样本数,采用拉丁超立方试验设计[11]构造样本组合,其中设计因素有前围钣金、外前围隔音垫和内前围隔音垫的厚度与对应的厚度面积占比. 根据工程经验,厚度设计水平上(下)限为原始方案数值分别上(下)浮动10%(20%),不同厚度对应面积占比设计水平为原始方案百分比分别增加(减少)10%(20%),同时有不同厚度面积占比总和为100%约束的限制,覆盖率设计水平为99.3%~99.7%,间隔0.1%,泄漏量设计水平为0.10%~0.30%,间隔0.05%,表1所示为汽车前围声学包原始方案. 通过拉丁超立方试验设计,生成了45组测试样本,外加1组原始状态,总共有46组样本. 测试中,依据标准SAE J1400—2017分别获取前围系统传递损失、前围钣金传递损失、外前围插入损失和内前围插入损失,其中部件孔洞由密封件进行密封处理.
表 1 前围声学包系统及零部件原始状态Table 1. Original state of firewall acoustic package system部件名称 材料名称 厚度/
mm面积
占比/%密度/
(kg·m−3)单价/
(元·m−3)前围钣金 高强度钢 ① 1.0 ② 30 7820 39100 ③ 1.2 ④ 70 外前围
隔音垫玻璃纤维 ⑤ 10.0 ⑥ 20 100 5800 ⑦ 20.0 ⑧ 80 内前围
隔音垫PU 泡沫 ⑨ 5.0 ⑩ 35 64 7200 ⑪ 10.0 ⑫ 65 棉毡 ⑬ 3.0 ⑭ 20 113 4000 ⑮ 5.0 ⑯ 80 前围覆盖率 ⑰ 99.5% ±0.1%, ±25 元 前围泄漏量 ⑱ 0.20% ±0.05%, ±35 元 注:①~⑱表示构建预测模型的设计变量. 2. 研究结果与讨论
2.1 基于SVR-DBNs的声学包预测结果
通过试验测试获取了准确的前围声学包系统与零部件传递损失与插入损失结果,在此基础上引入提出的SVR-DBNs方法,并结合多层级目标分解架构,建立有效适应于工程应用的声学包正向开发模型. 对于吸隔声性能目标,在构建第三层级至第二层级模型时,将零部件厚度与厚度对应的面积占比作为输入,将子系统传递损失或插入损失作为输出,为方便计算,选择传递损失和插入损失的1/3倍频点综合总值MTL (mean transmission loss)作为目标. 构建第二层级至第一层级模型时,将零部件的MTL、覆盖率和泄漏量作为输入,前围声学包系统的MTL作为输出. 对于重量和成本目标的建模与预测也是类似的流程. 在测试结果中随机选取35组样本作训练集,剩余11组做测试集进行学习,并取10次运行结果的平均值作为评价指标以降低随机误差. 对于多层级目标分解架构中的相邻层级,选择4层的SVR-DBNs网络(即含有2层隐含层)作为子模型进行建模,其中各子模型的输入层节点数为输入数据维度,隐含层节点数目通过网格寻优的方法在2与输入层维度范围内确定,输出层为基于高斯核函数的SVR. 建模所用软件为MATLAB R2020a,配置Intel 1165G7 CPU与16G内存,GPU为Intel Iris Xe Graphics with 96 EUs.
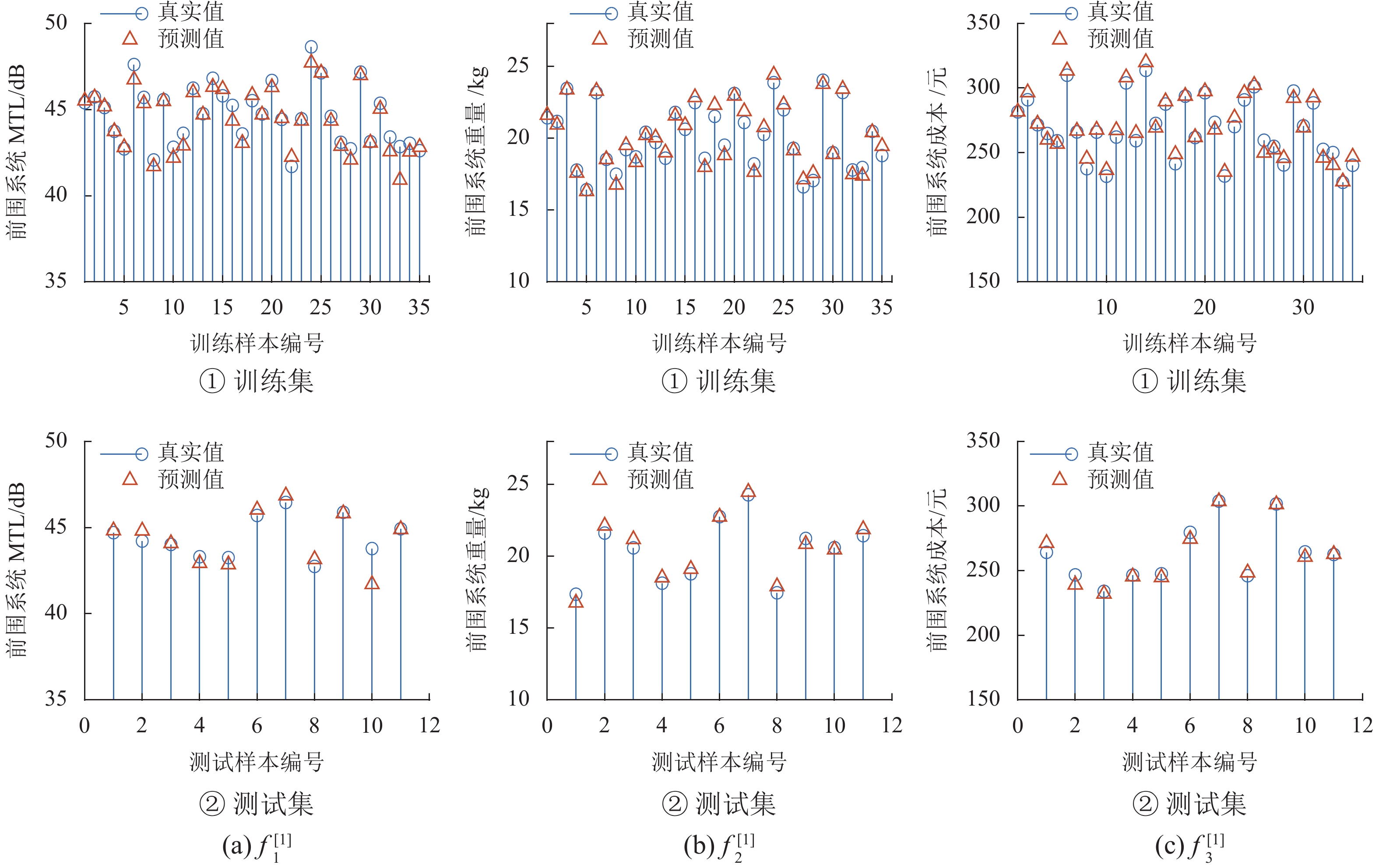
经过网络学习,图5所示为模型
$ {f}_{1}^{\left[1\right]} $ ~$ {f}_{3}^{\left[1\right]} $ 在训练集与测试集中的计算结果,其中,$ {f}_{1}^{\left[1\right]} $ ~$ {f}_{3}^{\left[1\right]} $ 模型相对误差最大值在各自的训练集与测试集中分别为$ {f}_{1}^{\left[1\right]} $ 的4.52%和$ {f}_{1}^{\left[1\right]} $ 的4.74%.表2统计了多层级模型中各子模型在测试集中的准确度,采用决定系数R2来评价. 由表2可知:第一层级模型的R2均高于0.975,其中
$ {f}_{3}^{\left[1\right]} $ 的决定系数值达到0.986,同时第二层级模型的R2均不低于0.970,表明所提的SVR-DBNs方法在前围声学包预测中的有效性;由于重量与成本目标相对于MTL性能目标具有较好的线性度,所以重量和成本模型的拟合精度要高于MTL性能模型. 实际上,成本和重量在不考虑复杂因素如人工成本、加工成本等的条件下还可以直接使用线性累加模型,但为了所提方法的泛化性,本文仍选取SVR-DBNs建立重量和成本的预测模型.表 2 多层级分解模型在测试集上的准确度Table 2. Accuracy of multi-level model on test set目标 第一~二层级(R2) 第二~三层级(R2) MTL 0.975 0.970、0.974、0.968 重量 0.983 0.979、0.984、0.981 成本 0.986 0.988、0.978、0.980 为验证SVR-DBNs的优越性,将常用的DBNs、SVR与BPNN模型应用于多层级目标分解架构并进行建模预测,其中DBNs的结构参数与SVR-DBNs一致但顶层采用线性回归模型,SVR采用高斯核函数并通过网格参数寻优,反向传播神经网络(BPNN)经过交叉验证择优选择模型结构,并通过遗传算法进行参数初始化. 表3所示为4种模型对前围声学包MTL、重量和成本的预测结果比较,可见:BPNN在测试集中的准确性相对最低,其R2在MTL、重量和成本上分别为0.917、0.924和0.931,同时均方误差(MSE)分别为0.771、1.267和13.457;SVR和DBNs的准确性较高,其中,DBNs的R2在MTL、重量和成本上分别为0.971、0.979和0.974,同时MSE分别为0.341,0.611和7.491. 对比可知,SVR-DBNs方法拥有最高的R2和最低的MSE,验证了所提方法的优越性.
表 3 声学包多目标优化结果Table 3. Multi-goals optimization results of acoustic package预测模型 MTL 重量 成本 R2 MSE/dB R2 MSE/kg R2 MSE/元 BPNN 0.917 0.771 0.924 1.267 0.931 13.457 SVR 0.955 0.364 0.959 0.846 0.961 7.950 DBNs 0.971 0.341 0.979 0.611 0.974 7.491 SVR-DBNs 0.975 0.296 0.983 0.579 0.986 4.360 2.2 优化结果与验证
基于前围声学包多层级目标分解架构与SVR-DBNs模型,对前围声学包的MTL、重量和成本进行多目标优化. 为了方便对多目标进行综合评价,引入“加权目标”,考虑到各个目标的重要程度不同,需设置不同的权重系数. 由于前围声学包主要目的是吸隔声,所以认为其MTL相较成本与重量更为重要,因此,拟定MTL、重量与成本的权重系数分别为0.50、0.25和0.25. 设计变量为前围系统各零部件的分块厚度与不同厚度的面积占比,根据工程可行性,设计空间为变量在原始值基础上±20%的区间范围,约束条件有两项: 前围声学包最大厚度不大于其在原始状态的最大厚度;各零部件不同厚度对应的面积占比之和为100%,所建立的优化模型如式(9)所示. 遗传算法有收敛速度快、计算准确等优点,因此采用遗传算法求解式(9),其中遗传算法参数设置为种群大小200,最大遗传代数500,交叉概率0.7,变异概率0.01,代沟0.95. 优化的参数结果如表4所示,根据优化参数得到的多目标预测结果与实测结果如表5所示.
表 4 前围声学包多目标优化设计参数Table 4. Multi-goals optimization design parameters of firewall acoustic package部件名称 材料名称 厚度/mm 面积占比/% 前围钣金 高强度钢 1.0 32 1.2 68 外前围隔音垫 玻璃纤维 14.0 28 20.0 72 内前围隔音垫 PU 泡沫 6.0 37 10.0 63 棉毡 4.0 28 5.0 72 前围覆盖率/% 99.6 前围泄露量/% 0.20 表 5 前围声学包多目标优化结果与实测结果Table 5. Multi-goals optimization results and measured results of firewall acoustic package状态 MTL/dB 重量/kg 成本/元 加权目标 原始状态 45.4 21.4 281.6 1.0247 优化后 预测值 48.6 19.3 267.3 0.9588 实测值 47.9 19.5 269.2 0.9693 {minY=0.4(1/y[1]1)/ξ1+0.3y[1]2/ξ2+0.3y[1]3/ξ3,y[1]k1=f[1]k1(x[2]1,x[2]2,⋯,x[2]R2),k1=1,2,3,R2=1,2,⋯,11,y[2]k2=f[2]k2(x[3]1,x[3]2,⋯,x[3]R3),y[2]k2=x[2]k2,k2=1,2,⋯,9,R3=1,2,⋯,16,s.t.H=∑R3=1,3,5,7max{x[3]R3,x[3]R3+1}⩽36.2,sj=x[3]R3+x[3]R3+1=100%,R3=9,11,13,15,j=(R3−7)/2,var.x[2]R2∈[0.8x[2]R1,1.2x[2]R1],x[3]R3∈[0.8x[3]R3,1.2x[3]R3], (9) 式中:
$ {\xi }_{1}、{\xi }_{2} $ 与$ {\xi }_{3} $ 分别为设计目标$ {y}_{1}^{\left[1\right]}、 $ $ {y}_{2}^{\left[1\right]} $ 与$ {y}_{3}^{\left[1\right]} $ 的归一化常数,一般取数据集目标的统计均值;$ {x}_{{R}_{3}}^{\left[3\right]} $ ,$ {R}_{3}\in \{1, 2,\cdots ,8\} $ 时为厚度参数,$ {R}_{3}\in \left\{9, 10,\cdots ,16\right\} $ 时为面积占比参数,由于MTL需要越大越好,因此优化时取$ {y}_{1}^{\left[1\right]} $ 的倒数,其余参数同式(1).根据表5可知:多层级架构模型优化的前围声学包加权目标为0.9588(MTL、重量和成本分别为48.6 dB、19.3 kg与267.3元),较原始状态的加权目标1.0247(MTL、重量和成本分别为45.4 dB、21.4 kg与281.6元)提升了6.44%(MTL、重量和成本分别改进了7.05%、9.94%与5.07%).
为了验证优化实际效果,对前围声学包按照优化状态进行了调整,并在混响室-半消声室内进行了验证,实测加权目标为0.9693(MTL、重量和成本分别为47.9 dB、19.5 kg与268.2元),预测结果与实测结果接近,两者相对误差为1.09%(MTL、重量和成本相对误差绝对值分别为1.44%、1.04%与0.71%),验证了所提方法的准确性与有效性.
3. 结 论
本文提出了SVR-DBNs方法对汽车前围声学包吸隔声性能、重量和成本多目标进行研究,并从噪声传递关系与层级目标分解角度出发,提出声学包多层级目标预测与优化方法,并在实际车型上进行了验证. 所提的SVR-DBNs方法对前围声学包性能、重量与成本目标预测准确度均在0.975以上,优于传统的BPNN、SVR与DBNs模型. 此外,研究中构建了前围声学包多层级架构模型,降低了整个优化模型的非线性度与复杂度,能够更容易地避开局部最优解. 基于该模型的优化结果与实测结果接近,两者加权目标相对误差为1.09%(MTL、重量和成本相对误差绝对值分别为1.44%、1.04%与0.71%),优化后的实测结果较前围声学包原始状态性能、重量和成本分别提升了5.51%、9.01%与4.40%,验证了所提方法的有效性与准确性.
致谢:感谢西南交通大学基础研究培育支持计划资助项目(2682021ZTPY068)、科技创新项目(2682022CX008,XJ2021KJZK054)的支持.
-
表 1 数值案例所取参数
Table 1. Parameters used in numerical examples
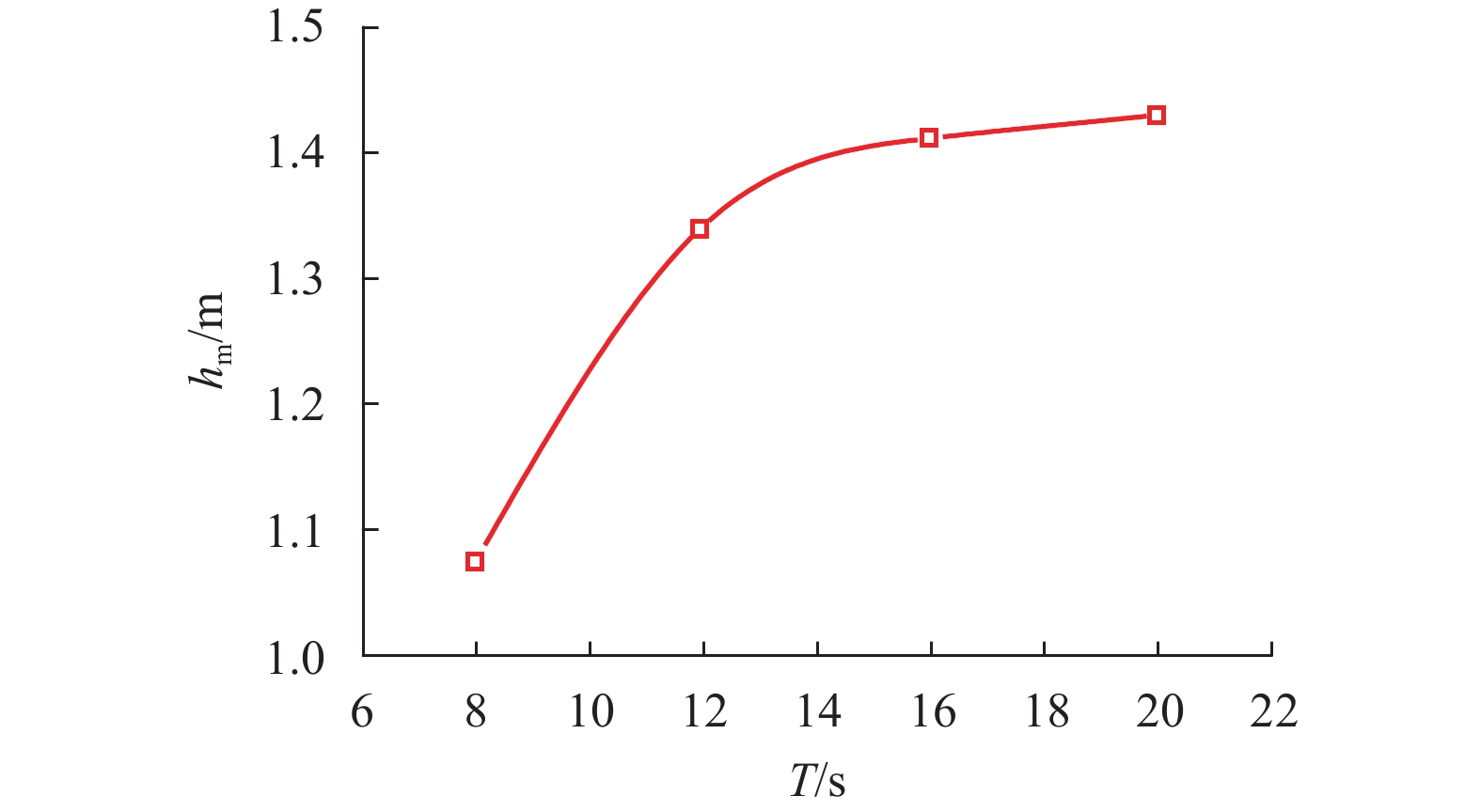
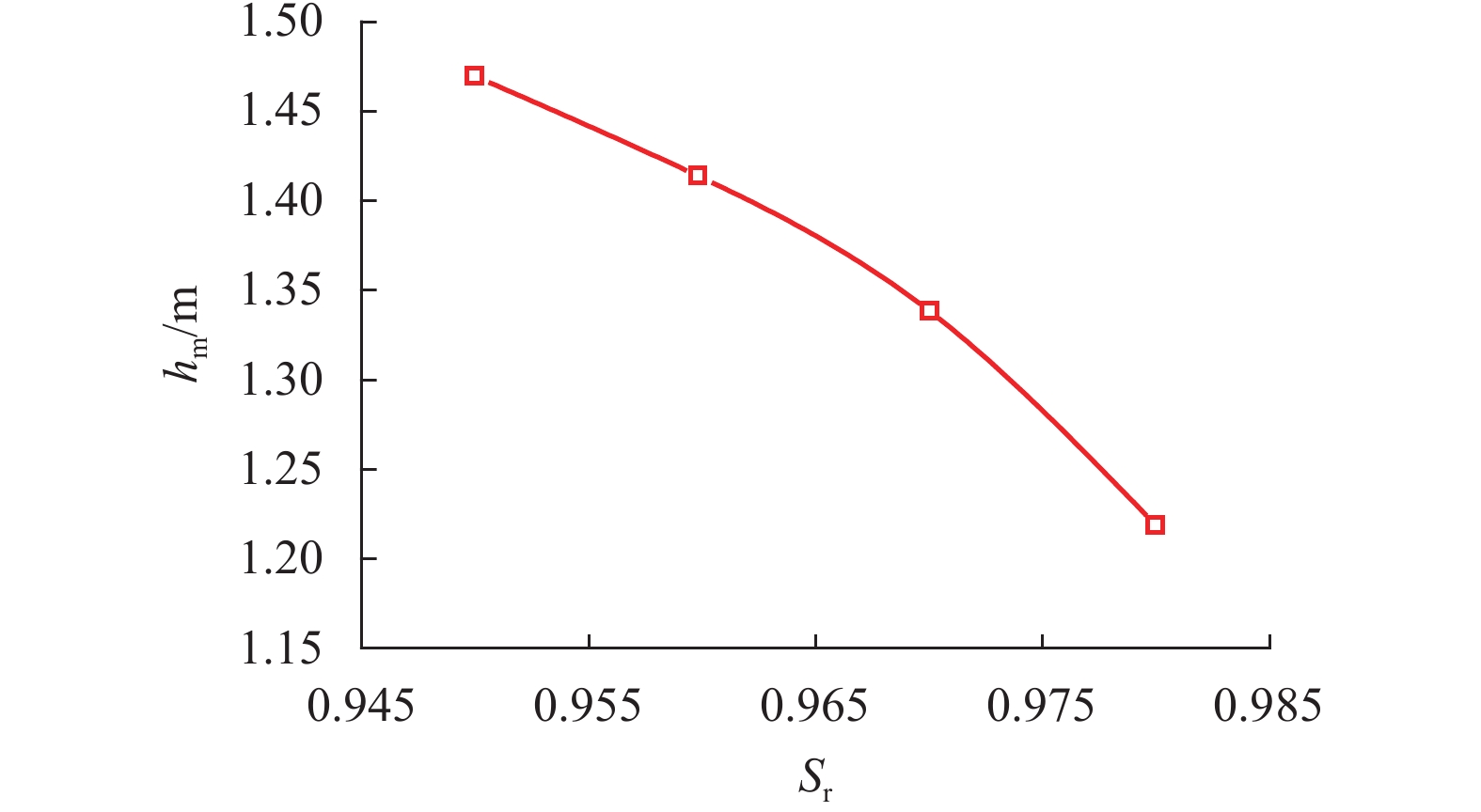
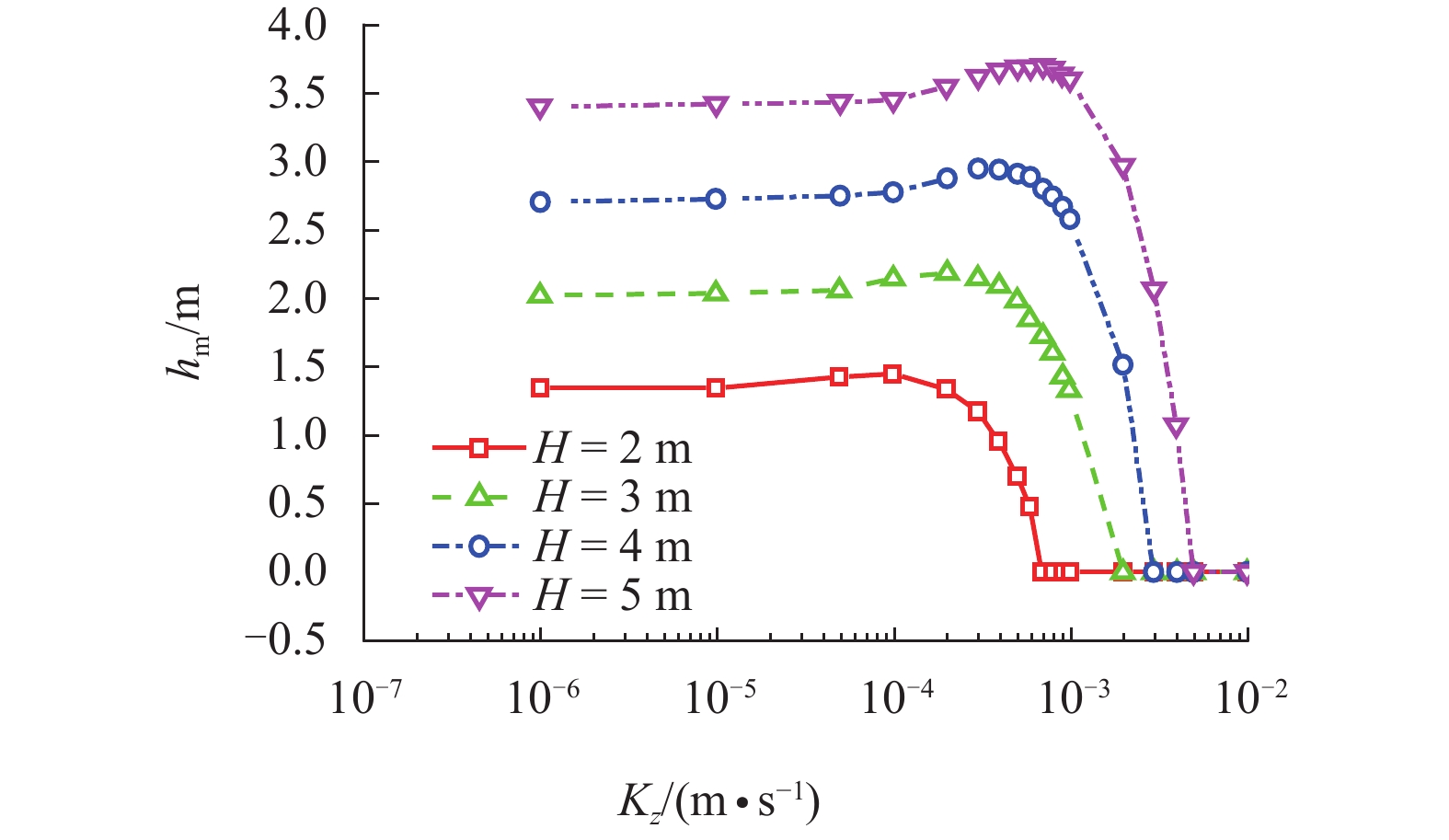
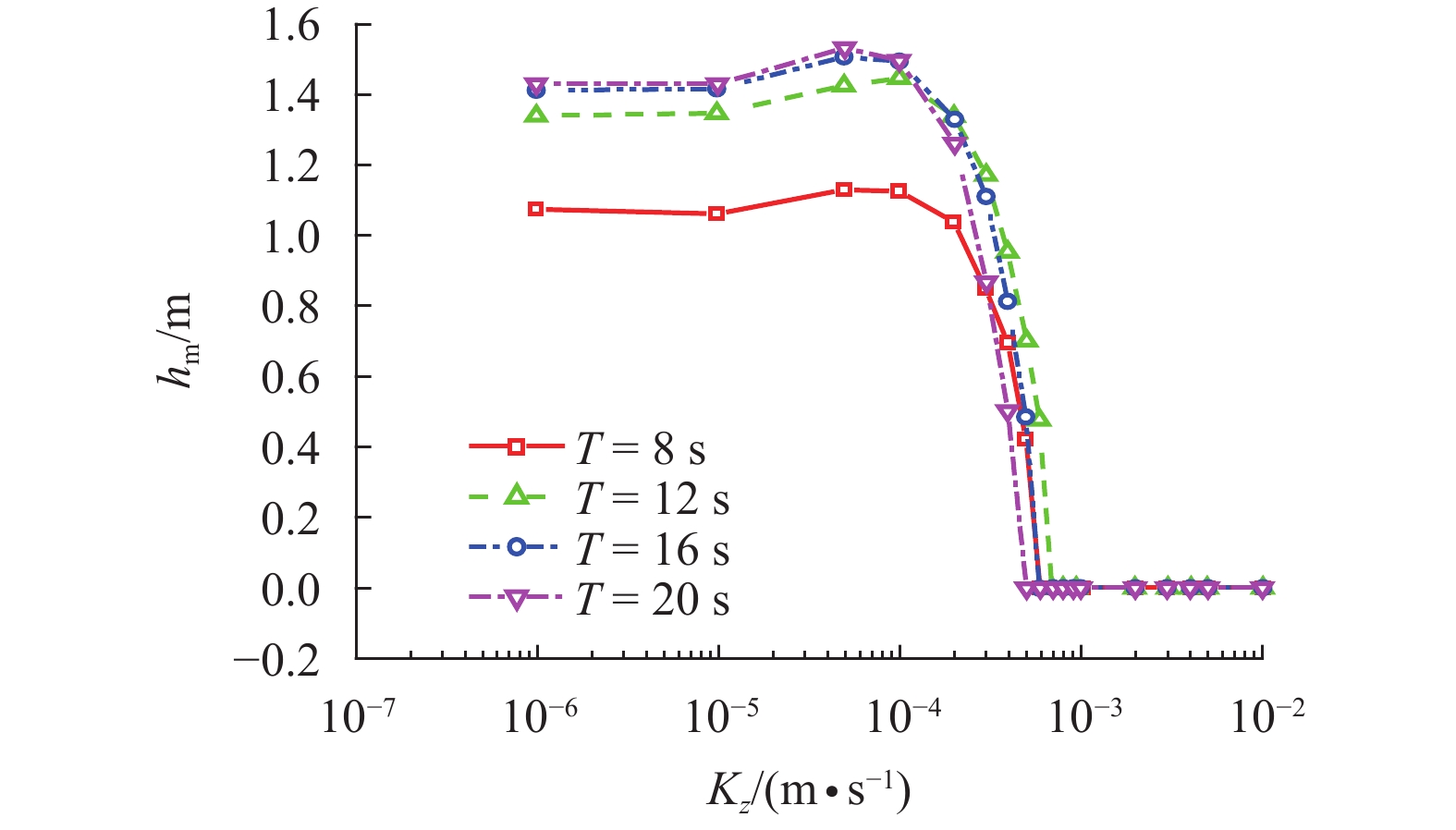
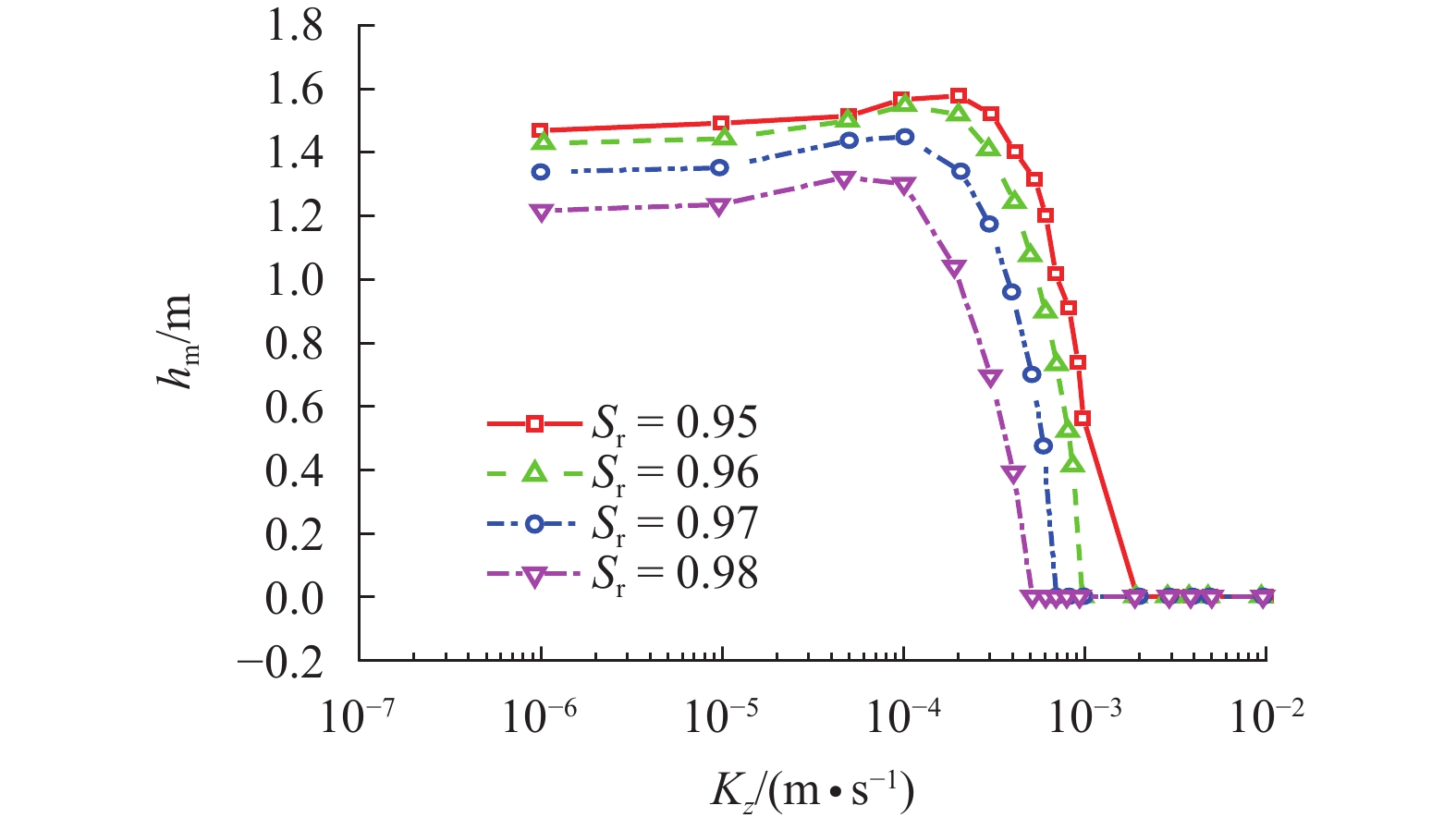
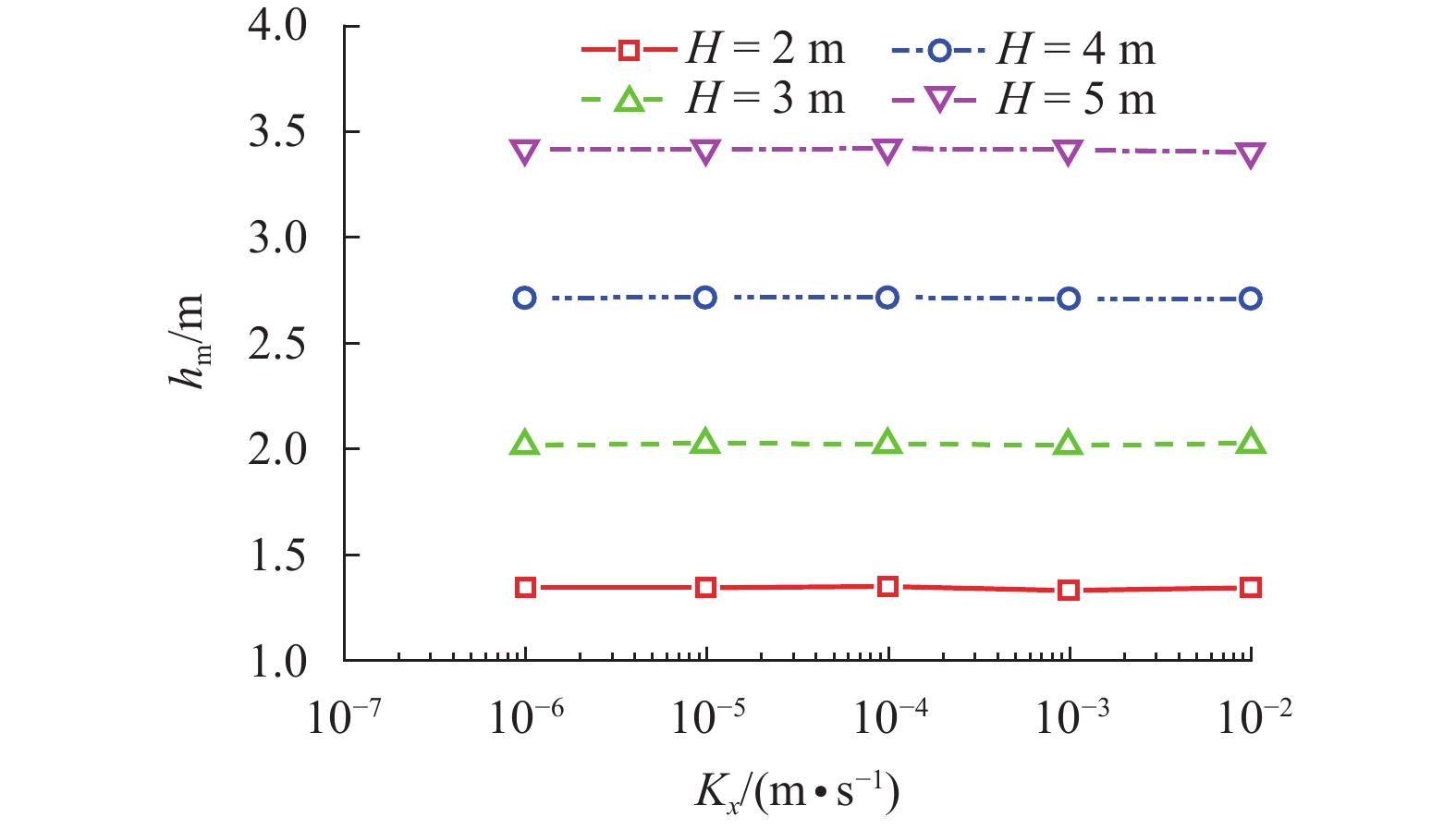
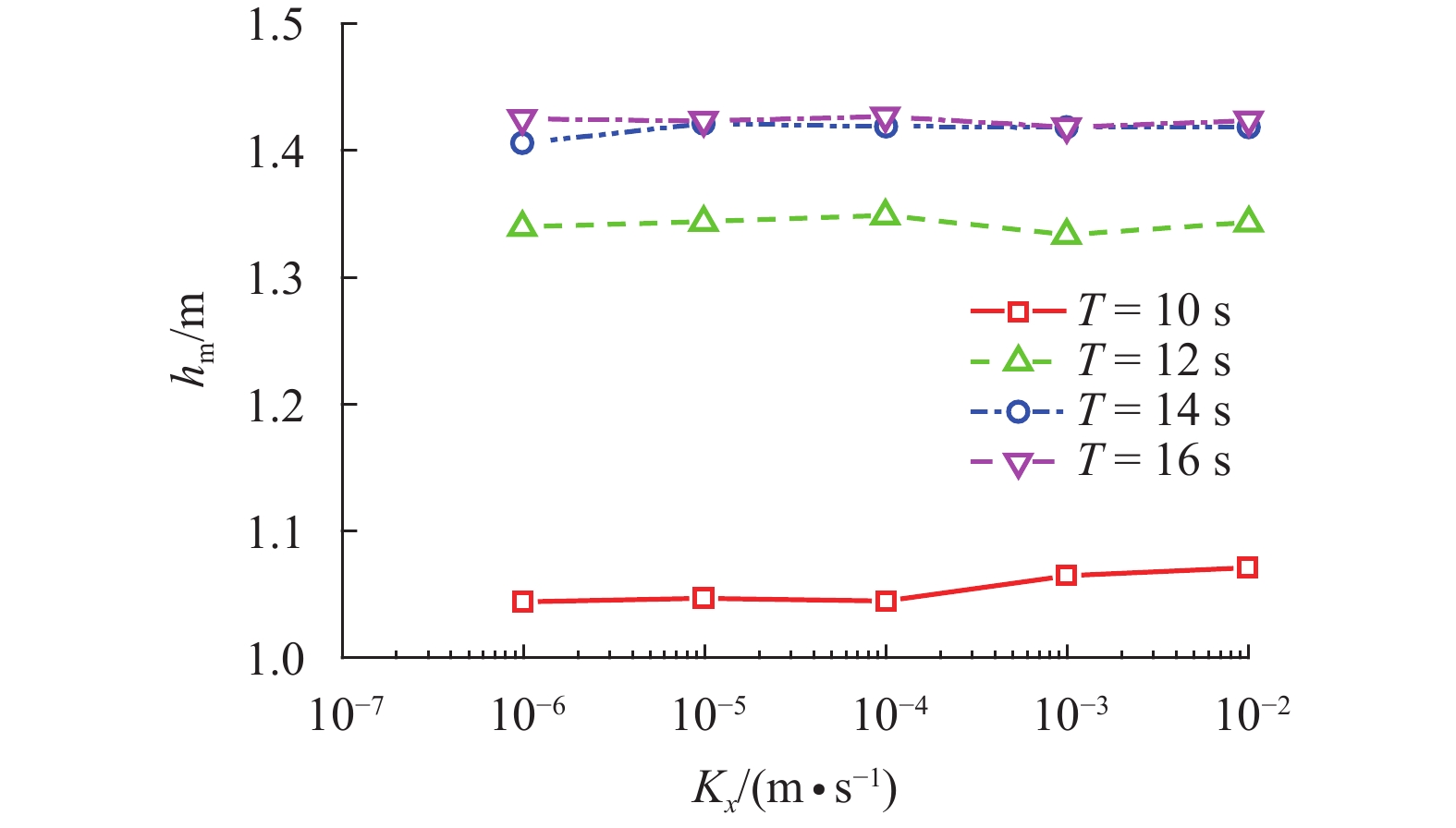
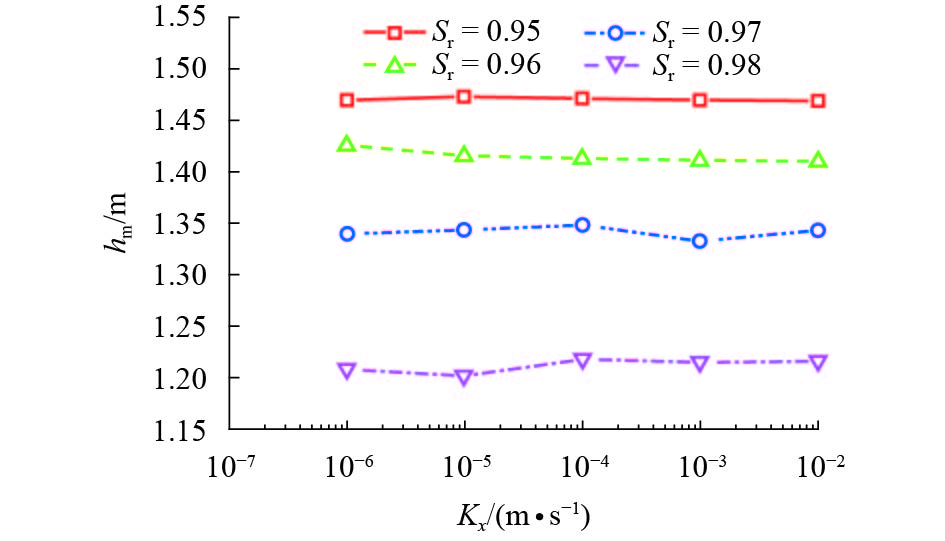
类型 参数 数值 波浪 H/m 2 d/m 10 T/s 12 h/m 20 海床土体 n 0.4 Kx/(m•s–2) 1 × 10–7 Kz/(m•s–1) 1 × 10–6 Sr 0.97 μs/(N•m–2) 1 × 107 -
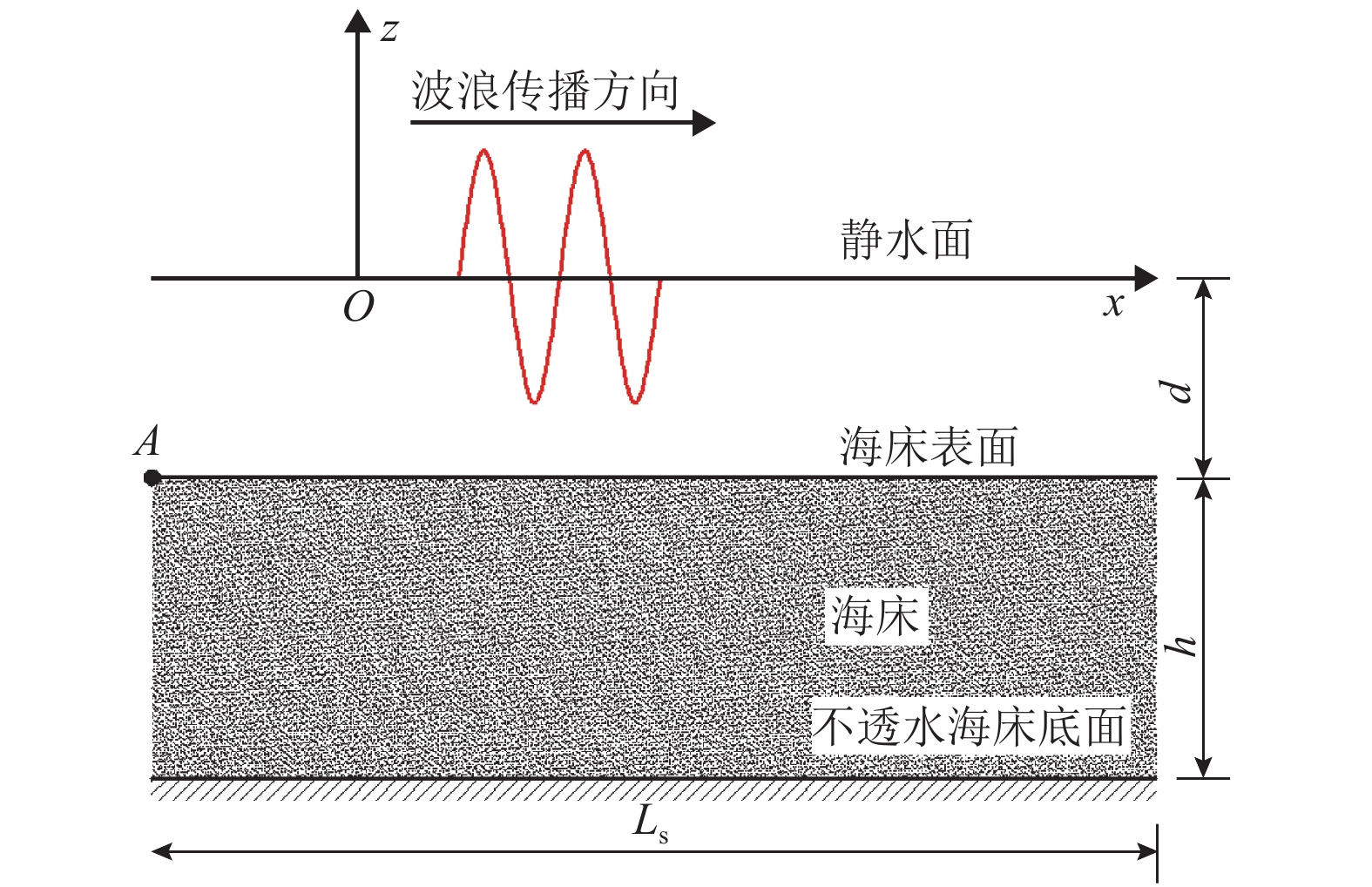
ZHANG J S, JENG D S, LIU P L F, et al. Response of a porous seabed to water waves over permeable submerged breakwaters with bragg rection[J]. Ocean Engineering, 2012, 43: 1-12. doi: 10.1016/j.oceaneng.2012.01.024 ZHANG J S, ZHANG Y, ZHANG C, et al. Numerical modeling of seabed response to the combined wave-current loading[J]. Journal of Offshore Mechanics and Arctic Engineering, 2013, 135(3): 031102. YE J H, JENG D S. Response of seabed to natural loadings-waves and currents[J]. Journal of Engineering Mechanics, 2012, 138(6): 601-613. doi: 10.1061/(ASCE)EM.1943-7889.0000356 YE J H, JENG D S, WANG R, et al. Validation of a 2D semi-coupled numerical model for fluid-structure-seabed interaction[J]. Journal of Fluids and Structures, 2013, 42: 333-357. doi: 10.1016/j.jfluidstructs.2013.04.008 SUMER B M, TRUELSEN C, FREDSØE J. Liquefaction around pipelines under waves[J]. Journal of Waterway,Port,Coastal,and Ocean Engineering, 2006, 132: 266-275. 段伦良,郑东生,张启博,等. 半埋式海底管道周围海床瞬态液化稳定性研究[J]. 西南交通大学学报,2017,52(4): 671-677.DUAN Lunliang, ZHENG Dongsheng, ZHANG Qibo, et al. Numerical tudy on wave-induced oscillatory soil liquefaction around a partially buried pipeline[J]. Journal of Southwest Jiaotong University, 2017, 52(4): 671-677. 段伦良,王少华,张启博,等. 三维水流作用下哑铃型围堰周围海床局部冲刷[J]. 西南交通大学学报,2018,53(4): 704-711.DUAN Lunliang, WANG Shaohua, ZHANG Qibo, et al. 3D current-induced local scour around dumbbell-shaped steel suspending cofferdams[J]. Journal of Southwest Jiaotong University, 2018, 53(4): 704-711. BIOT M A. General theory of three-dimensional consolidation[J]. Journal of Applied Physics, 1941, 12: 155-164. doi: 10.1063/1.1712886 MADSEN O S. Wave-induced pore pressures and effective stress in a porous bed[J]. Geotechnique, 1978, 28(4): 377-393. doi: 10.1680/geot.1978.28.4.377 YAMAMOTO T, SELLMEIHER H L, HIJUM E V. On the response of a porous-elastic bed to water waves[J]. Journal of Fluid Mechanics, 1978, 87(1): 193-206. doi: 10.1017/S0022112078003006 HSU J R C, JENG D S. Wave-induced soil response in an unsaturated anisotropic seabed of finite thickness[J]. International Journal for Numerical and Analytical Methods in Geomechanics, 1994, 18(11): 785-807. doi: 10.1002/(ISSN)1096-9853 ZEN K, YAMAZAKI H. Mechanism of wave-induced liquefaction and densification in seabed[J]. Soils and Foundations, 1990, 30(4): 90-104. doi: 10.3208/sandf1972.30.4_90 SAKAI T, HANTANAKA K, MASE H. Wave-induced effective stress in seabed and its momentary liquefaction[J]. Journal of Waterway,Port,Coastal,and Ocean Engineering, 1992, 118(2): 202-206. JENG D S, ZHAO H Y. Two-dimensional model for accumulation of pore pressure in marine sediments[J]. Journal of Waterway,Port,Coastal,and Ocean Engineering, 2015, 14(3): 1-12. 黄光爵,郑永来,武伯弢. 波浪作用下可液化海床最大液化深度[J]. 地震工程与工程振动,2012,32(5): 146-151.HUANG Guangjue, ZHENG Yonglai, WU Botao. The maximum liquefaction depth of liquefiable seabed under wave loading[J]. Journal of Earthquake Engineering and Engineering Vibration, 2012, 32(5): 146-151. SUZUKI K, TAKAHASHI S. Liquefaction of loosely deposited sandbed behind a breakwater due to wave overtopping[C]//Coastal Structures 2003. Portland: American Society of Civil Engineers, 2003: 656-662 LIU B, JENG D S, YE G L et al. Laboratory study for pore pressures in sandy deposit under wave loading[J]. Ocean Engineering, 2015, 106: 207-219. doi: 10.1016/j.oceaneng.2015.06.029 张金凤,张庆河,秦崇仁. 波浪作用下非均质各向异性海床响应的数值模拟[J]. 天津大学学报,2006,39(2): 159-164. doi: 10.3969/j.issn.0493-2137.2006.02.007ZHANG Jinfeng, ZHANG Qinghe, QIN Chongren. Numerical simulation of wave-induced response of inhomogeneous and anisotropic seabed[J]. Journal of Tianjin University, 2006, 39(2): 159-164. doi: 10.3969/j.issn.0493-2137.2006.02.007 YU H, ZENG X, LI B, et al. Effect of fabric anisotropy on liquefaction of sand[J]. Journal of Geotechnical and Geoenvironmental Engineering, 2013, 139(5): 765-774. doi: 10.1061/(ASCE)GT.1943-5606.0000807 ZHOU X L, WANG J H, ZHANG J, et al. Wave and current induced seabed response around a submarine pipeline in an anisotropic seabed[J]. Ocean Engineering, 2014, 75: 112-127. doi: 10.1016/j.oceaneng.2013.11.016 ZHAO H Y, JENG D S, LIAO C C. Effects of cross-anisotropic soil behaviour on the wave-induced residual liquefaction in the vicinity of pipeline buried in elasto-plastic seabed foundations[J]. Soil Dynamic and Earthquake Engineering, 2016, 80: 40-55. doi: 10.1016/j.soildyn.2015.10.004 LIN P Z, LIU P L F. Internal wave-maker for Navier-Stokes equations models[J]. Journal of Waterway,Port,Coastal,and Ocean Engineering, 1999, 125(4): 207-214. 期刊类型引用(1)
1. 张琪,周香莲,叶冠林. 波浪荷载引起不同埋深管线周围海床响应和液化分析. 上海交通大学学报. 2021(05): 489-496 .  百度学术
百度学术其他类型引用(4)
-






 下载:
下载:
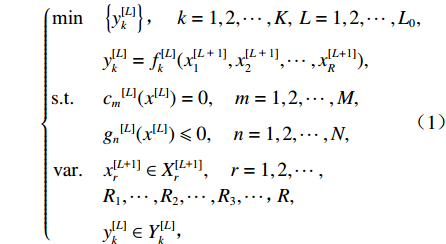
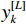
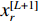
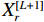
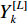
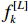

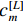
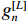

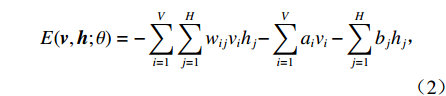
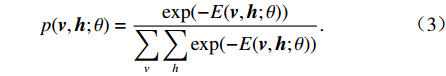
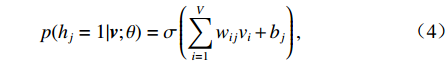

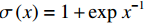
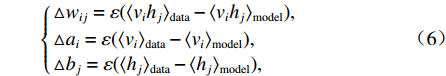
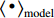
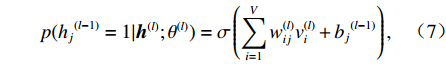
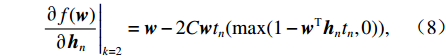
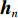
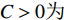


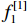

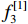
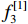

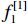
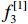
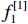

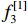
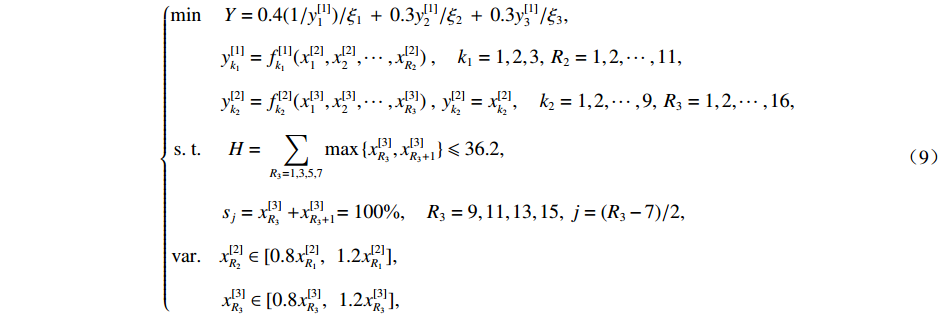
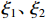
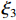
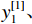
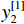
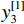
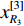
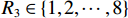
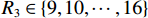
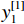
 下载:
下载:

 百度学术
百度学术