Influence of Porous Seabed Characteristics on Wave Forces Acting on Monopile
-
摘要: 为研究多孔介质海床的波浪衰减作用及单桩所受波浪力的变化规律，采用修正RANS方程和Forchheimer饱和阻力模型控制多孔介质内部流体流动，运用流体体积法追踪自由液面，建立波浪-多孔介质海床-单桩相互作用三维数值分析模型. 首先，基于波浪与多孔介质海床相互作用过程，研究了多孔介质海床对波浪传播的衰减作用；其次，分析了相同波浪条件下多孔介质海床及刚性海床时单桩所受波浪力的数值变化，突出了考虑海床多孔特性的必要性；最后，采用单一变量控制法，进一步研究了单桩所受波浪力数值随海床多孔特性参数的变化规律. 研究结果表明，海床多孔特性对波浪传播具有明显的衰减作用；给定的波浪参数和多孔介质海床条件下，单桩所受波浪力最大值比刚性海床情况提高约35%，若忽略海床多孔特性结构物会因低估波浪力数值而造成安全隐患；另外，结构物所受波浪力与海床孔隙率、颗粒直径、海床厚度及双层海床分布厚度及每层孔隙率密切相关. 其中，波浪力最大值随着海床颗粒直径的增加而递减，随着孔隙率的增大先增加后降低，且孔隙率会影响波浪力随海床厚度变化的趋势.Abstract: This study investigated the wave attenuation and wave forces acting on the monopile due to porosity characteristics of the seabed. A three-dimensional numerical analysis model of wave-porous seabed-monopile-interactions is established, in which the modified Reynolds-averaged Navier-Stokes (RANS) equation and Forchheimer saturation drag model are used to control porous flow, while the fluid volume method (VOF) is used to track free surface. Based on the proposed model, wave attenuation induced by porous seabed is first studied, taking into consideration the interaction between waves and porous seabed. Second, the numerical variation of the wave forces acting on the monopile under the same wave action with porous seabed or rigid seabed is analysed, highlighting the necessity of considering the porous characteristics of the seabed. Finally, the influence of the seabed porous characteristics on the numerical variation of the wave forces is further studied using the single variable control method. The numerical results show that the porous characteristics of the seabed have obvious attenuation effect on wave propagation. Additionally, under the given wave parameters and the characteristics of the porous seabed, the maximum wave forces acting on the monopile is about 35% higher than that of the rigid seabed. This suggests that ignoring the porous characteristics of seabed will result in an underestimation of the wave forces and could cause security risks. In addition, the wave forces acting on the structure are closely related to the porous characteristics of the seabed. The results show that the maximum wave forces decrease as the particle size increases, while increasing first and then decreasing with an increase of porosity. One thing to note is that porosity affects the variation trend of wave forces acting on monopile changing with seabed thickness.
-
Key words:
- porous seabed /
- porosity /
- particle size /
- wave forces /
- numerical analysis
-
图 2 波浪-多孔介质海床-单桩相互作用三维示意
Figure 2. 3D sketch of wave-porous seabed-monopile- interactions
图 7 波浪沿多孔介质海床衰减数值结果与相应试验结果对比
Figure 7. Comparison of numerical results for wave dampingalong the porous seabed with the correspondingexperimental results
图 9 Fx，max随海床孔隙率的变化曲线
Figure 9. Variation curve of maximum wave force inthe x axis with seabed porosity
图 11 Fx，max随多孔介质海床颗粒直径的变化曲线
Figure 11. Variation curve of maximum wave force in the x axis with partical size
图 12 不同海床孔隙率条件下Fx，max随海床相对厚度的变化曲线
Figure 12. Variation curves of maximum wave force inthe x axis with seabed relative thickness with different seabed porosity
-
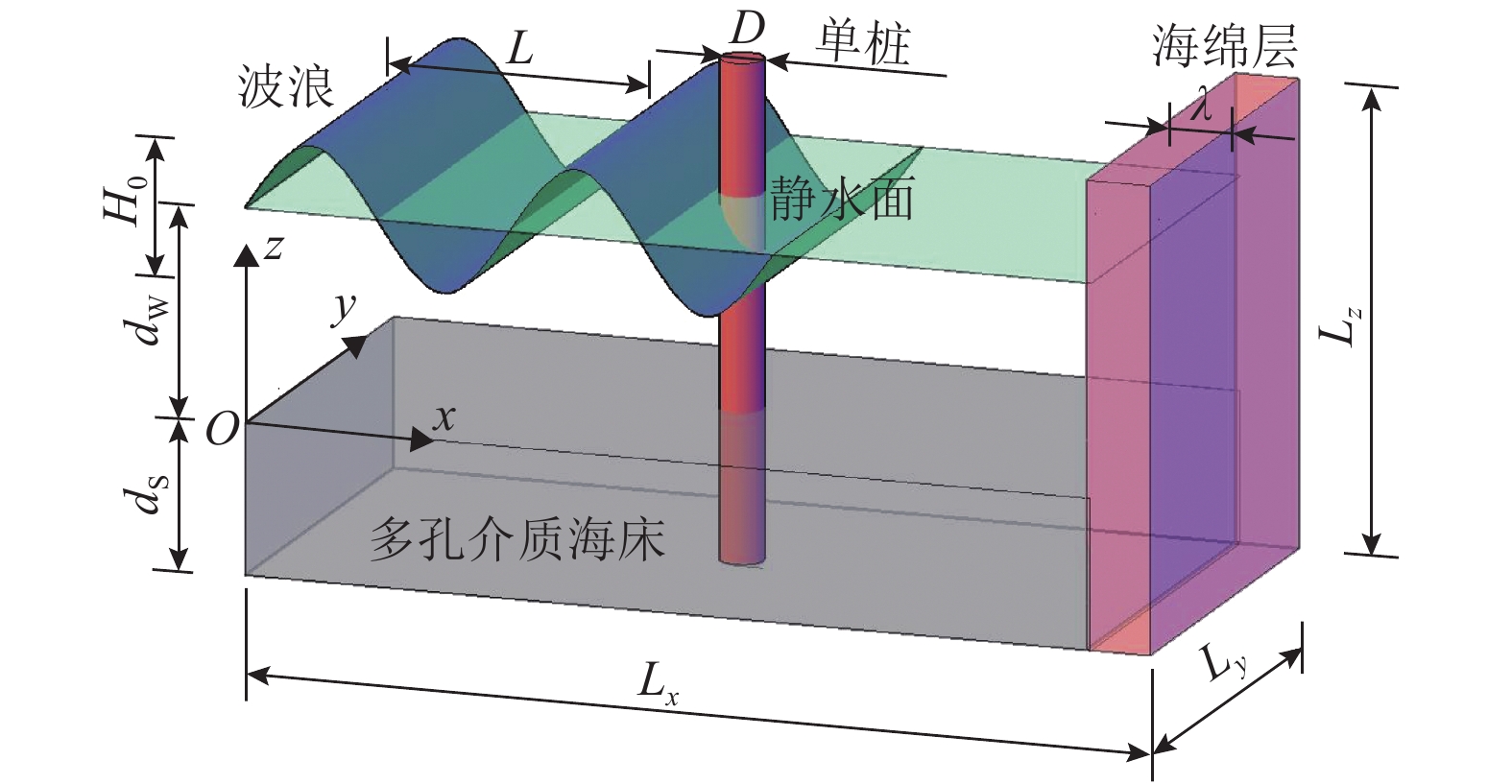
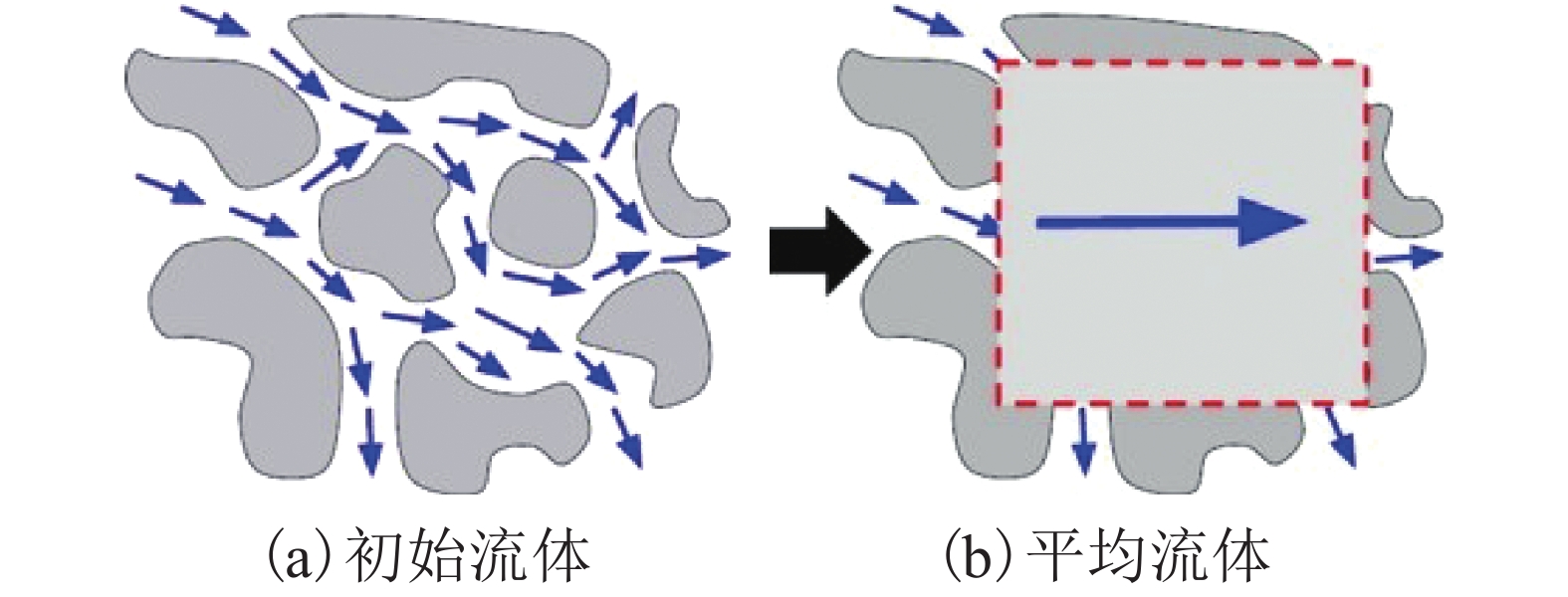
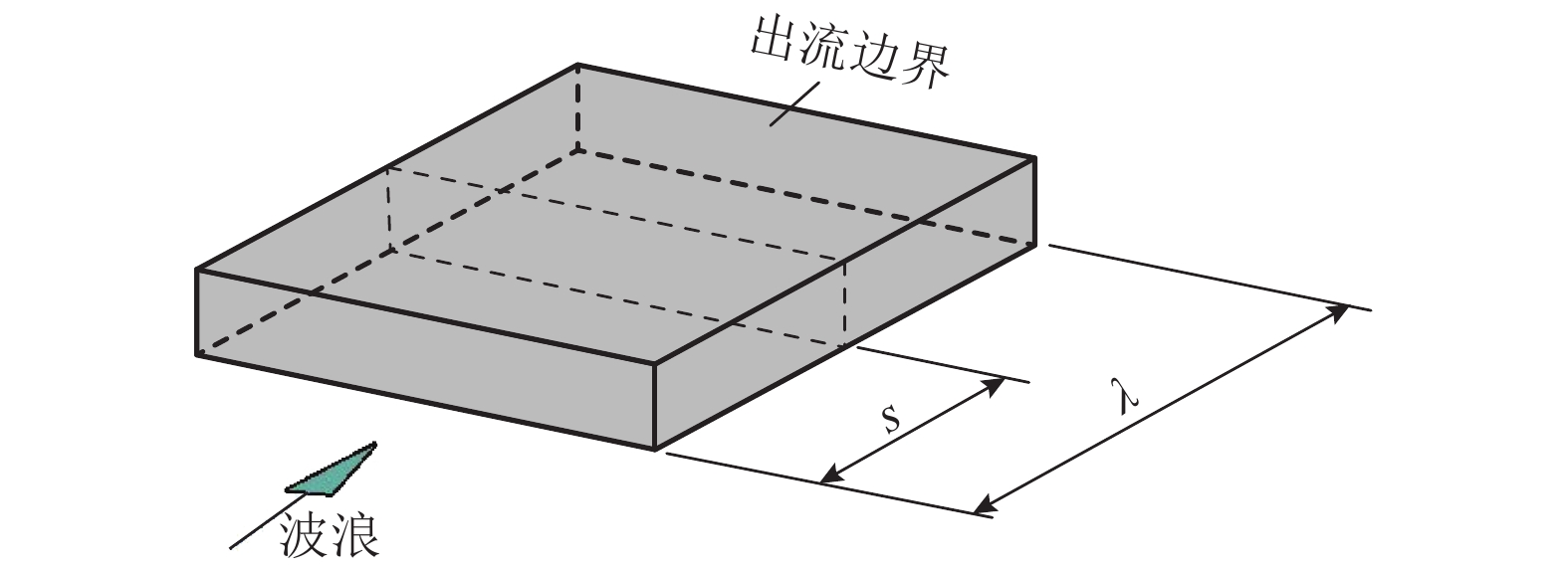
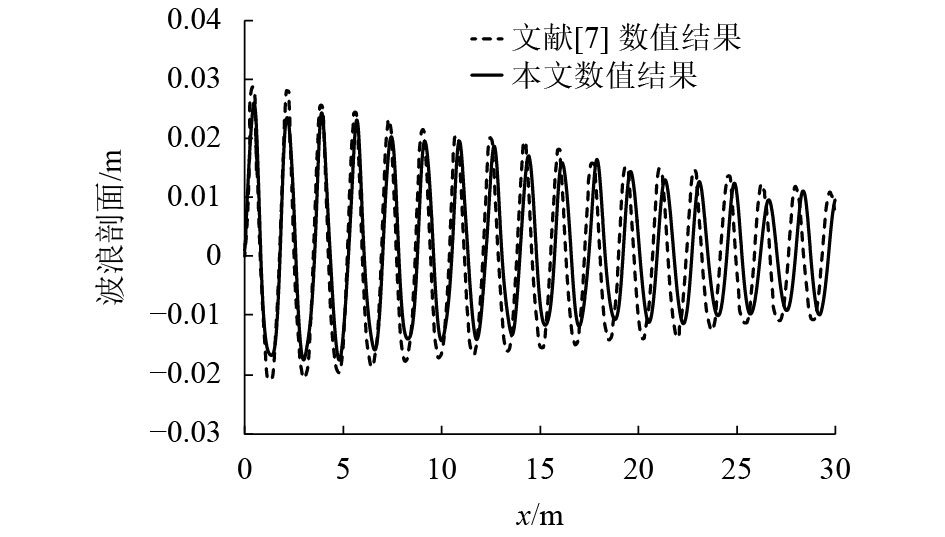
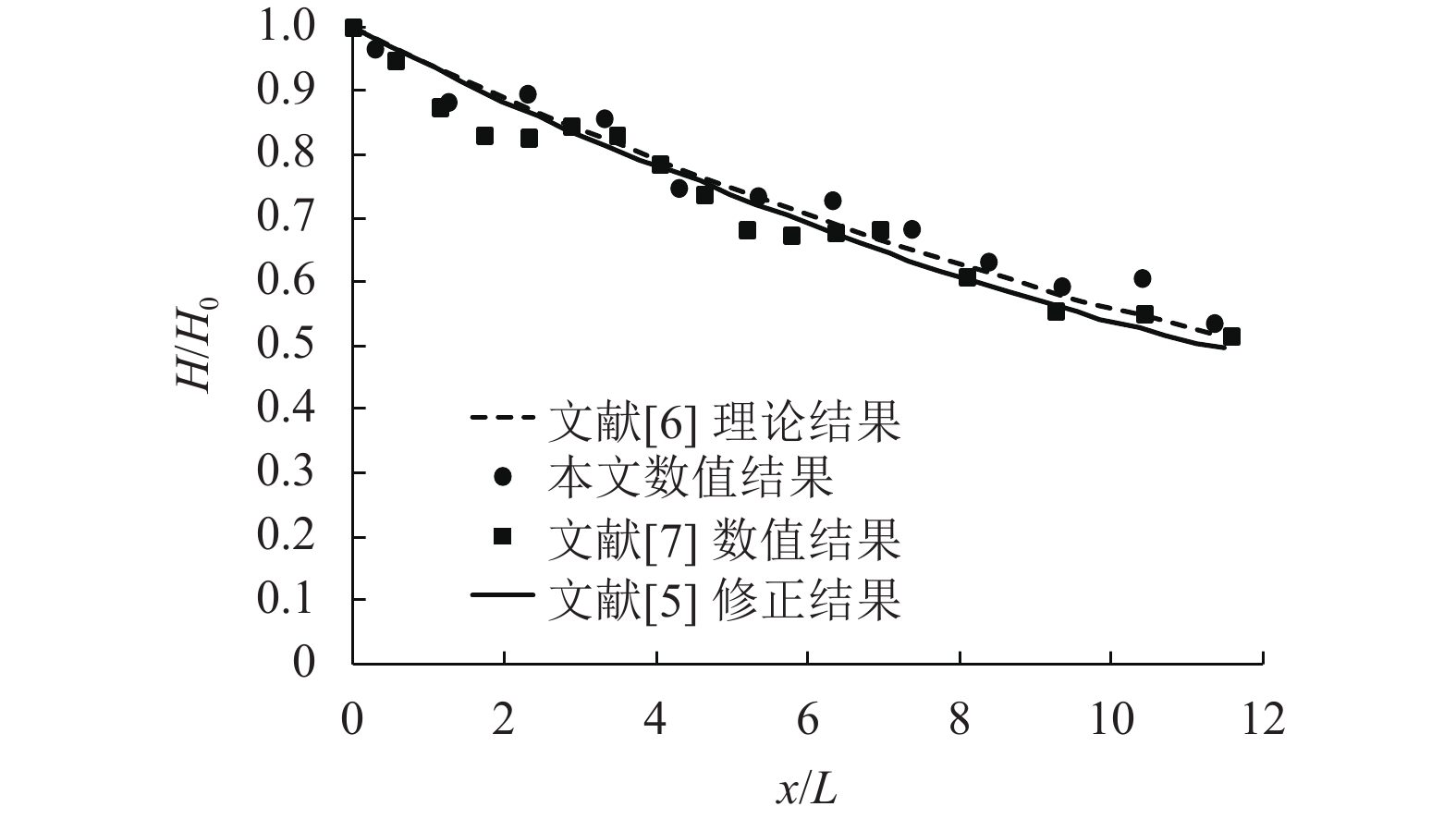
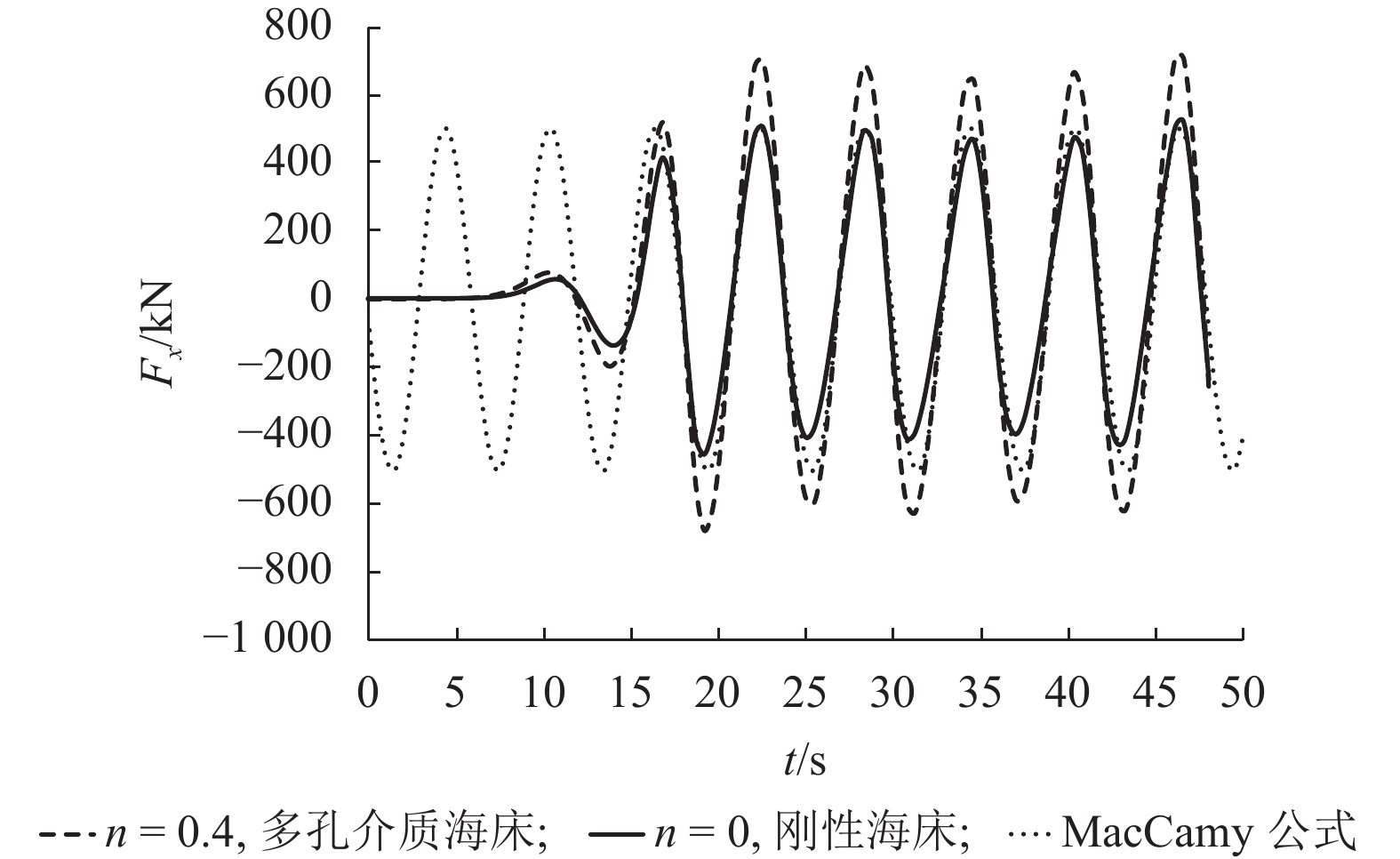
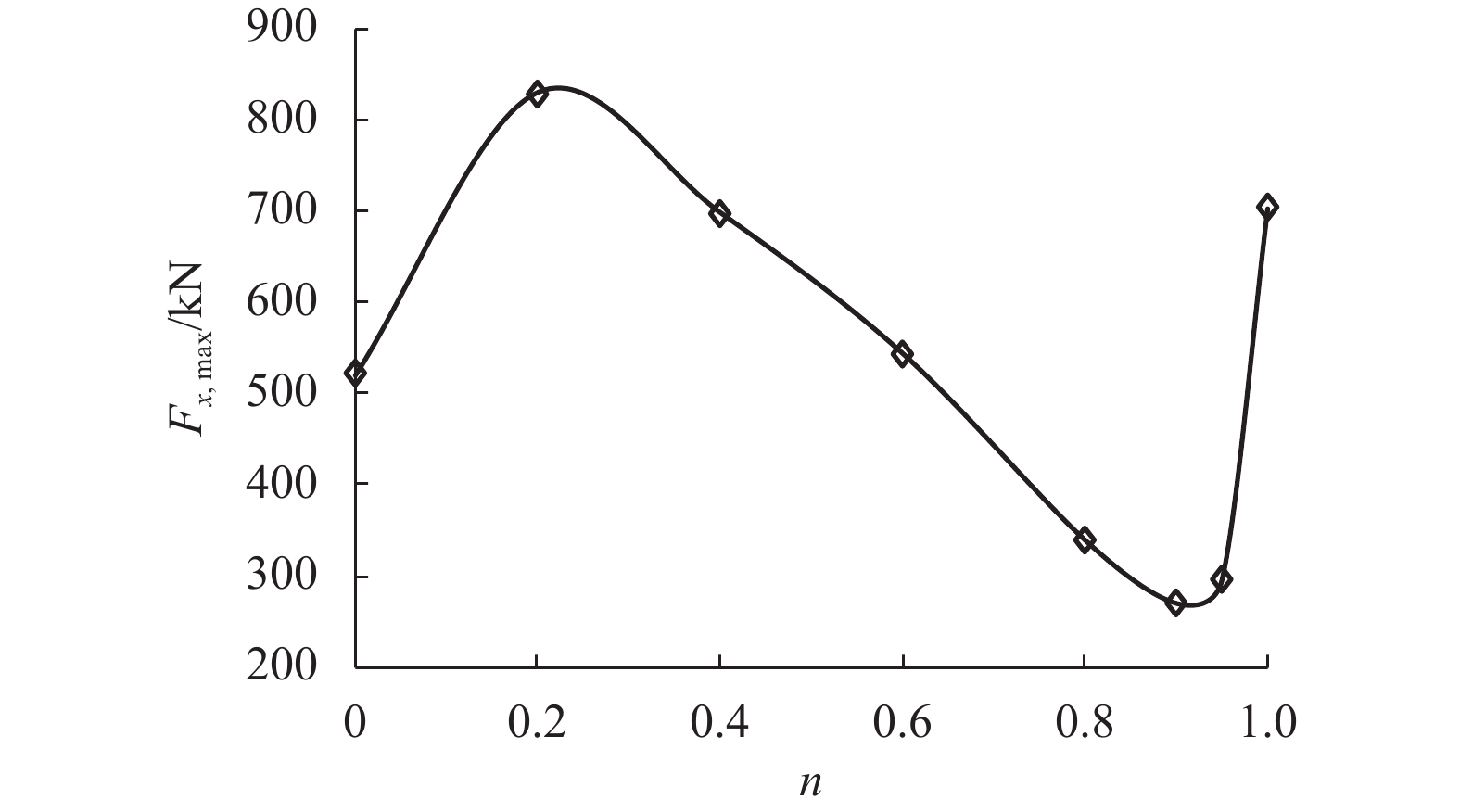
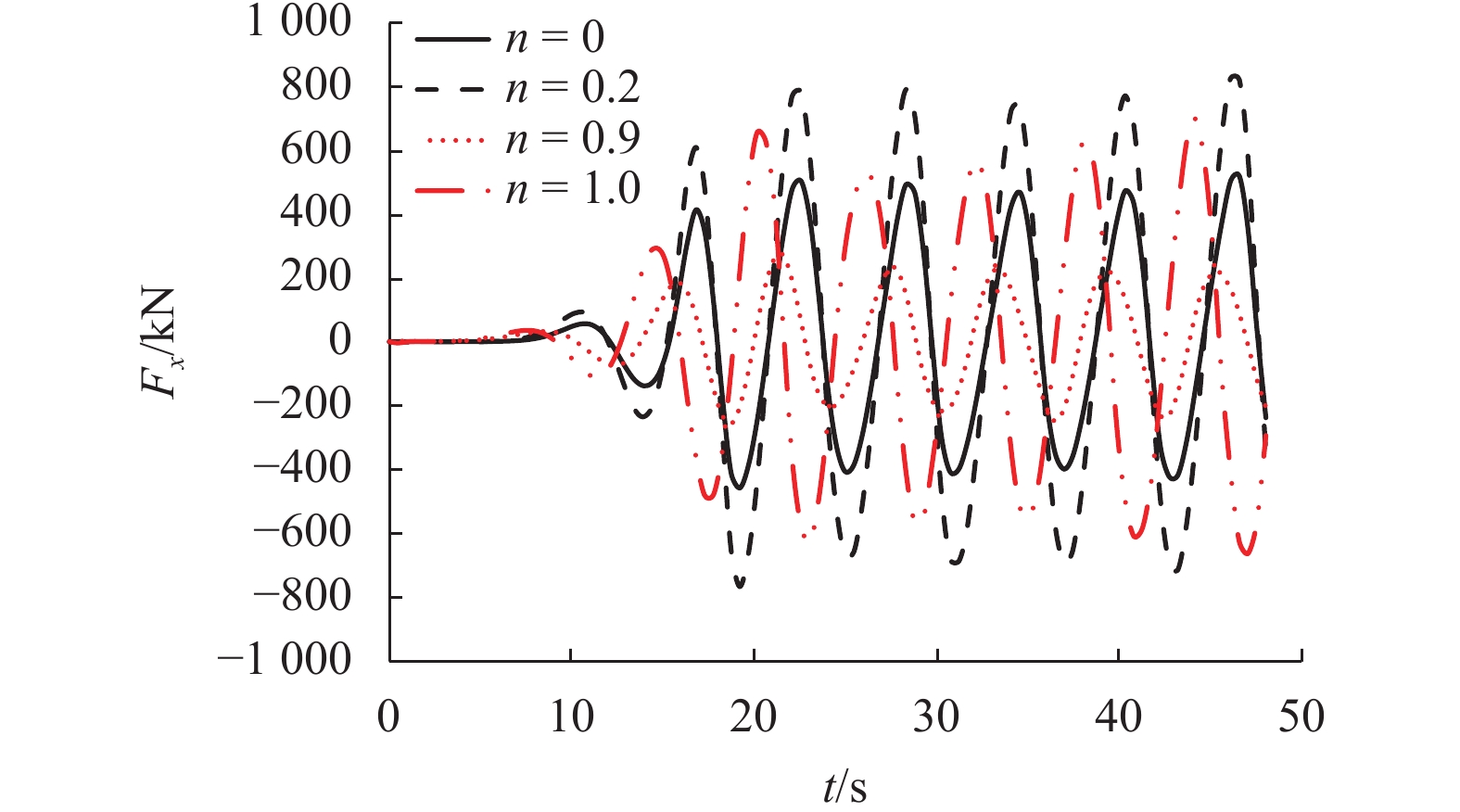
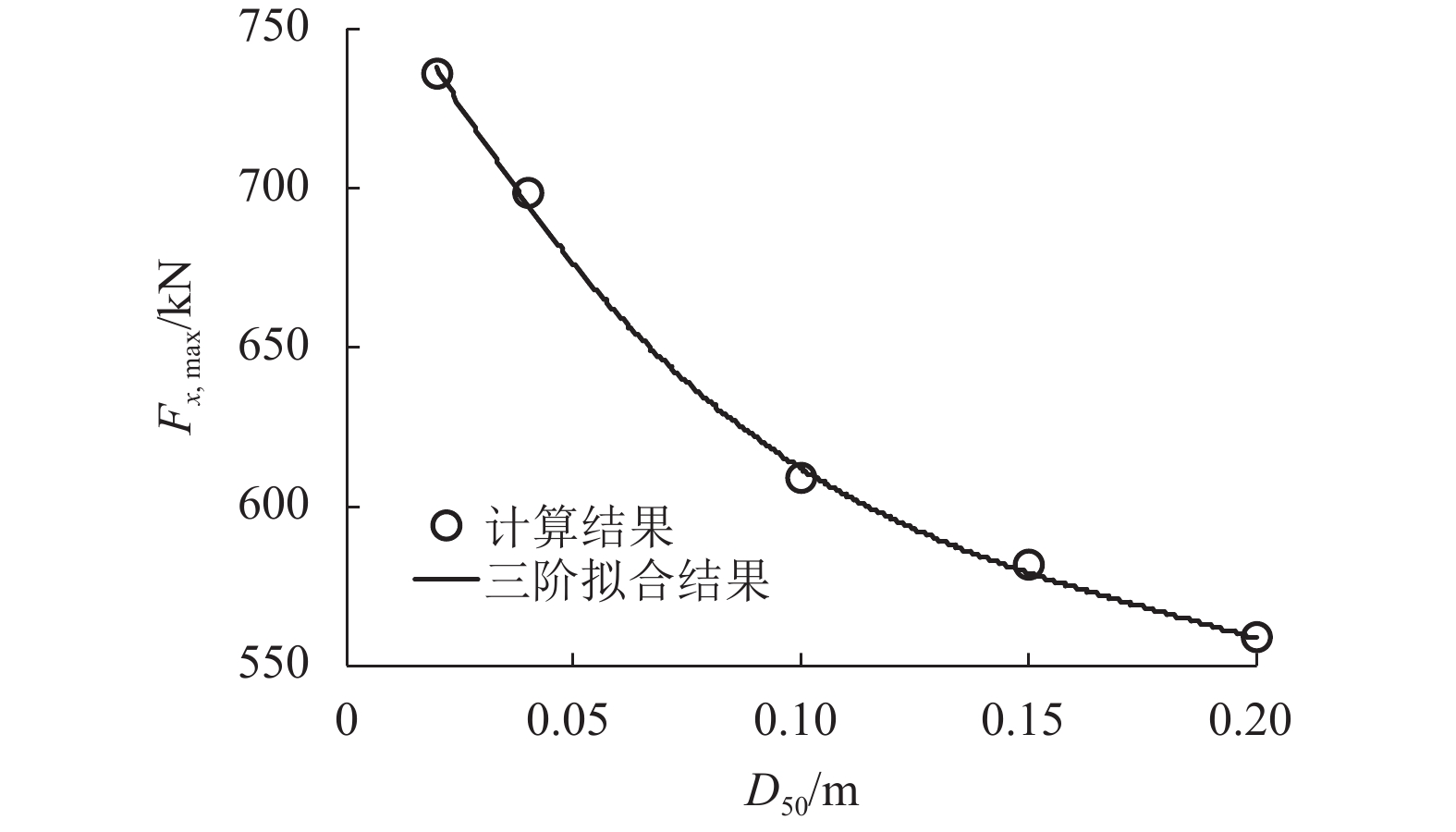
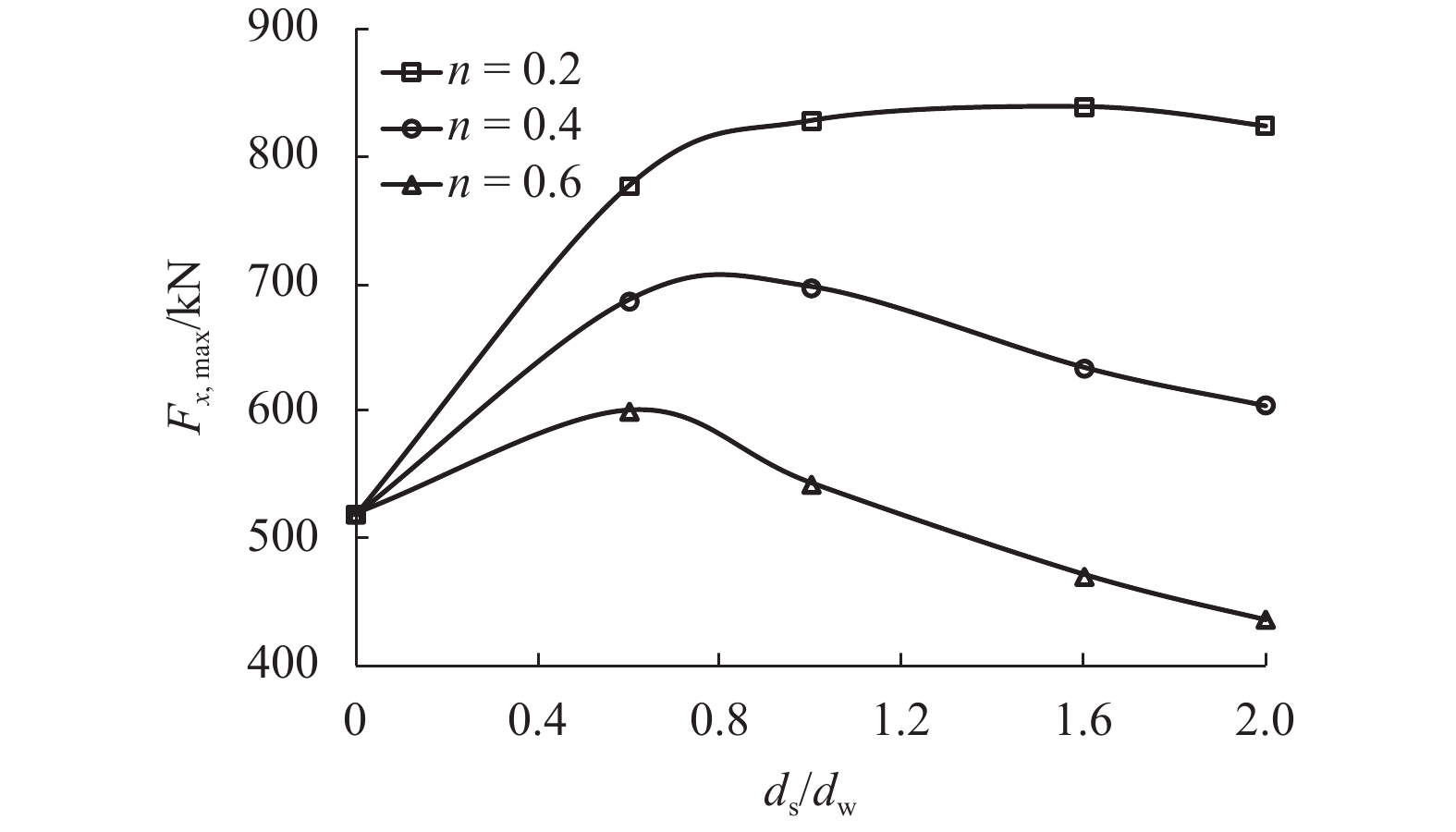
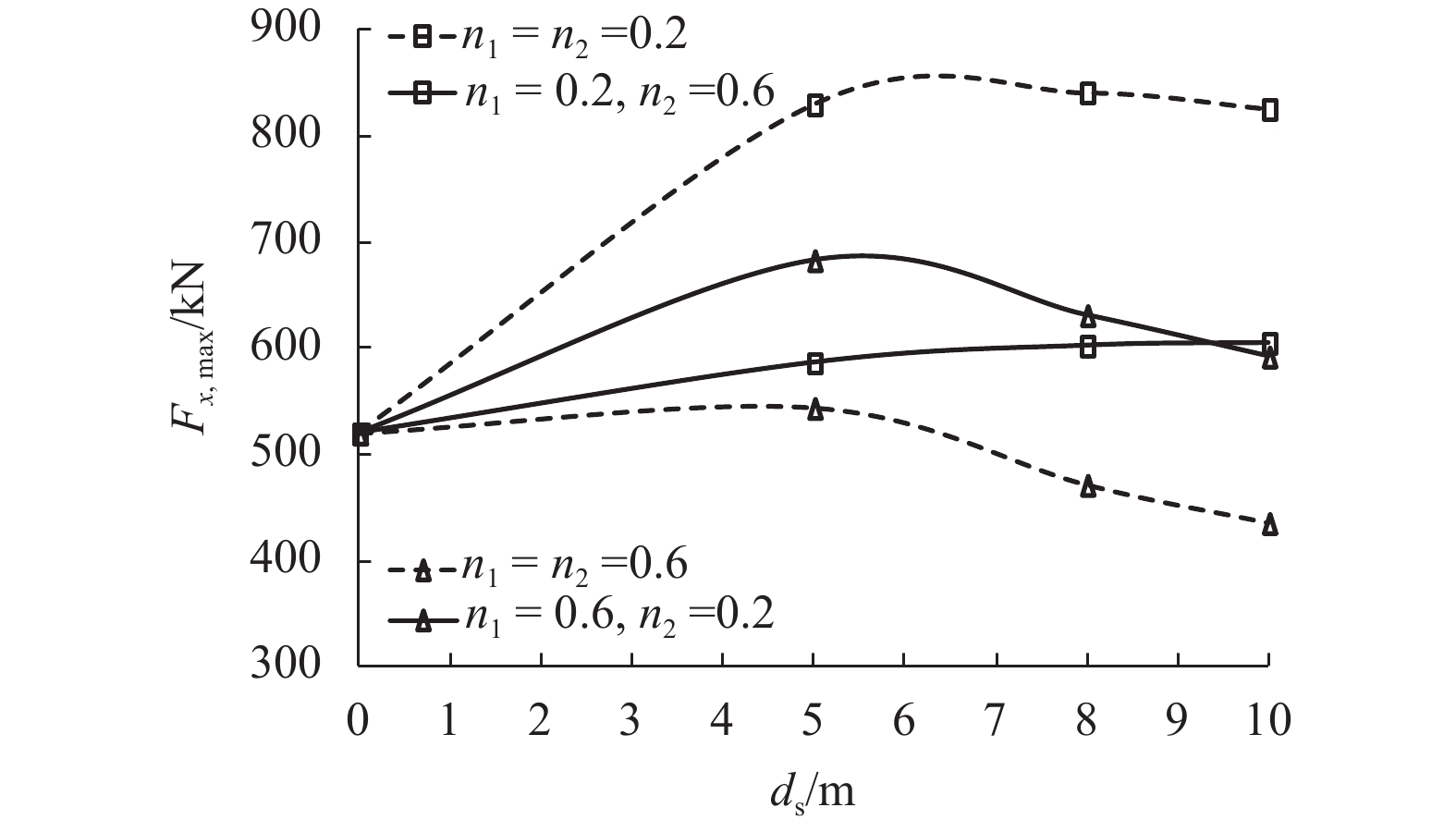
王伟, 杨敏. 海上风电机组地基基础: 设计理论与工程应用[M]. 北京: 中国建筑工业出版社, 2014: 1-20 LOSADA I J, LARA J L, JESUS M D. Modeling the interaction of water waves with porous coastal structures[J]. Journal of Waterway,Port,Coastal,and Ocean Engineering, 2016, 142(6): 1-18. PUTNAM J A. Loss of wave energy due to percolation in a permeable sea bottom[J]. Transactions,American Geophysical Union, 1949, 30(3): 349-356. doi: 10.1029/TR030i003p00349 LIU P F. Damping of water waves over porous bed[J]. Journal of the Hydraulics Division, 1973, 99: 2263-2271. SAVAGE R P, FAIRCHILD J C. Laboratory study of energy losses by bottom friction and percolation[J]. Beach Erosion Board,Corps of Engineers,Technical Memorandum, 1953, 31: 1-25. LIU P L F, DALRYMPLE R A. The damping of gravity waves due to percolation[J]. Coastal Engineering, 1984, 8(1): 33-49. doi: 10.1016/0378-3839(84)90021-8 KARUNARATHNA S, LIN P Z. Numerical simulation of wave damping over porous seabeds[J]. Coastal Engineering, 2006, 53(10): 845-855. doi: 10.1016/j.coastaleng.2006.05.003 MORISON J R, JOHNSON J W, SCHAAF S A. The force exerted by surface waves on piles[J]. Journal of Petroleum Technology, 1950, 2(5): 149-154. doi: 10.2118/950149-G MACCAMY R C, FUCHS R A. Wave forces on piles:a diffraction theory[J]. Beach Erosion Board, 1954, 69: 100-103. 李世森,张伟,秦崇仁. 大直径圆筒结构上波浪力的数值模拟与实验研究[J]. 中国港湾建设,2003(2): 11-16. doi: 10.3969/j.issn.1003-3688.2003.02.004LI Shisen, ZHANG Wei, QIN Chongren. Numerical simulation and experimental study of wave force on large diameter cylindrical structure[J]. China Harbour Engineering, 2003(2): 11-16. doi: 10.3969/j.issn.1003-3688.2003.02.004 祝兵,宋随弟,谭长建. 三维波浪作用下大直径圆柱绕流的数值模拟[J]. 西南交通大学学报,2012,47(2): 224-229.ZHU Bing, SONG Suidi, TAN Changjian. Numerical simulation for diffraction around large-diameter circular cylinder subjected to three-dimension wave[J]. Journal of Southwest Jiaotong University, 2012, 47(2): 224-229. 张文娟,王媛,倪小东. Forchheimer型非达西渗流参数特征分析[J]. 水电能源科学,2014(1): 52-54,164.ZHANG Wenjuan, WANG Yuan, NI Xiaodong. Analysis of parameters characteristics of Forchheimer’s non-Darcy seepage[J]. Water Resources and Power, 2014(1): 52-54,164. BOUSSINESQ J. Theory of wave and swells propagated in long horizontal rectangular canal and imparting to the liquid contained in this canal[J]. Journal of Mathematiques Pures et Appliquees, 1872, 17(2): 55-108. RODI W. Turbulence models and their application in hydraulics: a state of the art review[M]. 3rd edition. Rotterdam: CRC Press, 1984: 26-33 LAUNDER B E, SPALDING D B. The numerical computation of turbulence flows[J]. Computer Methods in Applied Mechanics and Engineering, 1974, 3(1): 269-289. CARMAN P C. Fluid flow through granular beds[J]. Transactions of the Institution of Chemical Engineering, 1937, 15(1): 150-167. DEAN R G. Relative validation of water wave theories[J]. Journal of the Waterways,Harbors and Coastal Engineering Division, 1970, 96(1): 105-119. FENTON J D. A fifth-order stokes theory for steady waves[J]. Journal of Waterway,Port,Coastal,and Ocean Engineering, 1985, 111(2): 216-234. ORLANSK I. A simple boundary condition for unbounded hyperbolic flows[J]. Journal of Computational Physics, 1976, 21(3): 251-269. doi: 10.1016/0021-9991(76)90023-1 HIRT C W, NICHOLS B D. Volume of fluid (VOF) method for the dynamics of free boundaries[J]. Journal of Computational Physics, 1981, 39(1): 201-225. doi: 10.1016/0021-9991(81)90145-5 -
下载:
点击查看大图
图(13)
计量
- 文章访问数: 461
- HTML全文浏览量: 245
- PDF下载量: 3
- 被引次数: 0