Buffeting Response and Equivalent Wind Load of Single Cantilever Corridor Bridge in Mountainous Areas
-
摘要: 为研究山区风环境下悬挑式人行桥梁抖振响应及风荷载,以某单悬臂观景廊桥为背景,通过风洞试验对结构的静力三分力系数以及不同风参数下的抖振响应进行了测量,并将结构横桥向最大等效风荷载规范计算值与试验值进行比较. 结果表明:山体地形对结构三分力系数及抖振响应影响较大,二者最大值均未出现在常规风向角;结构抖振响应随风速的增大而增大,受小幅风攻角的影响较小;横向抖振响应受一定程度紊流度变化的影响不敏感,但竖向及扭转响应整体随紊流度的增加呈明显增大趋势,在紊流度增大约40%的情况下二者均增大15%左右;竖向抖振响应随紊流积分尺度的增大(增幅约20%)而增大,增幅在9%左右,但积分尺度对横向抖振响应几乎无影响,对扭转响应的影响随风攻角的不同有较大差异,随着积分尺度的增大,3° 攻角下扭转响应增幅约为8%,0° 攻角其受积分尺度的变化影响较小;相比横桥向最大等效风荷载试验值,利用桥梁规范计算的结果偏于保守,静阵风系数的取值有待修正.Abstract: In order to study the buffeting response and the wind loading of a cantilevered pedestrian bridge in windy mountainous, a corridor bridge model was put in a wind tunnel to obtain its aerostatic force coefficients and buffeting responses. The maximum equivalent wind load in the transverse direction was calculated according to the bridges structural code and compared to the experimental results. The complex mountainous terrain has a significant influence on the structural aerostatic force coefficients and the buffeting responses, although neither of their maximums arises due to the general wind yaw. The structural buffeting responses increase with higher wind speeds, while are affected little by the wind attack angles in a small range. The lateral buffeting response is neither sensitive to turbulence intensity nor the turbulence integral scale. The vertical and torsional responses show an uptrend with increasing turbulence intensity, and both increase by around 15% when the turbulence intensity increases by around 40%. The vertical responses increase by about 9% if the turbulence integral scale increases by about 20%, while the lateral responses are affected little by a change in integral scale. In spite of the small influence of the turbulence integral scales on the lateral responses, the torsional responses vary greatly at different wind attack angles. At an attack angle of 3°, the torsional responses increase by around 8% with the increased integral scale, but they are influenced little by the change in integral scale at a 0° attack angle. Compared with the lateral wind loading found in the wind tunnel, the maximum equivalent calculated from the bridges structural code seems too conservative, and hence the code’s static gust factor should be further investigated.
-
表 1 各类风场特性参数
Table 1. Characteristic parameters of wind fields
风场类别 Um/(m•s–1) Uf/(m•s–1) Iu Iw Lu/m Lw/m 风场I 6.87 48.58 0.117 7 0.098 4 1.023 2 0.431 3 4.65 32.88 0.108 4 0.098 5 0.850 3 0.367 8 风场II 6.87 48.58 0.069 8 0.052 7 1.082 8 0.452 4 4.68 33.09 0.068 9 0.051 1 0.939 4 0.417 4 风场III 6.85 48.44 0.065 0 0.049 9 1.300 2 0.556 5 4.68 33.09 0.063 1 0.050 1 1.096 0 0.448 8 表 2 紊流度的变化对结构抖振响应的影响
Table 2. Effects of variation of turbulence intensity on the structural buffeting responses
结构抖振响应 I对结构抖振响应的影响 σx 随着 I的增大 ΔσIx较小,大体均在5%以内,甚至有些工况响应减小. I对 σx影响较小 σy 随着 I的增大各风向角响应值均明显增大, + 3° 攻角下整体 ΔσIy最大,各风向角响应 ΔσIy平均达到24.1%左右,0° 攻角 ΔσIy平均约为13.7%,–3° 攻角约为13.6% σθ 随着 I的增大各风向角响应值均明显增大,–3° 攻角 ΔσIθ平均约为14.9%,0° 攻角约为14.4%, + 3° 攻角约为17.1% 表 3 紊流积分尺度的变化对结构抖振响应的影响
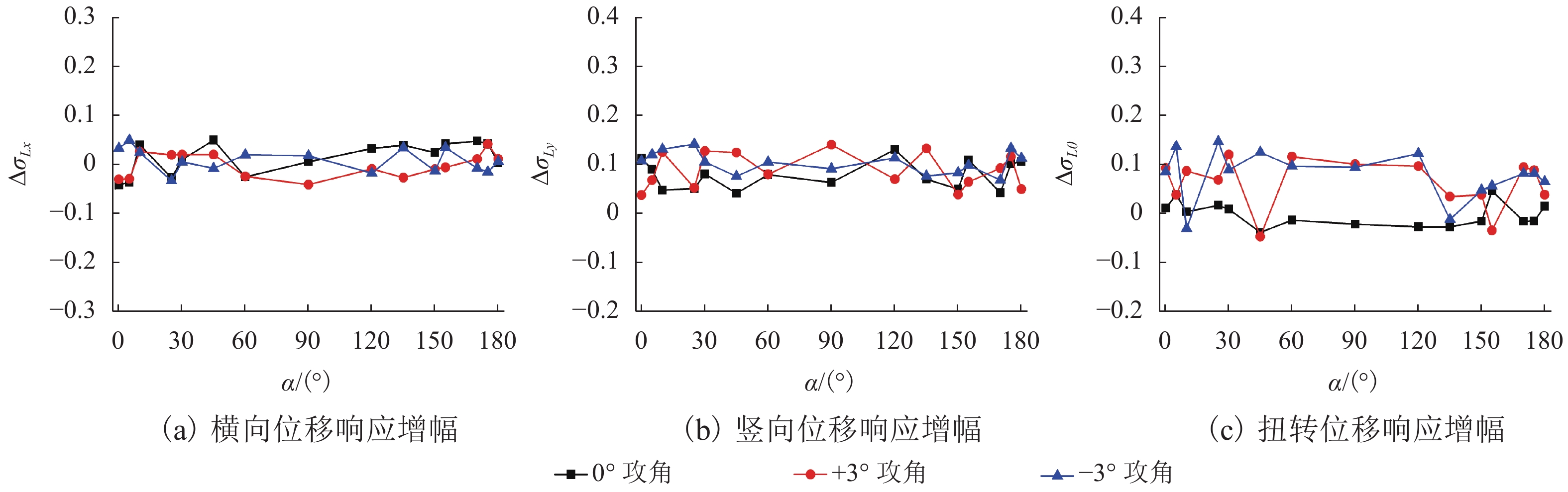
Table 3. Effects of variation of turbulence integral scale on the structural buffeting responses
结构抖振响应 L对结构抖振响应的影响 σx 所有工况各 ΔσLx不超过 ±5%, L的变化几乎无影响 σy 随着 L的增大各风向角响应值均呈现增大趋势,–3° 攻角各风向角 ΔσLy平均约为10.4%,0° 攻角 ΔσLy平均约为7.8%, + 3° 攻角 ΔσLy平均约为8.8% σθ ± 3° 攻角下,随着 L的增大各风向角响应值大体均增大,个别风向角出现减小的情况且减幅较小,除去个别响应值随L减小的工况, + 3° 和 – 3° 攻角下各风向角响应值 ΔσLθ平均分别约为9.4%、7.8%. 在0°攻角下随着 L的增大响应没有统一的变化规律,增量有正有负,且 ΔLθ较小, L影响较小 表 4 等效风荷载计算结果
Table 4. Results of equivalent wind loading
计算方法 静阵风系数 等效风荷载/N 计算误差值 《桥规》 1.325 24 361.55 38.5% 风洞试验 17 582.98 -
XIE J, HUNTER M, IRWIN P. Experimental and analytical approaches in wind engineering studies for bridges[R]. Budapest: Responding to Tomorrow’s Challenges in Structural Engineering, 2006 项海帆. 进入21世纪的桥梁风工程研究[J]. 同济大学学报 (自然科学版),2002,30(5): 529-532XIANG Haifan. Study on bridge wind engineering into 21st century[J]. Journal of Tongji University (Natural Science), 2002, 30(5): 529-532 叶征伟. 山区高墩大跨连续刚构桥风环境及风荷载研究[D]. 杭州: 浙江大学, 2012 KOSSMANN M, VÖGTLIN R, CORSMEIER U, et al. Aspects of the convective boundary layer structure over complex terrain[J]. Atmospheric Environment, 1998, 32(7): 1323-1348 doi: 10.1016/S1352-2310(97)00271-9 于舰涵,李明水,廖海黎. 山区地形对桥位风场影响的数值模拟[J]. 西南交通大学学报,2016,51(4): 654-662 doi: 10.3969/j.issn.0258-2724.2016.04.008YU Jianhan, LI Mingshui, LIAO Haili. Numerical simulation of effect of mountainous topography on wind field at bridge site[J]. Journal of Southwest Jiaotong University, 2016, 51(4): 654-662 doi: 10.3969/j.issn.0258-2724.2016.04.008 王凯,廖海黎,李明水,等. 山区峡谷桥梁设计基准风速的确定方法[J]. 西南交通大学学报,2013,48(1): 29-35 doi: 10.3969/j.issn.0258-2724.2013.01.005WANG Kai, LIAO Haili, LI Mingshui, et al. Determination method for basic design wind speed of mountainous-valley bridge[J]. Journal of Southwest Jiaotong University, 2013, 48(1): 29-35 doi: 10.3969/j.issn.0258-2724.2013.01.005 徐洪涛. 山区峡谷风特性参数及大跨度桁梁桥风致振动研究[D]. 成都: 西南交通大学, 2009 U.S. Department of Commerce, National Bureau of Standards. Turbulent wind effects on tension leg platform surge[S]. Washington D C: Government Printing Office, 1983 中交公路规划设计院. 公路桥梁抗风设计规范: JTG/TD60-01—2004[S]. 北京: 人民交通出版社, 2004 KIMURA K, TANAKA H. Bridge buffeting due to wind with yaw angles[J]. Journal of Wind Engineering & Industrial Aerodynamics, 1992, 42(1/2/3): 1309-1320 ZHU L D, XU Y L. Buffeting response of long-span cable-supported bridges under skew winds, part 1:theory[J]. Journal of Sound & Vibration, 2005, 281(3/4/5): 647-673 BARNARD R H. Wind loads on cantilevered roof structures[J]. Journal of Wind Engineering & Industrial Aerodynamics, 1981, 8(1): 21-30 王浩,李爱群,焦常科,等. 桥塔风效应对大跨度悬索桥抖振响应的影响[J]. 振动与冲击,2010,29(8): 103-106 doi: 10.3969/j.issn.1000-3835.2010.08.023WANG Hao, LI Aiqun, JIAO Changke, et al. Bridge tower wind effects on buffeting response of long-span suspension bridges[J]. Journal of Vibration and Shock, 2010, 29(8): 103-106 doi: 10.3969/j.issn.1000-3835.2010.08.023 庞加斌,葛耀君,陆烨. 大气边界层湍流积分尺度的分析方法[J]. 同济大学学报 (自然科学版),2002,30(5): 529-532PANG Jiabin, GE Yaojun, LU Ye. Methods for analysis of turbulence integral length in atmospheric boundary-layer[J]. Journal of Tongji University (Natural science), 2002, 30(5): 529-532 公路桥梁抗风设计指南编写组. 公路桥梁抗风设计指南[M]. 北京: 人民交通出版社, 1996: 24 期刊类型引用(12)
1. 胡朋,陈婉婷,韩艳,陈飞,丁少凌. 基于大涡模拟的山区桥址风场及其对桥梁抖振响应的影响. 湖南大学学报(自然科学版). 2025(01): 160-175 .  百度学术
百度学术2. 张捷,刘小波,杨霄,张天翼. 山区峡谷大跨度斜拉桥施工阶段抖振响应及抗风索减振措施研究. 世界桥梁. 2025(01): 79-87 .  百度学术
百度学术3. 敬大德,苏益. 桥梁气动外形改变对大跨桁梁桥抗风性能影响研究. 中外公路. 2024(01): 119-125 .  百度学术
百度学术4. 李航,欧阳璐,范周健,刘启明,王海,孙秀贵. 异形人行景观斜拉桥全桥气弹模型风洞试验研究. 市政技术. 2023(09): 185-191 .  百度学术
百度学术5. 李永志,董世杰,王佳豪,吴川逸晖,吴潮彬,殷东朔. 廊架对人行刚构桥涡振性能的影响. 河海大学学报(自然科学版). 2023(05): 87-96 .  百度学术
百度学术6. 徐梓栋,王浩,赵恺雍,郎天翼,温学华. 台风环境大跨度廊桥非平稳抖振分析及舒适度评估. 东南大学学报(自然科学版). 2023(06): 1028-1033 .  百度学术
百度学术7. 王可峰,陈涛,苏益. 某山区人行廊桥三分力系数风洞实验研究. 城市道桥与防洪. 2022(02): 179-182+185+22-23 .  百度学术
百度学术8. 王良. 廊桥风雨亭气动参数及风振系数分析. 城市道桥与防洪. 2021(10): 106-109+16 .  百度学术
百度学术9. 赵军杰,牛华伟,陈政清,周子祺,郑甲强,黄文理. 开槽变截面人行桥的气动性能与TMD减振研究. 铁道科学与工程学报. 2020(09): 2296-2302 .  百度学术
百度学术10. 范大蔚,佟佳慧. 一种基于环境预报数据的阵风建模方法. 系统仿真学报. 2020(12): 2409-2414 .  百度学术
百度学术11. 肖春生,范大蔚,林建军. 大气紊流对飞行器飞行和命中率影响研究. 战术导弹技术. 2020(06): 127-131 .  百度学术
百度学术12. 苏益,李明水. 大跨度桥梁抖振响应的直接估算方法. 中国公路学报. 2019(10): 84-95 .  百度学术
百度学术其他类型引用(9)
-





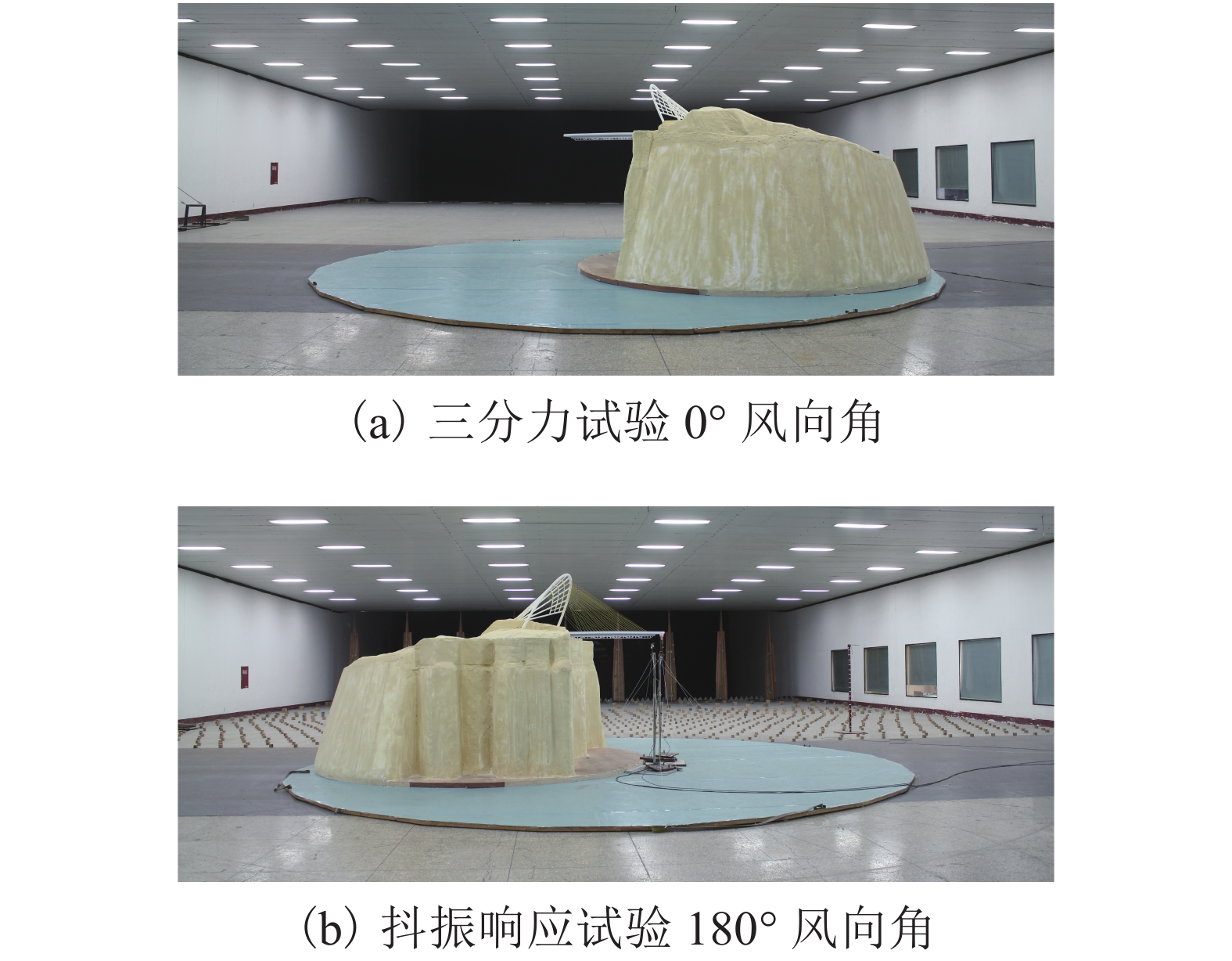
 下载:
下载:
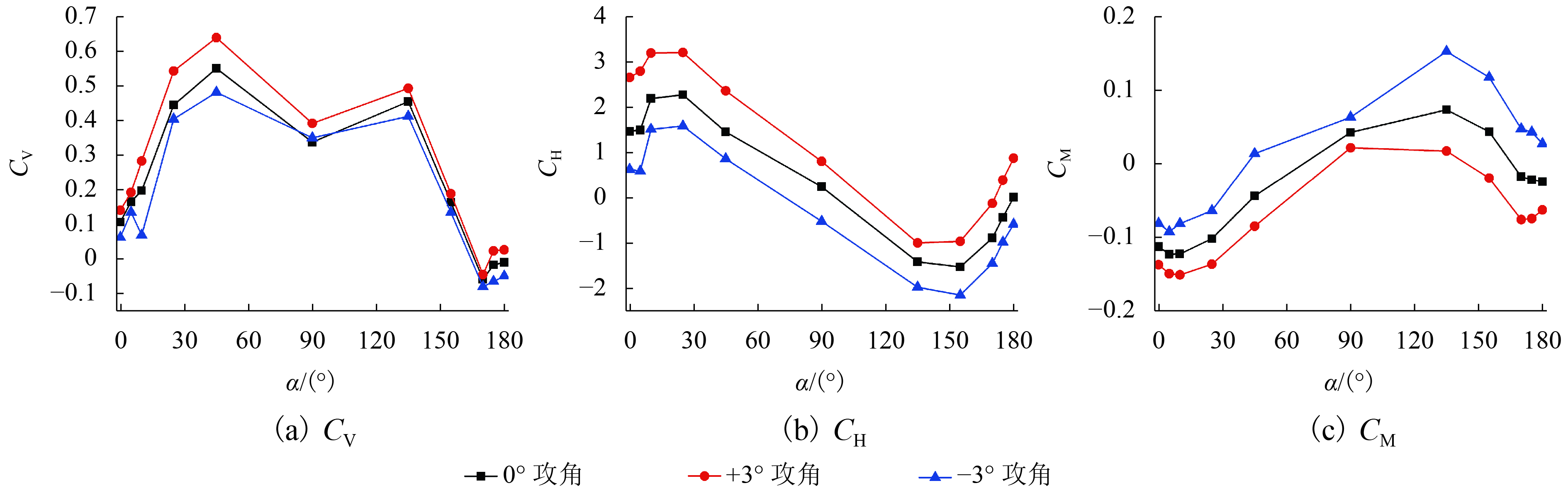

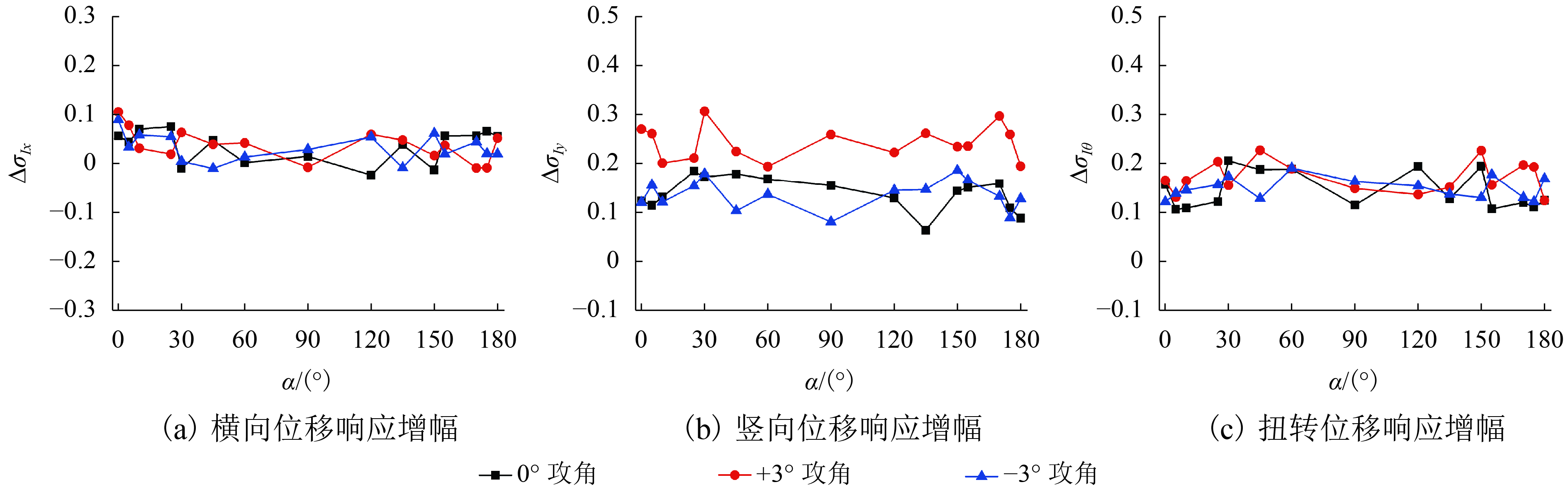
 百度学术
百度学术
